Question Number 165505 by mathlove last updated on 02/Feb/22
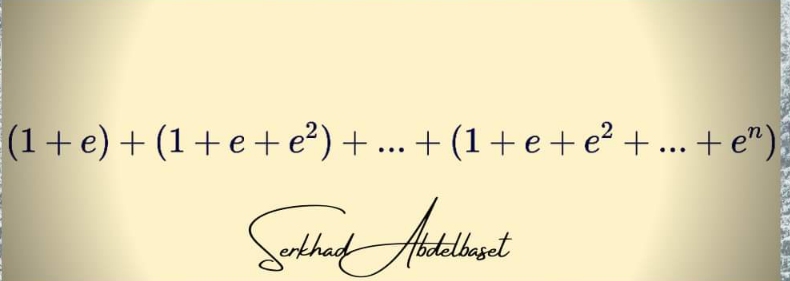
Answered by TheSupreme last updated on 02/Feb/22
![Σ_(i=0) ^n (n−i)e^i =n((1−e^(n+1) )/(1−e))−Σie^i = =n((1−e^(n+1) )/(1−e))−(1/e)ΣD(e^i )= =n((1−e^(n+1) )/(1−e))−(1/e)D(((1−e^(n+1) )/(1−e)))= =((n(1−e^(n+1) ))/(1−e))−(1/e)[((−(n+1)(1−e)+(1−e^(n+1) ))/((1−e)^2 ))]= =((ne(1−e^(n+1) )(1−e)−(1−e^(n+1) )+(1−e)(n+1))/(e(1−e)^2 ))= =((ne(1−e^(n+1) −e+e^(n+2) )−1+e^(n+1) +n+1−ne−1)/(e(1−e)^2 ))= =((−ne^(n+2) −ne^2 +ne^(n+3) +e^(n+1) +n−1)/(e(1−e)^2 ))](https://www.tinkutara.com/question/Q165507.png)
$$\underset{{i}=\mathrm{0}} {\overset{{n}} {\sum}}\left({n}−{i}\right){e}^{{i}} ={n}\frac{\mathrm{1}−{e}^{{n}+\mathrm{1}} }{\mathrm{1}−{e}}−\Sigma{ie}^{{i}} = \\ $$$$={n}\frac{\mathrm{1}−{e}^{{n}+\mathrm{1}} }{\mathrm{1}−{e}}−\frac{\mathrm{1}}{{e}}\Sigma{D}\left({e}^{{i}} \right)= \\ $$$$={n}\frac{\mathrm{1}−{e}^{{n}+\mathrm{1}} }{\mathrm{1}−{e}}−\frac{\mathrm{1}}{{e}}{D}\left(\frac{\mathrm{1}−{e}^{{n}+\mathrm{1}} }{\mathrm{1}−{e}}\right)= \\ $$$$=\frac{{n}\left(\mathrm{1}−{e}^{{n}+\mathrm{1}} \right)}{\mathrm{1}−{e}}−\frac{\mathrm{1}}{{e}}\left[\frac{−\left({n}+\mathrm{1}\right)\left(\mathrm{1}−{e}\right)+\left(\mathrm{1}−{e}^{{n}+\mathrm{1}} \right)}{\left(\mathrm{1}−{e}\right)^{\mathrm{2}} }\right]= \\ $$$$=\frac{{ne}\left(\mathrm{1}−{e}^{{n}+\mathrm{1}} \right)\left(\mathrm{1}−{e}\right)−\left(\mathrm{1}−{e}^{{n}+\mathrm{1}} \right)+\left(\mathrm{1}−{e}\right)\left({n}+\mathrm{1}\right)}{{e}\left(\mathrm{1}−{e}\right)^{\mathrm{2}} }= \\ $$$$=\frac{{ne}\left(\mathrm{1}−{e}^{{n}+\mathrm{1}} −{e}+{e}^{{n}+\mathrm{2}} \right)−\mathrm{1}+{e}^{{n}+\mathrm{1}} +{n}+\mathrm{1}−{ne}−\mathrm{1}}{{e}\left(\mathrm{1}−{e}\right)^{\mathrm{2}} }= \\ $$$$=\frac{−{ne}^{{n}+\mathrm{2}} −{ne}^{\mathrm{2}} +{ne}^{{n}+\mathrm{3}} +{e}^{{n}+\mathrm{1}} +{n}−\mathrm{1}}{{e}\left(\mathrm{1}−{e}\right)^{\mathrm{2}} } \\ $$
