Menu Close

Question-166208

Tinku Tara 0 Comments
Pin




Question Number 166208 by ajfour last updated on 15/Feb/22
Commented by ajfour last updated on 15/Feb/22
If both circles have equal radii, find it.
$${If}\:{both}\:{circles}\:{have}\:{equal}\:{radii}, \\ $$$${find}\:{it}. \\ $$
Answered by Eulerian last updated on 15/Feb/22
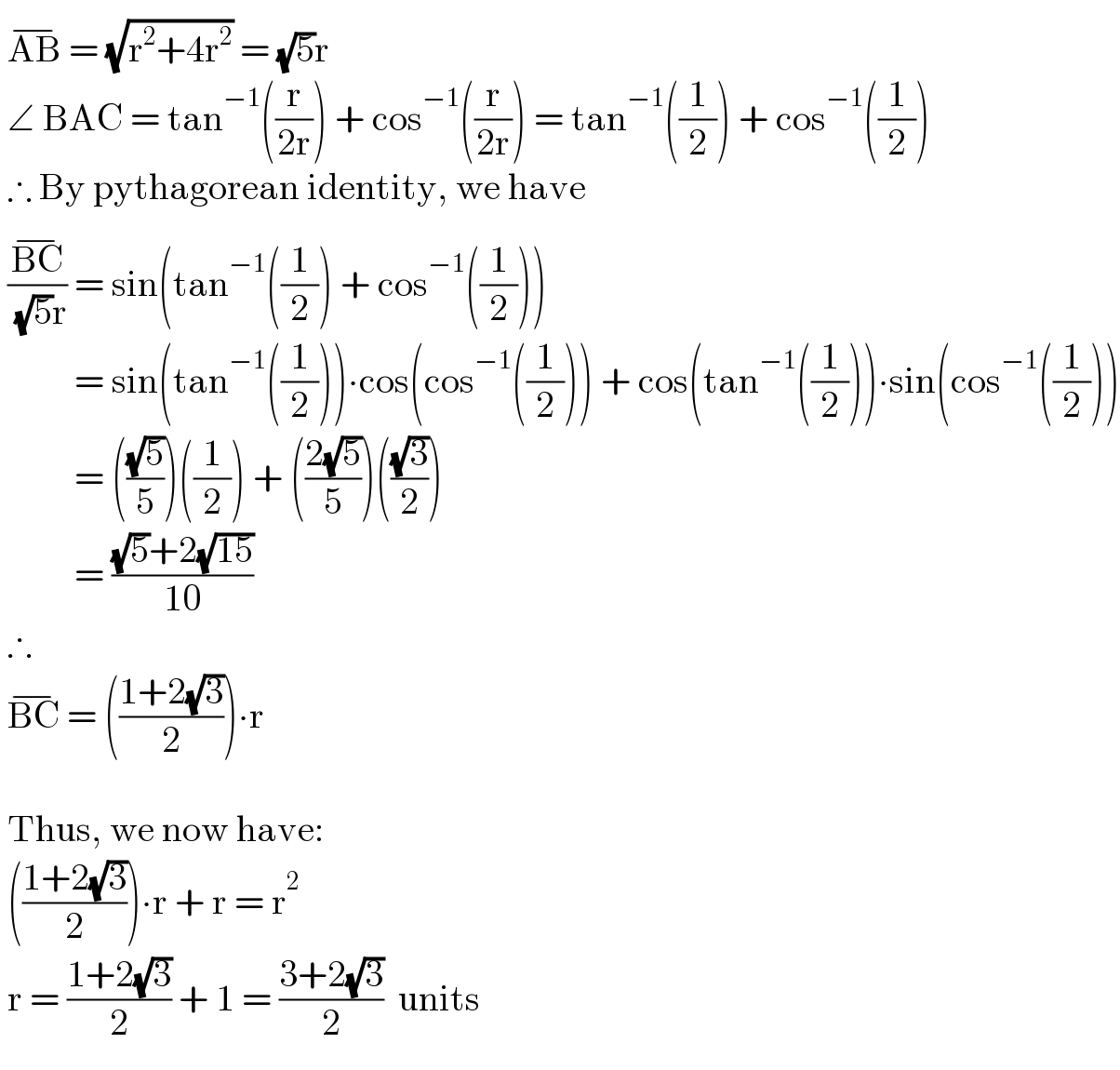
AB^(−) = (√(r^2 +4r^2 )) = (√5)r ∠ BAC = tan^(−1) ((r/(2r))) + cos^(−1) ((r/(2r))) = tan^(−1) ((1/2)) + cos^(−1) ((1/2)) ∴ By pythagorean identity, we have ((BC^(−) )/( (√5)r)) = sin(tan^(−1) ((1/2)) + cos^(−1) ((1/2))) = sin(tan^(−1) ((1/2)))∙cos(cos^(−1) ((1/2))) + cos(tan^(−1) ((1/2)))∙sin(cos^(−1) ((1/2))) = (((√5)/5))((1/2)) + (((2(√5))/5))(((√3)/2)) = (((√5)+2(√(15)))/(10)) ∴ BC^(−) = (((1+2(√3))/2))∙r Thus, we now have: (((1+2(√3))/2))∙r + r = r^2 r = ((1+2(√3))/2) + 1 = ((3+2(√3))/2) units
$$\:\overline {\mathrm{AB}}\:=\:\sqrt{\mathrm{r}^{\mathrm{2}} +\mathrm{4r}^{\mathrm{2}} }\:=\:\sqrt{\mathrm{5}}\mathrm{r} \\ $$$$\:\angle\:\mathrm{BAC}\:=\:\mathrm{tan}^{−\mathrm{1}} \left(\frac{\mathrm{r}}{\mathrm{2r}}\right)\:+\:\mathrm{cos}^{−\mathrm{1}} \left(\frac{\mathrm{r}}{\mathrm{2r}}\right)\:=\:\mathrm{tan}^{−\mathrm{1}} \left(\frac{\mathrm{1}}{\mathrm{2}}\right)\:+\:\mathrm{cos}^{−\mathrm{1}} \left(\frac{\mathrm{1}}{\mathrm{2}}\right) \\ $$$$\:\therefore\:\mathrm{By}\:\mathrm{pythagorean}\:\mathrm{identity},\:\mathrm{we}\:\mathrm{have} \\ $$$$\:\frac{\overline {\mathrm{BC}}}{\:\sqrt{\mathrm{5}}\mathrm{r}}\:=\:\mathrm{sin}\left(\mathrm{tan}^{−\mathrm{1}} \left(\frac{\mathrm{1}}{\mathrm{2}}\right)\:+\:\mathrm{cos}^{−\mathrm{1}} \left(\frac{\mathrm{1}}{\mathrm{2}}\right)\right)\: \\ $$$$\:\:\:\:\:\:\:\:\:\:=\:\mathrm{sin}\left(\mathrm{tan}^{−\mathrm{1}} \left(\frac{\mathrm{1}}{\mathrm{2}}\right)\right)\centerdot\mathrm{cos}\left(\mathrm{cos}^{−\mathrm{1}} \left(\frac{\mathrm{1}}{\mathrm{2}}\right)\right)\:+\:\mathrm{cos}\left(\mathrm{tan}^{−\mathrm{1}} \left(\frac{\mathrm{1}}{\mathrm{2}}\right)\right)\centerdot\mathrm{sin}\left(\mathrm{cos}^{−\mathrm{1}} \left(\frac{\mathrm{1}}{\mathrm{2}}\right)\right) \\ $$$$\:\:\:\:\:\:\:\:\:\:=\:\left(\frac{\sqrt{\mathrm{5}}}{\mathrm{5}}\right)\left(\frac{\mathrm{1}}{\mathrm{2}}\right)\:+\:\left(\frac{\mathrm{2}\sqrt{\mathrm{5}}}{\mathrm{5}}\right)\left(\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\right) \\ $$$$\:\:\:\:\:\:\:\:\:\:=\:\frac{\sqrt{\mathrm{5}}+\mathrm{2}\sqrt{\mathrm{15}}}{\mathrm{10}} \\ $$$$\:\therefore \\ $$$$\:\overline {\mathrm{BC}}\:=\:\left(\frac{\mathrm{1}+\mathrm{2}\sqrt{\mathrm{3}}}{\mathrm{2}}\right)\centerdot\mathrm{r} \\ $$$$\: \\ $$$$\:\mathrm{Thus},\:\mathrm{we}\:\mathrm{now}\:\mathrm{have}: \\ $$$$\:\left(\frac{\mathrm{1}+\mathrm{2}\sqrt{\mathrm{3}}}{\mathrm{2}}\right)\centerdot\mathrm{r}\:+\:\mathrm{r}\:=\:\mathrm{r}^{\mathrm{2}} \\ $$$$\:\mathrm{r}\:=\:\frac{\mathrm{1}+\mathrm{2}\sqrt{\mathrm{3}}}{\mathrm{2}}\:+\:\mathrm{1}\:=\:\frac{\mathrm{3}+\mathrm{2}\sqrt{\mathrm{3}}}{\mathrm{2}}\:\:\mathrm{units} \\ $$
Commented by ajfour last updated on 15/Feb/22
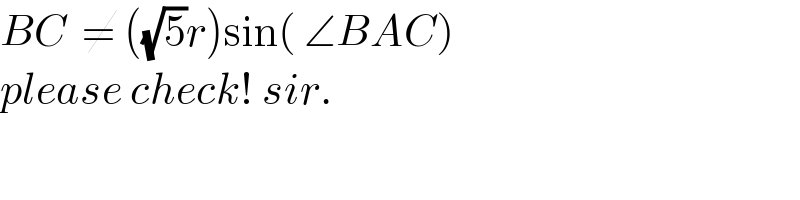
BC ≠ ((√5)r)sin( ∠BAC) please check! sir.
$${BC}\:\:\neq\:\left(\sqrt{\mathrm{5}}{r}\right)\mathrm{sin}\left(\:\angle{BAC}\right) \\ $$$${please}\:{check}!\:{sir}. \\ $$
Answered by mr W last updated on 15/Feb/22
Commented by Tawa11 last updated on 16/Feb/22
Great sir.
$$\mathrm{Great}\:\mathrm{sir}. \\ $$
Commented by mr W last updated on 16/Feb/22
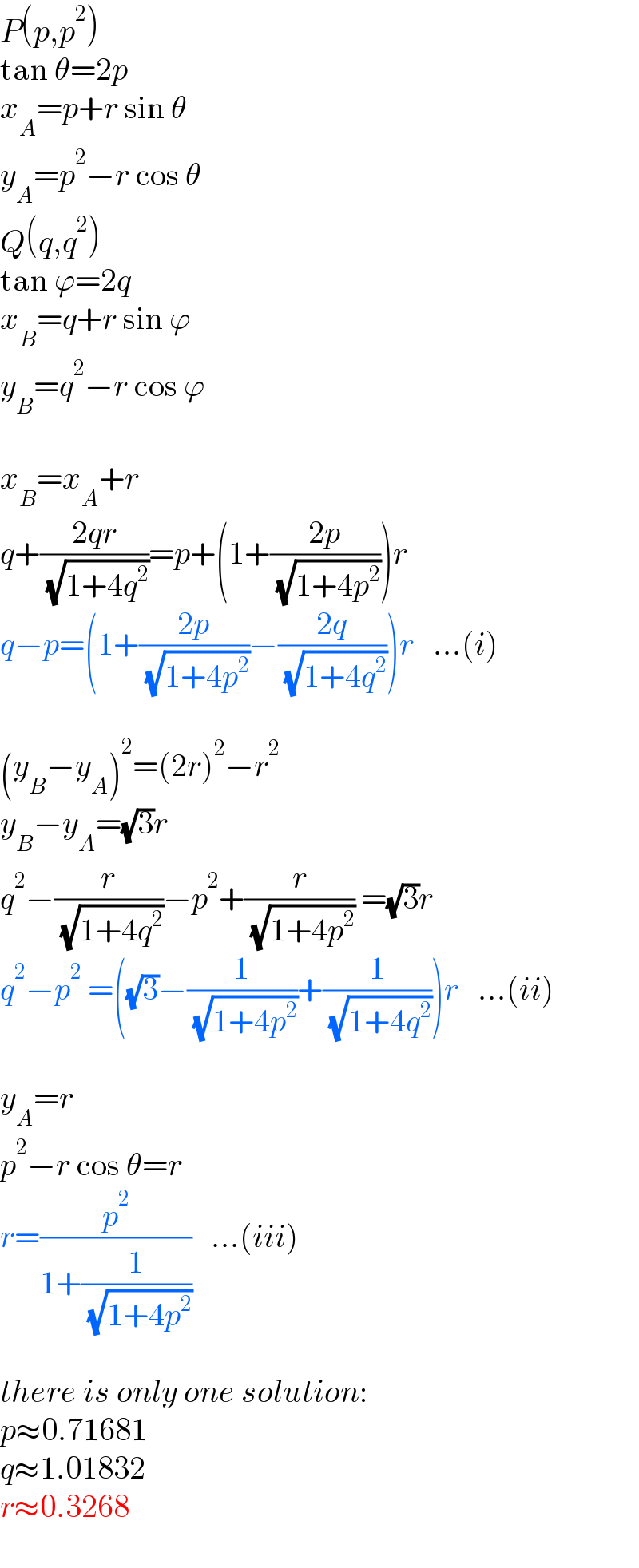
P(p,p^2 ) tan θ=2p x_A =p+r sin θ y_A =p^2 −r cos θ Q(q,q^2 ) tan ϕ=2q x_B =q+r sin ϕ y_B =q^2 −r cos ϕ x_B =x_A +r q+((2qr)/( (√(1+4q^2 ))))=p+(1+((2p)/( (√(1+4p^2 )))))r q−p=(1+((2p)/( (√(1+4p^2 ))))−((2q)/( (√(1+4q^2 )))))r ...(i) (y_B −y_A )^2 =(2r)^2 −r^2 y_B −y_A =(√3)r q^2 −(r/( (√(1+4q^2 ))))−p^2 +(r/( (√(1+4p^2 )))) =(√3)r q^2 −p^2 =((√3)−(1/( (√(1+4p^2 ))))+(1/( (√(1+4q^2 )))))r ...(ii) y_A =r p^2 −r cos θ=r r=(p^2 /(1+(1/( (√(1+4p^2 )))))) ...(iii) there is only one solution: p≈0.71681 q≈1.01832 r≈0.3268
$${P}\left({p},{p}^{\mathrm{2}} \right) \\ $$$$\mathrm{tan}\:\theta=\mathrm{2}{p} \\ $$$${x}_{{A}} ={p}+{r}\:\mathrm{sin}\:\theta \\ $$$${y}_{{A}} ={p}^{\mathrm{2}} −{r}\:\mathrm{cos}\:\theta \\ $$$${Q}\left({q},{q}^{\mathrm{2}} \right) \\ $$$$\mathrm{tan}\:\varphi=\mathrm{2}{q} \\ $$$${x}_{{B}} ={q}+{r}\:\mathrm{sin}\:\varphi \\ $$$${y}_{{B}} ={q}^{\mathrm{2}} −{r}\:\mathrm{cos}\:\varphi \\ $$$$ \\ $$$${x}_{{B}} ={x}_{{A}} +{r} \\ $$$${q}+\frac{\mathrm{2}{qr}}{\:\sqrt{\mathrm{1}+\mathrm{4}{q}^{\mathrm{2}} }}={p}+\left(\mathrm{1}+\frac{\mathrm{2}{p}}{\:\sqrt{\mathrm{1}+\mathrm{4}{p}^{\mathrm{2}} }}\right){r} \\ $$$${q}−{p}=\left(\mathrm{1}+\frac{\mathrm{2}{p}}{\:\sqrt{\mathrm{1}+\mathrm{4}{p}^{\mathrm{2}} }}−\frac{\mathrm{2}{q}}{\:\sqrt{\mathrm{1}+\mathrm{4}{q}^{\mathrm{2}} }}\right){r}\:\:\:…\left({i}\right) \\ $$$$ \\ $$$$\left({y}_{{B}} −{y}_{{A}} \right)^{\mathrm{2}} =\left(\mathrm{2}{r}\right)^{\mathrm{2}} −{r}^{\mathrm{2}} \\ $$$${y}_{{B}} −{y}_{{A}} =\sqrt{\mathrm{3}}{r} \\ $$$${q}^{\mathrm{2}} −\frac{{r}}{\:\sqrt{\mathrm{1}+\mathrm{4}{q}^{\mathrm{2}} }}−{p}^{\mathrm{2}} +\frac{{r}}{\:\sqrt{\mathrm{1}+\mathrm{4}{p}^{\mathrm{2}} }}\:=\sqrt{\mathrm{3}}{r} \\ $$$${q}^{\mathrm{2}} −{p}^{\mathrm{2}} \:=\left(\sqrt{\mathrm{3}}−\frac{\mathrm{1}}{\:\sqrt{\mathrm{1}+\mathrm{4}{p}^{\mathrm{2}} }}+\frac{\mathrm{1}}{\:\sqrt{\mathrm{1}+\mathrm{4}{q}^{\mathrm{2}} }}\right){r}\:\:\:…\left({ii}\right) \\ $$$$ \\ $$$${y}_{{A}} ={r} \\ $$$${p}^{\mathrm{2}} −{r}\:\mathrm{cos}\:\theta={r} \\ $$$${r}=\frac{{p}^{\mathrm{2}} }{\mathrm{1}+\frac{\mathrm{1}}{\:\sqrt{\mathrm{1}+\mathrm{4}{p}^{\mathrm{2}} }}}\:\:\:…\left({iii}\right) \\ $$$$ \\ $$$${there}\:{is}\:{only}\:{one}\:{solution}: \\ $$$${p}\approx\mathrm{0}.\mathrm{71681} \\ $$$${q}\approx\mathrm{1}.\mathrm{01832} \\ $$$${r}\approx\mathrm{0}.\mathrm{3268} \\ $$
Commented by mr W last updated on 16/Feb/22
Commented by mr W last updated on 16/Feb/22

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *