Question-166372

Facebook Tweet Pin

Question Number 166372 by mathlove last updated on 19/Feb/22

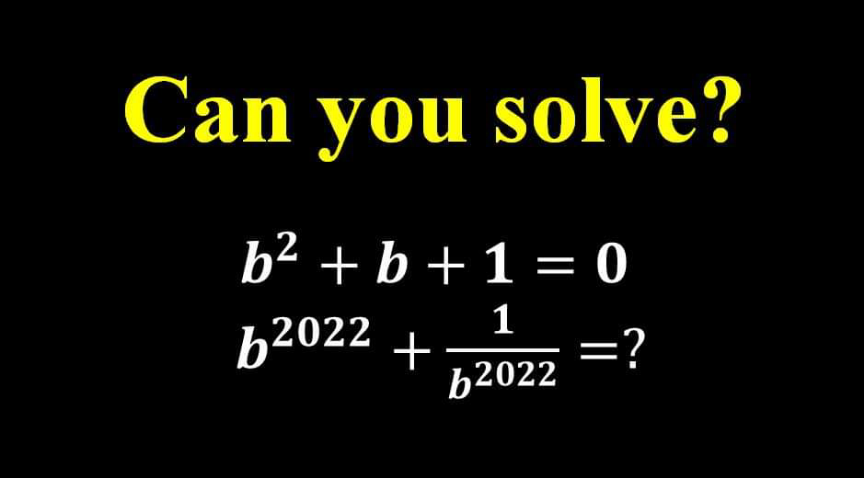
Answered by MJS_new last updated on 19/Feb/22

$$\mathrm{Yes}\:\mathrm{I}\:\mathrm{can}. \\ $$

Commented by mr W last updated on 19/Feb/22

$${yes},\:{we}\:{can}! \\ $$

Commented by peter frank last updated on 19/Feb/22

$$\mathrm{hahahhahah} \\ $$

Answered by MathsFan last updated on 19/Feb/22

$$\boldsymbol{\mathrm{yes}}\:\boldsymbol{\mathrm{i}}\:\boldsymbol{\mathrm{can}}! \\ $$

Answered by Rasheed.Sindhi last updated on 19/Feb/22

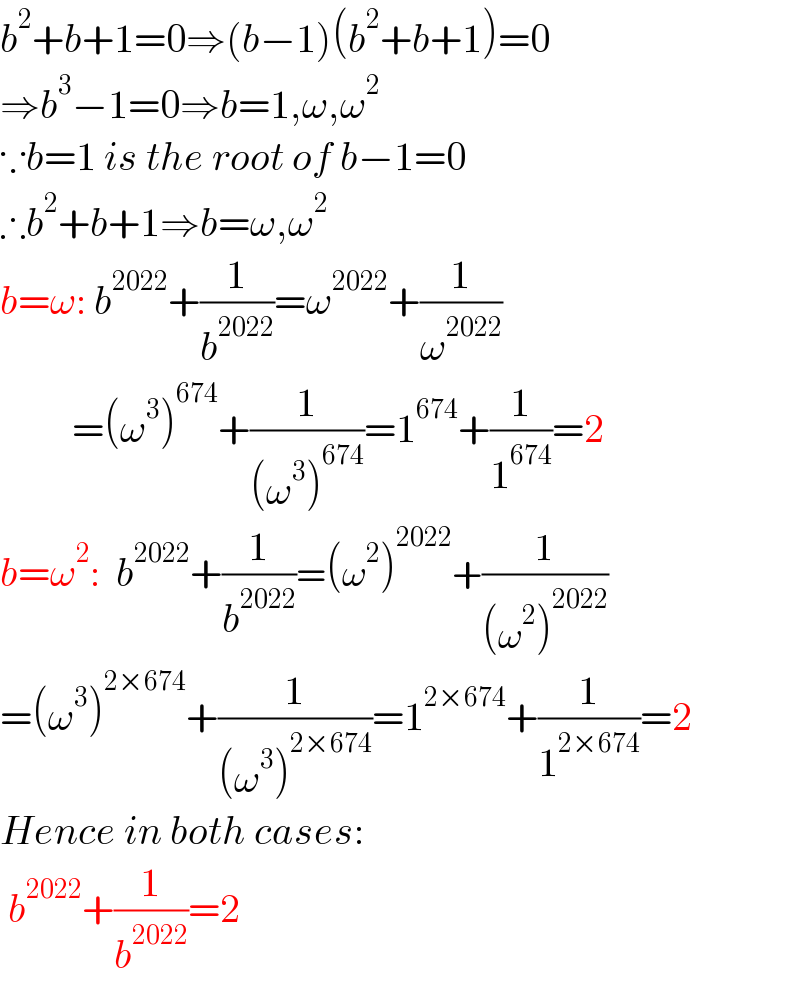
b^2 +b+1=0⇒(b−1)(b^2 +b+1)=0 ⇒b^3 −1=0⇒b=1,ω,ω^2 ∵b=1 is the root of b−1=0 ∴b^2 +b+1⇒b=ω,ω^2 b=ω: b^(2022) +(1/b^(2022) )=ω^(2022) +(1/ω^(2022) ) =(ω^3 )^(674) +(1/((ω^3 )^(674) ))=1^(674) +(1/1^(674) )=2 b=ω^2 : b^(2022) +(1/b^(2022) )=(ω^2 )^(2022) +(1/((ω^2 )^(2022) )) =(ω^3 )^(2×674) +(1/((ω^3 )^(2×674) ))=1^(2×674) +(1/1^(2×674) )=2 Hence in both cases: b^(2022) +(1/b^(2022) )=2

$${b}^{\mathrm{2}} +{b}+\mathrm{1}=\mathrm{0}\Rightarrow\left({b}−\mathrm{1}\right)\left({b}^{\mathrm{2}} +{b}+\mathrm{1}\right)=\mathrm{0} \\ $$$$\Rightarrow{b}^{\mathrm{3}} −\mathrm{1}=\mathrm{0}\Rightarrow{b}=\mathrm{1},\omega,\omega^{\mathrm{2}} \\ $$$$\because{b}=\mathrm{1}\:{is}\:{the}\:{root}\:{of}\:{b}−\mathrm{1}=\mathrm{0} \\ $$$$\therefore{b}^{\mathrm{2}} +{b}+\mathrm{1}\Rightarrow{b}=\omega,\omega^{\mathrm{2}} \\ $$$${b}=\omega:\:{b}^{\mathrm{2022}} +\frac{\mathrm{1}}{{b}^{\mathrm{2022}} }=\omega^{\mathrm{2022}} +\frac{\mathrm{1}}{\omega^{\mathrm{2022}} } \\ $$$$\:\:\:\:\:\:\:\:\:=\left(\omega^{\mathrm{3}} \right)^{\mathrm{674}} +\frac{\mathrm{1}}{\left(\omega^{\mathrm{3}} \right)^{\mathrm{674}} }=\mathrm{1}^{\mathrm{674}} +\frac{\mathrm{1}}{\mathrm{1}^{\mathrm{674}} }=\mathrm{2} \\ $$$${b}=\omega^{\mathrm{2}} :\:\:{b}^{\mathrm{2022}} +\frac{\mathrm{1}}{{b}^{\mathrm{2022}} }=\left(\omega^{\mathrm{2}} \right)^{\mathrm{2022}} +\frac{\mathrm{1}}{\left(\omega^{\mathrm{2}} \right)^{\mathrm{2022}} } \\ $$$$=\left(\omega^{\mathrm{3}} \right)^{\mathrm{2}×\mathrm{674}} +\frac{\mathrm{1}}{\left(\omega^{\mathrm{3}} \right)^{\mathrm{2}×\mathrm{674}} }=\mathrm{1}^{\mathrm{2}×\mathrm{674}} +\frac{\mathrm{1}}{\mathrm{1}^{\mathrm{2}×\mathrm{674}} }=\mathrm{2} \\ $$$${Hence}\:{in}\:{both}\:{cases}: \\ $$$$\:{b}^{\mathrm{2022}} +\frac{\mathrm{1}}{{b}^{\mathrm{2022}} }=\mathrm{2} \\ $$

Commented by mathlove last updated on 19/Feb/22