Menu Close

Question-166392

Tinku Tara 0 Comments
Pin




Question Number 166392 by leicianocosta last updated on 19/Feb/22
Answered by som(math1967) last updated on 19/Feb/22
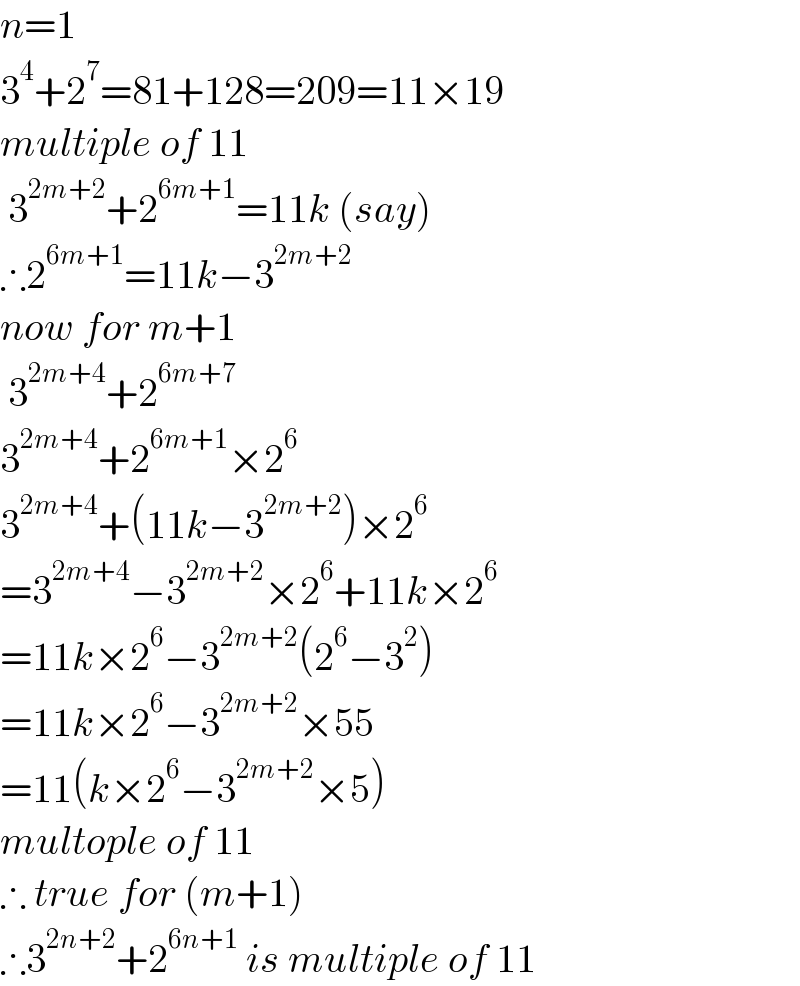
n=1 3^4 +2^7 =81+128=209=11×19 multiple of 11 3^(2m+2) +2^(6m+1) =11k (say) ∴2^(6m+1) =11k−3^(2m+2) now for m+1 3^(2m+4) +2^(6m+7) 3^(2m+4) +2^(6m+1) ×2^6 3^(2m+4) +(11k−3^(2m+2) )×2^6 =3^(2m+4) −3^(2m+2) ×2^6 +11k×2^6 =11k×2^6 −3^(2m+2) (2^6 −3^2 ) =11k×2^6 −3^(2m+2) ×55 =11(k×2^6 −3^(2m+2) ×5) multople of 11 ∴ true for (m+1) ∴3^(2n+2) +2^(6n+1) is multiple of 11
$${n}=\mathrm{1} \\ $$$$\mathrm{3}^{\mathrm{4}} +\mathrm{2}^{\mathrm{7}} =\mathrm{81}+\mathrm{128}=\mathrm{209}=\mathrm{11}×\mathrm{19} \\ $$$${multiple}\:{of}\:\mathrm{11} \\ $$$$\:\mathrm{3}^{\mathrm{2}{m}+\mathrm{2}} +\mathrm{2}^{\mathrm{6}{m}+\mathrm{1}} =\mathrm{11}{k}\:\left({say}\right) \\ $$$$\therefore\mathrm{2}^{\mathrm{6}{m}+\mathrm{1}} =\mathrm{11}{k}−\mathrm{3}^{\mathrm{2}{m}+\mathrm{2}} \\ $$$${now}\:{for}\:{m}+\mathrm{1} \\ $$$$\:\mathrm{3}^{\mathrm{2}{m}+\mathrm{4}} +\mathrm{2}^{\mathrm{6}{m}+\mathrm{7}} \\ $$$$\mathrm{3}^{\mathrm{2}{m}+\mathrm{4}} +\mathrm{2}^{\mathrm{6}{m}+\mathrm{1}} ×\mathrm{2}^{\mathrm{6}} \\ $$$$\mathrm{3}^{\mathrm{2}{m}+\mathrm{4}} +\left(\mathrm{11}{k}−\mathrm{3}^{\mathrm{2}{m}+\mathrm{2}} \right)×\mathrm{2}^{\mathrm{6}} \\ $$$$=\mathrm{3}^{\mathrm{2}{m}+\mathrm{4}} −\mathrm{3}^{\mathrm{2}{m}+\mathrm{2}} ×\mathrm{2}^{\mathrm{6}} +\mathrm{11}{k}×\mathrm{2}^{\mathrm{6}} \\ $$$$=\mathrm{11}{k}×\mathrm{2}^{\mathrm{6}} −\mathrm{3}^{\mathrm{2}{m}+\mathrm{2}} \left(\mathrm{2}^{\mathrm{6}} −\mathrm{3}^{\mathrm{2}} \right) \\ $$$$=\mathrm{11}{k}×\mathrm{2}^{\mathrm{6}} −\mathrm{3}^{\mathrm{2}{m}+\mathrm{2}} ×\mathrm{55} \\ $$$$=\mathrm{11}\left({k}×\mathrm{2}^{\mathrm{6}} −\mathrm{3}^{\mathrm{2}{m}+\mathrm{2}} ×\mathrm{5}\right) \\ $$$${multople}\:{of}\:\mathrm{11} \\ $$$$\therefore\:{true}\:{for}\:\left({m}+\mathrm{1}\right) \\ $$$$\therefore\mathrm{3}^{\mathrm{2}{n}+\mathrm{2}} +\mathrm{2}^{\mathrm{6}{n}+\mathrm{1}} \:{is}\:{multiple}\:{of}\:\mathrm{11} \\ $$
Answered by JDamian last updated on 19/Feb/22
q=3^(2n+2) +2^(6n+1) =3^(2(n+1)) +2^(5n+n+1) q=9^(n+1) +2^(n+1) ∙32^n r=q mod 11 r=[(11−2)^(n+1) +2^(n+1) (33−1)^n ] mod 11= =[(−2)^(n+1) +2∙2^n ∙(−1)^n ] mod 11= =[(−2)(−2)^n +2∙(−2)^n ] mod 11= =[(−2+2)∙(−2)^n ] mod 11= =[0∙(−2)^n ] mod 11= =0
$${q}=\mathrm{3}^{\mathrm{2}{n}+\mathrm{2}} +\mathrm{2}^{\mathrm{6}{n}+\mathrm{1}} =\mathrm{3}^{\mathrm{2}\left({n}+\mathrm{1}\right)} +\mathrm{2}^{\mathrm{5}{n}+{n}+\mathrm{1}} \\ $$$${q}=\mathrm{9}^{{n}+\mathrm{1}} +\mathrm{2}^{{n}+\mathrm{1}} \centerdot\mathrm{32}^{{n}} \\ $$$$ \\ $$$${r}={q}\:{mod}\:\mathrm{11} \\ $$$${r}=\left[\left(\mathrm{11}−\mathrm{2}\right)^{{n}+\mathrm{1}} +\mathrm{2}^{{n}+\mathrm{1}} \left(\mathrm{33}−\mathrm{1}\right)^{{n}} \right]\:{mod}\:\mathrm{11}= \\ $$$$=\left[\left(−\mathrm{2}\right)^{{n}+\mathrm{1}} +\mathrm{2}\centerdot\mathrm{2}^{{n}} \centerdot\left(−\mathrm{1}\right)^{{n}} \right]\:{mod}\:\mathrm{11}= \\ $$$$=\left[\left(−\mathrm{2}\right)\left(−\mathrm{2}\right)^{\boldsymbol{{n}}} +\mathrm{2}\centerdot\left(−\mathrm{2}\right)^{\boldsymbol{{n}}} \right]\:{mod}\:\mathrm{11}= \\ $$$$=\left[\left(−\mathrm{2}+\mathrm{2}\right)\centerdot\left(−\mathrm{2}\right)^{{n}} \right]\:{mod}\:\mathrm{11}= \\ $$$$=\left[\mathrm{0}\centerdot\left(−\mathrm{2}\right)^{{n}} \right]\:{mod}\:\mathrm{11}= \\ $$$$=\mathrm{0} \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *