Question Number 166530 by amin96 last updated on 21/Feb/22
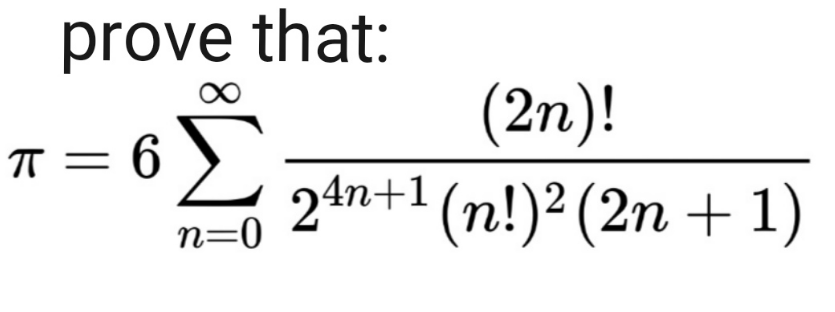
Answered by qaz last updated on 22/Feb/22
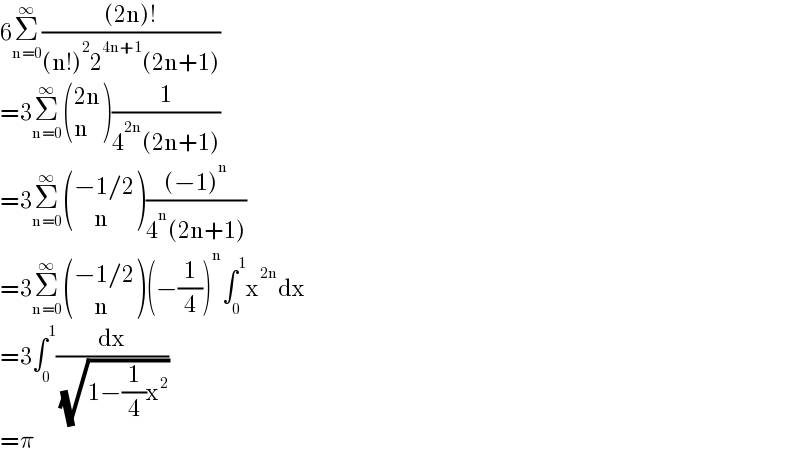
$$\mathrm{6}\underset{\mathrm{n}=\mathrm{0}} {\overset{\infty} {\sum}}\frac{\left(\mathrm{2n}\right)!}{\left(\mathrm{n}!\right)^{\mathrm{2}} \mathrm{2}^{\mathrm{4n}+\mathrm{1}} \left(\mathrm{2n}+\mathrm{1}\right)} \\ $$$$=\mathrm{3}\underset{\mathrm{n}=\mathrm{0}} {\overset{\infty} {\sum}}\begin{pmatrix}{\mathrm{2n}}\\{\mathrm{n}}\end{pmatrix}\frac{\mathrm{1}}{\mathrm{4}^{\mathrm{2n}} \left(\mathrm{2n}+\mathrm{1}\right)} \\ $$$$=\mathrm{3}\underset{\mathrm{n}=\mathrm{0}} {\overset{\infty} {\sum}}\begin{pmatrix}{−\mathrm{1}/\mathrm{2}}\\{\:\:\:\:\:\mathrm{n}}\end{pmatrix}\frac{\left(−\mathrm{1}\right)^{\mathrm{n}} }{\mathrm{4}^{\mathrm{n}} \left(\mathrm{2n}+\mathrm{1}\right)} \\ $$$$=\mathrm{3}\underset{\mathrm{n}=\mathrm{0}} {\overset{\infty} {\sum}}\begin{pmatrix}{−\mathrm{1}/\mathrm{2}}\\{\:\:\:\:\:\mathrm{n}}\end{pmatrix}\left(−\frac{\mathrm{1}}{\mathrm{4}}\right)^{\mathrm{n}} \int_{\mathrm{0}} ^{\mathrm{1}} \mathrm{x}^{\mathrm{2n}} \mathrm{dx} \\ $$$$=\mathrm{3}\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\mathrm{dx}}{\:\sqrt{\mathrm{1}−\frac{\mathrm{1}}{\mathrm{4}}\mathrm{x}^{\mathrm{2}} }} \\ $$$$=\pi \\ $$
