Question Number 166701 by ajfour last updated on 25/Feb/22
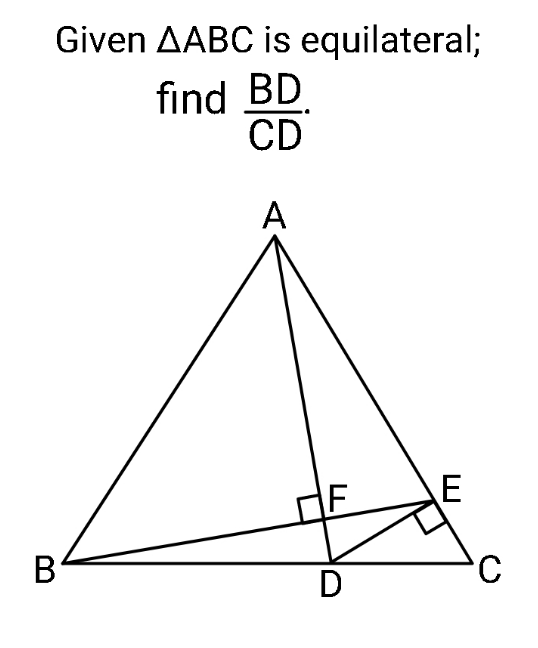
Answered by mr W last updated on 26/Feb/22
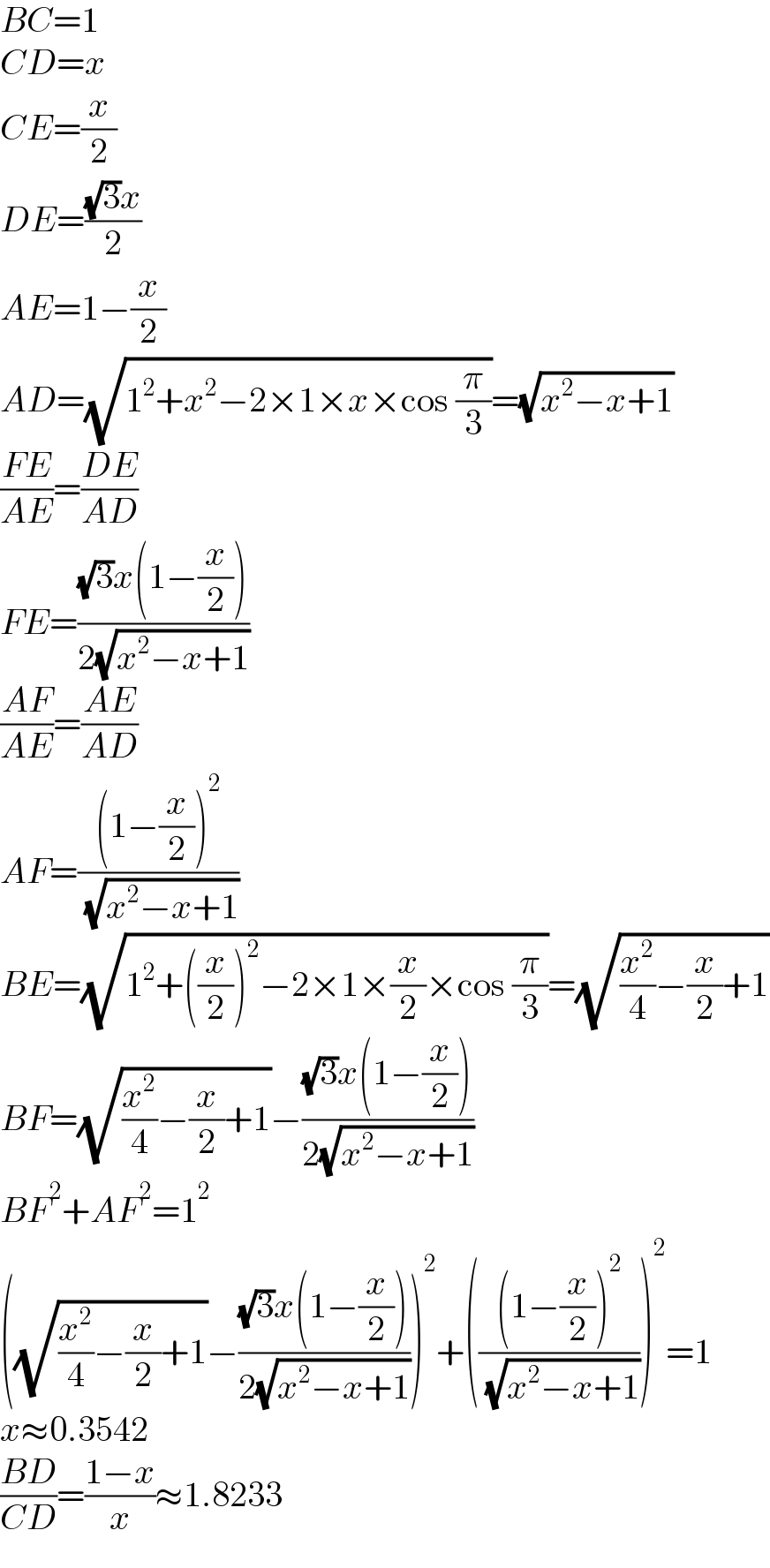
$${BC}=\mathrm{1} \\ $$$${CD}={x} \\ $$$${CE}=\frac{{x}}{\mathrm{2}} \\ $$$${DE}=\frac{\sqrt{\mathrm{3}}{x}}{\mathrm{2}} \\ $$$${AE}=\mathrm{1}−\frac{{x}}{\mathrm{2}} \\ $$$${AD}=\sqrt{\mathrm{1}^{\mathrm{2}} +{x}^{\mathrm{2}} −\mathrm{2}×\mathrm{1}×{x}×\mathrm{cos}\:\frac{\pi}{\mathrm{3}}}=\sqrt{{x}^{\mathrm{2}} −{x}+\mathrm{1}} \\ $$$$\frac{{FE}}{{AE}}=\frac{{DE}}{{AD}} \\ $$$${FE}=\frac{\sqrt{\mathrm{3}}{x}\left(\mathrm{1}−\frac{{x}}{\mathrm{2}}\right)}{\mathrm{2}\sqrt{{x}^{\mathrm{2}} −{x}+\mathrm{1}}} \\ $$$$\frac{{AF}}{{AE}}=\frac{{AE}}{{AD}} \\ $$$${AF}=\frac{\left(\mathrm{1}−\frac{{x}}{\mathrm{2}}\right)^{\mathrm{2}} }{\:\sqrt{{x}^{\mathrm{2}} −{x}+\mathrm{1}}} \\ $$$${BE}=\sqrt{\mathrm{1}^{\mathrm{2}} +\left(\frac{{x}}{\mathrm{2}}\right)^{\mathrm{2}} −\mathrm{2}×\mathrm{1}×\frac{{x}}{\mathrm{2}}×\mathrm{cos}\:\frac{\pi}{\mathrm{3}}}=\sqrt{\frac{{x}^{\mathrm{2}} }{\mathrm{4}}−\frac{{x}}{\mathrm{2}}+\mathrm{1}} \\ $$$${BF}=\sqrt{\frac{{x}^{\mathrm{2}} }{\mathrm{4}}−\frac{{x}}{\mathrm{2}}+\mathrm{1}}−\frac{\sqrt{\mathrm{3}}{x}\left(\mathrm{1}−\frac{{x}}{\mathrm{2}}\right)}{\mathrm{2}\sqrt{{x}^{\mathrm{2}} −{x}+\mathrm{1}}} \\ $$$${BF}^{\mathrm{2}} +{AF}^{\mathrm{2}} =\mathrm{1}^{\mathrm{2}} \\ $$$$\left(\sqrt{\frac{{x}^{\mathrm{2}} }{\mathrm{4}}−\frac{{x}}{\mathrm{2}}+\mathrm{1}}−\frac{\sqrt{\mathrm{3}}{x}\left(\mathrm{1}−\frac{{x}}{\mathrm{2}}\right)}{\mathrm{2}\sqrt{{x}^{\mathrm{2}} −{x}+\mathrm{1}}}\right)^{\mathrm{2}} +\left(\frac{\left(\mathrm{1}−\frac{{x}}{\mathrm{2}}\right)^{\mathrm{2}} }{\:\sqrt{{x}^{\mathrm{2}} −{x}+\mathrm{1}}}\right)^{\mathrm{2}} =\mathrm{1} \\ $$$${x}\approx\mathrm{0}.\mathrm{3542} \\ $$$$\frac{{BD}}{{CD}}=\frac{\mathrm{1}−{x}}{{x}}\approx\mathrm{1}.\mathrm{8233} \\ $$
Commented by Tawa11 last updated on 26/Feb/22

$$\mathrm{Great}\:\mathrm{sir} \\ $$
Commented by ajfour last updated on 26/Feb/22
Thanks sir. I got it exact easily somehow!
Commented by mr W last updated on 26/Feb/22
$${very}\:{nice}\:{solution}! \\ $$
Answered by ajfour last updated on 26/Feb/22
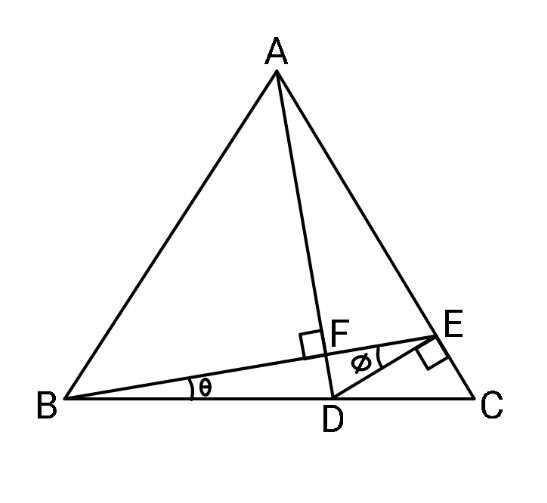
Commented by ajfour last updated on 26/Feb/22
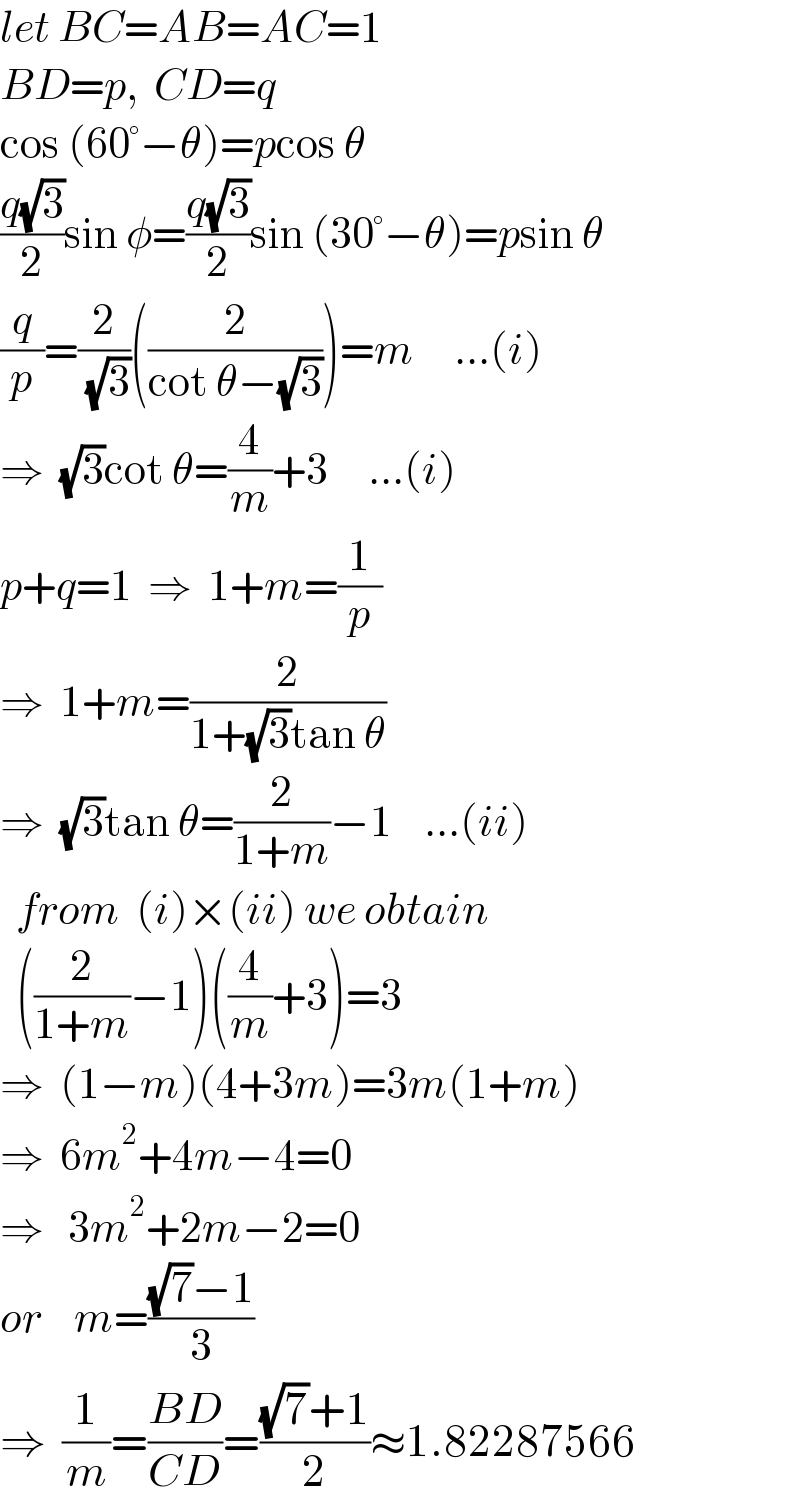
$${let}\:{BC}={AB}={AC}=\mathrm{1} \\ $$$${BD}={p},\:\:{CD}={q} \\ $$$$\mathrm{cos}\:\left(\mathrm{60}°−\theta\right)={p}\mathrm{cos}\:\theta \\ $$$$\frac{{q}\sqrt{\mathrm{3}}}{\mathrm{2}}\mathrm{sin}\:\phi=\frac{{q}\sqrt{\mathrm{3}}}{\mathrm{2}}\mathrm{sin}\:\left(\mathrm{30}°−\theta\right)={p}\mathrm{sin}\:\theta \\ $$$$\frac{{q}}{{p}}=\frac{\mathrm{2}}{\:\sqrt{\mathrm{3}}}\left(\frac{\mathrm{2}}{\mathrm{cot}\:\theta−\sqrt{\mathrm{3}}}\right)={m}\:\:\:\:\:…\left({i}\right) \\ $$$$\Rightarrow\:\:\sqrt{\mathrm{3}}\mathrm{cot}\:\theta=\frac{\mathrm{4}}{{m}}+\mathrm{3}\:\:\:\:\:…\left({i}\right) \\ $$$${p}+{q}=\mathrm{1}\:\:\Rightarrow\:\:\mathrm{1}+{m}=\frac{\mathrm{1}}{{p}} \\ $$$$\Rightarrow\:\:\mathrm{1}+{m}=\frac{\mathrm{2}}{\mathrm{1}+\sqrt{\mathrm{3}}\mathrm{tan}\:\theta} \\ $$$$\Rightarrow\:\:\sqrt{\mathrm{3}}\mathrm{tan}\:\theta=\frac{\mathrm{2}}{\mathrm{1}+{m}}−\mathrm{1}\:\:\:\:…\left({ii}\right) \\ $$$$\:\:{from}\:\:\left({i}\right)×\left({ii}\right)\:{we}\:{obtain} \\ $$$$\:\:\left(\frac{\mathrm{2}}{\mathrm{1}+{m}}−\mathrm{1}\right)\left(\frac{\mathrm{4}}{{m}}+\mathrm{3}\right)=\mathrm{3} \\ $$$$\Rightarrow\:\:\left(\mathrm{1}−{m}\right)\left(\mathrm{4}+\mathrm{3}{m}\right)=\mathrm{3}{m}\left(\mathrm{1}+{m}\right) \\ $$$$\Rightarrow\:\:\mathrm{6}{m}^{\mathrm{2}} +\mathrm{4}{m}−\mathrm{4}=\mathrm{0} \\ $$$$\Rightarrow\:\:\:\mathrm{3}{m}^{\mathrm{2}} +\mathrm{2}{m}−\mathrm{2}=\mathrm{0} \\ $$$${or}\:\:\:\:{m}=\frac{\sqrt{\mathrm{7}}−\mathrm{1}}{\mathrm{3}}\:\: \\ $$$$\Rightarrow\:\:\frac{\mathrm{1}}{{m}}=\frac{{BD}}{{CD}}=\frac{\sqrt{\mathrm{7}}+\mathrm{1}}{\mathrm{2}}\approx\mathrm{1}.\mathrm{82287566} \\ $$
Commented by Tawa11 last updated on 03/Mar/22

$$\mathrm{Great}\:\mathrm{sir} \\ $$
