Question Number 166779 by ajfour last updated on 27/Feb/22
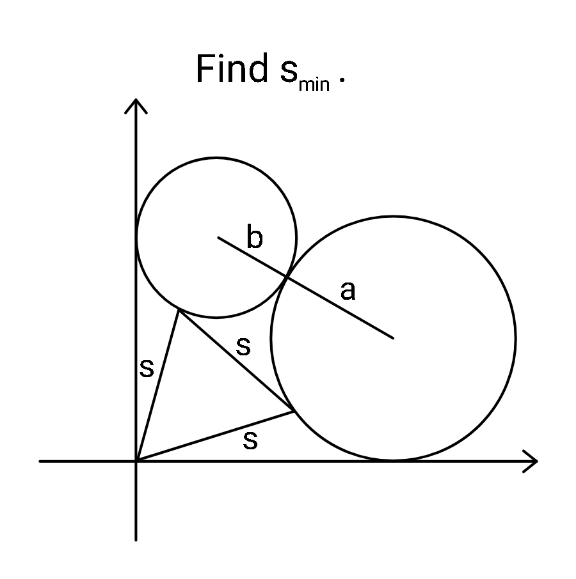
Answered by mr W last updated on 27/Feb/22
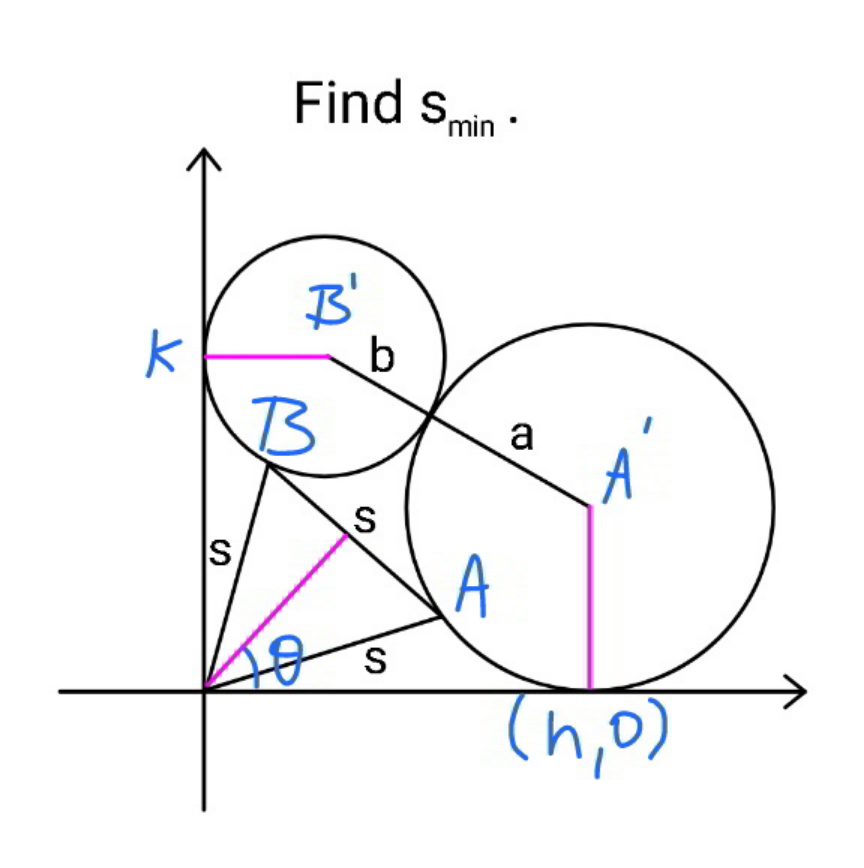
Commented by mr W last updated on 28/Feb/22
![A′(h,a) B′(b,k) (h−b)^2 +(k−a)^2 =(a+b)^2 k^2 −2ak+h^2 −2b(h+a)=0 ⇒k=a+(√((a+2b−h)(h+a))) x_A =(s/2)((√3)cos θ+sin θ) y_A =(s/2)((√3)sin θ−cos θ) x_B =(s/2)((√3)cos θ−sin θ) y_B =(s/2)((√3)sin θ+cos θ) ((s/2)((√3)cos θ+sin θ)−h)^2 +((s/2)((√3)sin θ−cos θ)−a)^2 =a^2 s^2 −[((√3)h−a)cos θ+((√3)a+h)sin θ]s+h^2 =0 ...(i) ((s/2)((√3)cos θ−sin θ)−b)^2 +((s/2)((√3)sin θ+cos θ)−k)^2 =b^2 s^2 −[((√3)b+k)cos θ+((√3)k−b)sin θ]s+k^2 =0 ...(ii) ((√3)h−a)cos θ+((√3)a+h)sin θ=((s^2 +h^2 )/s) cos (θ−tan^(−1) (((√3)a+h)/( (√3)h−a)))=((s^2 +h^2 )/( 2s(√(h^2 +a^2 )))) ⇒θ=tan^(−1) (((√3)a+h)/( (√3)h−a))+cos^(−1) ((s^2 +h^2 )/( 2s(√(h^2 +a^2 )))) s^2 −2s(√(k^2 +b^2 )) cos (θ−tan^(−1) (((√3)k−b)/( (√3)b+k)))+k^2 =0 s^2 −2s(√(k^2 +b^2 )) cos (cos^(−1) ((s^2 −h^2 )/( 2s(√(h^2 +a^2 ))))+tan^(−1) (((√3)a+h)/( (√3)h−a))−tan^(−1) (((√3)k−b)/( (√3)b+k)))+k^2 =0 ...](https://www.tinkutara.com/question/Q166781.png)
$${A}'\left({h},{a}\right) \\ $$$${B}'\left({b},{k}\right) \\ $$$$\left({h}−{b}\right)^{\mathrm{2}} +\left({k}−{a}\right)^{\mathrm{2}} =\left({a}+{b}\right)^{\mathrm{2}} \\ $$$${k}^{\mathrm{2}} −\mathrm{2}{ak}+{h}^{\mathrm{2}} −\mathrm{2}{b}\left({h}+{a}\right)=\mathrm{0} \\ $$$$\Rightarrow{k}={a}+\sqrt{\left({a}+\mathrm{2}{b}−{h}\right)\left({h}+{a}\right)} \\ $$$${x}_{{A}} =\frac{{s}}{\mathrm{2}}\left(\sqrt{\mathrm{3}}\mathrm{cos}\:\theta+\mathrm{sin}\:\theta\right) \\ $$$${y}_{{A}} =\frac{{s}}{\mathrm{2}}\left(\sqrt{\mathrm{3}}\mathrm{sin}\:\theta−\mathrm{cos}\:\theta\right) \\ $$$${x}_{{B}} =\frac{{s}}{\mathrm{2}}\left(\sqrt{\mathrm{3}}\mathrm{cos}\:\theta−\mathrm{sin}\:\theta\right) \\ $$$${y}_{{B}} =\frac{{s}}{\mathrm{2}}\left(\sqrt{\mathrm{3}}\mathrm{sin}\:\theta+\mathrm{cos}\:\theta\right) \\ $$$$\left(\frac{{s}}{\mathrm{2}}\left(\sqrt{\mathrm{3}}\mathrm{cos}\:\theta+\mathrm{sin}\:\theta\right)−{h}\right)^{\mathrm{2}} +\left(\frac{{s}}{\mathrm{2}}\left(\sqrt{\mathrm{3}}\mathrm{sin}\:\theta−\mathrm{cos}\:\theta\right)−{a}\right)^{\mathrm{2}} ={a}^{\mathrm{2}} \\ $$$${s}^{\mathrm{2}} −\left[\left(\sqrt{\mathrm{3}}{h}−{a}\right)\mathrm{cos}\:\theta+\left(\sqrt{\mathrm{3}}{a}+{h}\right)\mathrm{sin}\:\theta\right]{s}+{h}^{\mathrm{2}} =\mathrm{0}\:\:\:…\left({i}\right) \\ $$$$\left(\frac{{s}}{\mathrm{2}}\left(\sqrt{\mathrm{3}}\mathrm{cos}\:\theta−\mathrm{sin}\:\theta\right)−{b}\right)^{\mathrm{2}} +\left(\frac{{s}}{\mathrm{2}}\left(\sqrt{\mathrm{3}}\mathrm{sin}\:\theta+\mathrm{cos}\:\theta\right)−{k}\right)^{\mathrm{2}} ={b}^{\mathrm{2}} \\ $$$${s}^{\mathrm{2}} −\left[\left(\sqrt{\mathrm{3}}{b}+{k}\right)\mathrm{cos}\:\theta+\left(\sqrt{\mathrm{3}}{k}−{b}\right)\mathrm{sin}\:\theta\right]{s}+{k}^{\mathrm{2}} =\mathrm{0}\:\:\:…\left({ii}\right) \\ $$$$ \\ $$$$\left(\sqrt{\mathrm{3}}{h}−{a}\right)\mathrm{cos}\:\theta+\left(\sqrt{\mathrm{3}}{a}+{h}\right)\mathrm{sin}\:\theta=\frac{{s}^{\mathrm{2}} +{h}^{\mathrm{2}} }{{s}} \\ $$$$\mathrm{cos}\:\left(\theta−\mathrm{tan}^{−\mathrm{1}} \frac{\sqrt{\mathrm{3}}{a}+{h}}{\:\sqrt{\mathrm{3}}{h}−{a}}\right)=\frac{{s}^{\mathrm{2}} +{h}^{\mathrm{2}} }{\:\mathrm{2}{s}\sqrt{{h}^{\mathrm{2}} +{a}^{\mathrm{2}} }} \\ $$$$\Rightarrow\theta=\mathrm{tan}^{−\mathrm{1}} \frac{\sqrt{\mathrm{3}}{a}+{h}}{\:\sqrt{\mathrm{3}}{h}−{a}}+\mathrm{cos}^{−\mathrm{1}} \frac{{s}^{\mathrm{2}} +{h}^{\mathrm{2}} }{\:\mathrm{2}{s}\sqrt{{h}^{\mathrm{2}} +{a}^{\mathrm{2}} }} \\ $$$$ \\ $$$${s}^{\mathrm{2}} −\mathrm{2}{s}\sqrt{{k}^{\mathrm{2}} +{b}^{\mathrm{2}} }\:\mathrm{cos}\:\left(\theta−\mathrm{tan}^{−\mathrm{1}} \frac{\sqrt{\mathrm{3}}{k}−{b}}{\:\sqrt{\mathrm{3}}{b}+{k}}\right)+{k}^{\mathrm{2}} =\mathrm{0} \\ $$$${s}^{\mathrm{2}} −\mathrm{2}{s}\sqrt{{k}^{\mathrm{2}} +{b}^{\mathrm{2}} }\:\mathrm{cos}\:\left(\mathrm{cos}^{−\mathrm{1}} \frac{{s}^{\mathrm{2}} −{h}^{\mathrm{2}} }{\:\mathrm{2}{s}\sqrt{{h}^{\mathrm{2}} +{a}^{\mathrm{2}} }}+\mathrm{tan}^{−\mathrm{1}} \frac{\sqrt{\mathrm{3}}{a}+{h}}{\:\sqrt{\mathrm{3}}{h}−{a}}−\mathrm{tan}^{−\mathrm{1}} \frac{\sqrt{\mathrm{3}}{k}−{b}}{\:\sqrt{\mathrm{3}}{b}+{k}}\right)+{k}^{\mathrm{2}} =\mathrm{0} \\ $$$$… \\ $$
Commented by ajfour last updated on 28/Feb/22
![(h−scos α)^2 +(a−ssin α)^2 =a^2 (b−ssin β)^2 +(k−scos β)^2 =b^2 ⇒ h=scos α+(√(a^2 −(a−ssin α)^2 )) k=scos β+(√(b^2 −(b−ssin β)^2 )) α+β=(π/6) ; α−β=2θ ⇒ α=(π/(12))+θ , β=(π/(12))−θ (h−b)^2 +(k−a)^2 =(a+b)^2 ⇒ {scos ((π/(12))+θ) −b+(√(a^2 −[a−ssin ((π/(12))+θ)]^2 ))}^2 +{scos ((π/(12))−θ)−a+(√(b^2 −[b−ssin ((π/(12))−θ)]^2 ))}^2 =(a+b)^2](https://www.tinkutara.com/question/Q166794.png)
$$\left({h}−{s}\mathrm{cos}\:\alpha\right)^{\mathrm{2}} +\left({a}−{s}\mathrm{sin}\:\alpha\right)^{\mathrm{2}} ={a}^{\mathrm{2}} \\ $$$$\left({b}−{s}\mathrm{sin}\:\beta\right)^{\mathrm{2}} +\left({k}−{s}\mathrm{cos}\:\beta\right)^{\mathrm{2}} ={b}^{\mathrm{2}} \\ $$$$\Rightarrow \\ $$$$\:\:{h}={s}\mathrm{cos}\:\alpha+\sqrt{{a}^{\mathrm{2}} −\left({a}−{s}\mathrm{sin}\:\alpha\right)^{\mathrm{2}} } \\ $$$$\:\:{k}={s}\mathrm{cos}\:\beta+\sqrt{{b}^{\mathrm{2}} −\left({b}−{s}\mathrm{sin}\:\beta\right)^{\mathrm{2}} } \\ $$$$\alpha+\beta=\frac{\pi}{\mathrm{6}}\:\:;\:\alpha−\beta=\mathrm{2}\theta \\ $$$$\Rightarrow\:\:\alpha=\frac{\pi}{\mathrm{12}}+\theta\:\:,\:\:\beta=\frac{\pi}{\mathrm{12}}−\theta \\ $$$$\left({h}−{b}\right)^{\mathrm{2}} +\left({k}−{a}\right)^{\mathrm{2}} =\left({a}+{b}\right)^{\mathrm{2}} \\ $$$$\Rightarrow \\ $$$$\left\{{s}\mathrm{cos}\:\left(\frac{\pi}{\mathrm{12}}+\theta\right)\:−{b}+\sqrt{{a}^{\mathrm{2}} −\left[{a}−{s}\mathrm{sin}\:\left(\frac{\pi}{\mathrm{12}}+\theta\right)\right]^{\mathrm{2}} }\right\}^{\mathrm{2}} \\ $$$$+\left\{{s}\mathrm{cos}\:\left(\frac{\pi}{\mathrm{12}}−\theta\right)−{a}+\sqrt{{b}^{\mathrm{2}} −\left[{b}−{s}\mathrm{sin}\:\left(\frac{\pi}{\mathrm{12}}−\theta\right)\right]^{\mathrm{2}} }\right\}^{\mathrm{2}} \\ $$$$\:\:\:\:=\left({a}+{b}\right)^{\mathrm{2}} \\ $$$$ \\ $$
Commented by mr W last updated on 28/Feb/22

$${very}\:{nice}\:{way}! \\ $$
Commented by Tawa11 last updated on 28/Feb/22

$$\mathrm{Great}\:\mathrm{sir} \\ $$
