Question-167840

Question Number 167840 by mathlove last updated on 27/Mar/22

Commented by cortano1 last updated on 27/Mar/22

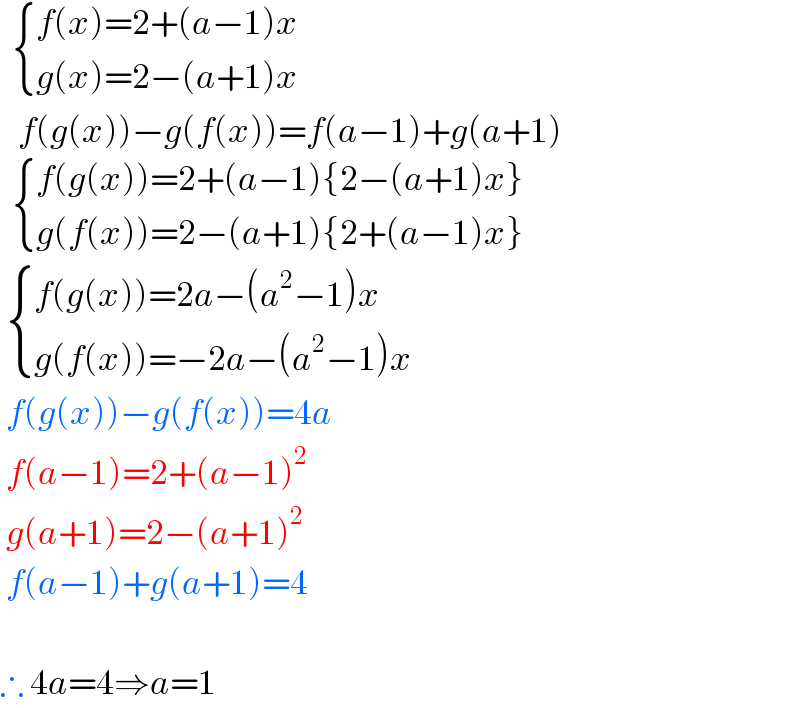
{ ((f(x)=2+(a−1)x)),((g(x)=2−(a+1)x)) :} f(g(x))−g(f(x))=f(a−1)+g(a+1) { ((f(g(x))=2+(a−1){2−(a+1)x})),((g(f(x))=2−(a+1){2+(a−1)x})) :} { ((f(g(x))=2a−(a^2 −1)x)),((g(f(x))=−2a−(a^2 −1)x)) :} f(g(x))−g(f(x))=4a f(a−1)=2+(a−1)^2 g(a+1)=2−(a+1)^2 f(a−1)+g(a+1)=4 ∴ 4a=4⇒a=1

$$\:\:\begin{cases}{{f}\left({x}\right)=\mathrm{2}+\left({a}−\mathrm{1}\right){x}}\\{{g}\left({x}\right)=\mathrm{2}−\left({a}+\mathrm{1}\right){x}}\end{cases} \\ $$$$\:\:\:{f}\left({g}\left({x}\right)\right)−{g}\left({f}\left({x}\right)\right)={f}\left({a}−\mathrm{1}\right)+{g}\left({a}+\mathrm{1}\right) \\ $$$$\:\:\begin{cases}{{f}\left({g}\left({x}\right)\right)=\mathrm{2}+\left({a}−\mathrm{1}\right)\left\{\mathrm{2}−\left({a}+\mathrm{1}\right){x}\right\}}\\{{g}\left({f}\left({x}\right)\right)=\mathrm{2}−\left({a}+\mathrm{1}\right)\left\{\mathrm{2}+\left({a}−\mathrm{1}\right){x}\right\}}\end{cases} \\ $$$$\:\begin{cases}{{f}\left({g}\left({x}\right)\right)=\mathrm{2}{a}−\left({a}^{\mathrm{2}} −\mathrm{1}\right){x}}\\{{g}\left({f}\left({x}\right)\right)=−\mathrm{2}{a}−\left({a}^{\mathrm{2}} −\mathrm{1}\right){x}}\end{cases} \\ $$$$\:{f}\left({g}\left({x}\right)\right)−{g}\left({f}\left({x}\right)\right)=\mathrm{4}{a} \\ $$$$\:{f}\left({a}−\mathrm{1}\right)=\mathrm{2}+\left({a}−\mathrm{1}\right)^{\mathrm{2}} \\ $$$$\:{g}\left({a}+\mathrm{1}\right)=\mathrm{2}−\left({a}+\mathrm{1}\right)^{\mathrm{2}} \\ $$$$\:{f}\left({a}−\mathrm{1}\right)+{g}\left({a}+\mathrm{1}\right)=\mathrm{4} \\ $$$$ \\ $$$$\therefore\:\mathrm{4}{a}=\mathrm{4}\Rightarrow{a}=\mathrm{1} \\ $$

Facebook Tweet Pin

Leave a Reply Cancel reply