Question Number 168982 by Tawa11 last updated on 22/Apr/22

Commented by mr W last updated on 22/Apr/22

$${all}\:{answers}\:{seem}\:{to}\:{be}\:{wrong}. \\ $$
Answered by mr W last updated on 22/Apr/22
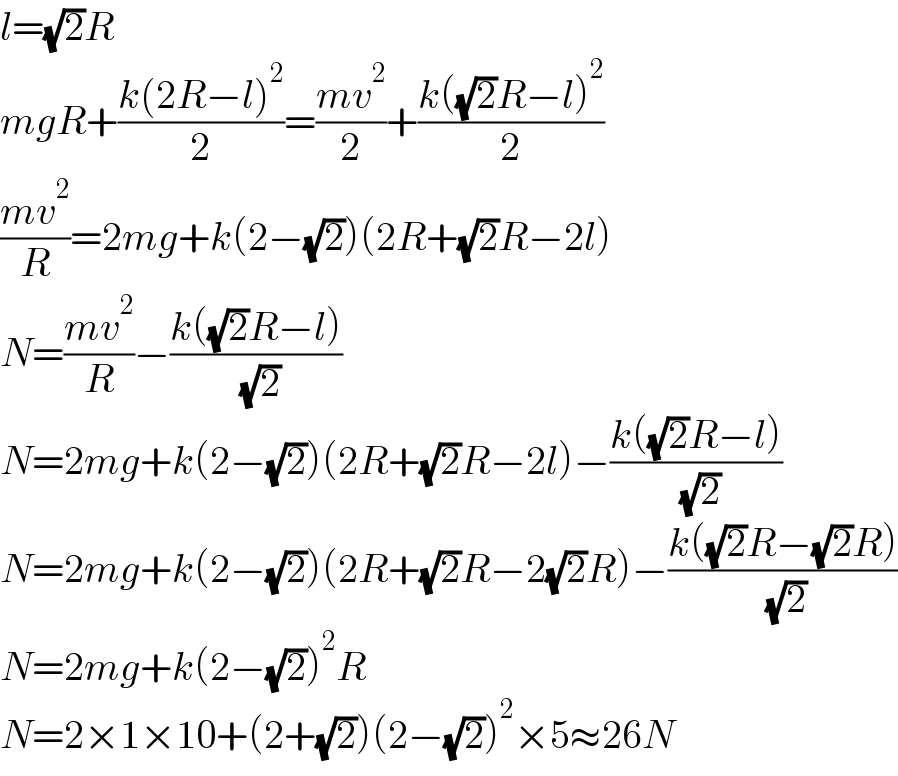
$${l}=\sqrt{\mathrm{2}}{R} \\ $$$${mgR}+\frac{{k}\left(\mathrm{2}{R}−{l}\right)^{\mathrm{2}} }{\mathrm{2}}=\frac{{mv}^{\mathrm{2}} }{\mathrm{2}}+\frac{{k}\left(\sqrt{\mathrm{2}}{R}−{l}\right)^{\mathrm{2}} }{\mathrm{2}} \\ $$$$\frac{{mv}^{\mathrm{2}} }{{R}}=\mathrm{2}{mg}+{k}\left(\mathrm{2}−\sqrt{\mathrm{2}}\right)\left(\mathrm{2}{R}+\sqrt{\mathrm{2}}{R}−\mathrm{2}{l}\right) \\ $$$${N}=\frac{{mv}^{\mathrm{2}} }{{R}}−\frac{{k}\left(\sqrt{\mathrm{2}}{R}−{l}\right)}{\:\sqrt{\mathrm{2}}} \\ $$$${N}=\mathrm{2}{mg}+{k}\left(\mathrm{2}−\sqrt{\mathrm{2}}\right)\left(\mathrm{2}{R}+\sqrt{\mathrm{2}}{R}−\mathrm{2}{l}\right)−\frac{{k}\left(\sqrt{\mathrm{2}}{R}−{l}\right)}{\:\sqrt{\mathrm{2}}} \\ $$$${N}=\mathrm{2}{mg}+{k}\left(\mathrm{2}−\sqrt{\mathrm{2}}\right)\left(\mathrm{2}{R}+\sqrt{\mathrm{2}}{R}−\mathrm{2}\sqrt{\mathrm{2}}{R}\right)−\frac{{k}\left(\sqrt{\mathrm{2}}{R}−\sqrt{\mathrm{2}}{R}\right)}{\:\sqrt{\mathrm{2}}} \\ $$$${N}=\mathrm{2}{mg}+{k}\left(\mathrm{2}−\sqrt{\mathrm{2}}\right)^{\mathrm{2}} {R} \\ $$$${N}=\mathrm{2}×\mathrm{1}×\mathrm{10}+\left(\mathrm{2}+\sqrt{\mathrm{2}}\right)\left(\mathrm{2}−\sqrt{\mathrm{2}}\right)^{\mathrm{2}} ×\mathrm{5}\approx\mathrm{26}{N} \\ $$
Commented by mr W last updated on 23/Apr/22

$${am}\:{I}\:{right}? \\ $$
Commented by Tawa11 last updated on 22/Apr/22
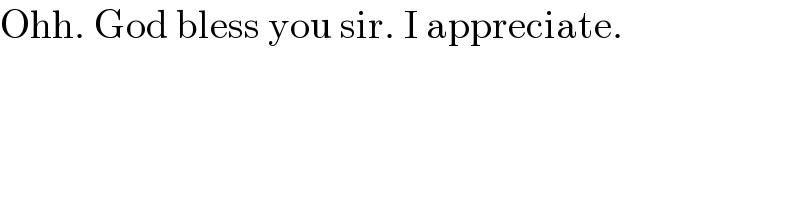
$$\mathrm{Ohh}.\:\mathrm{God}\:\mathrm{bless}\:\mathrm{you}\:\mathrm{sir}.\:\mathrm{I}\:\mathrm{appreciate}. \\ $$
Commented by Tawa11 last updated on 22/Apr/22
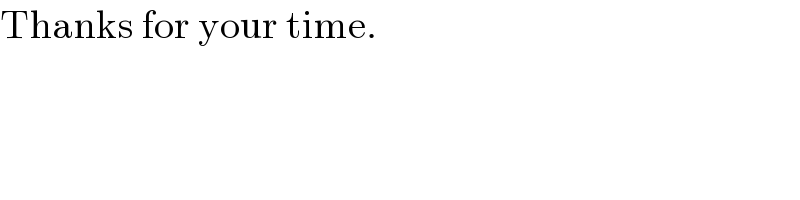
$$\mathrm{Thanks}\:\mathrm{for}\:\mathrm{your}\:\mathrm{time}. \\ $$
Commented by Tawa11 last updated on 23/Apr/22

$$\mathrm{They}\:\mathrm{choose}\:\:\mathrm{30}\:\:\mathrm{sir}. \\ $$
