Question Number 169078 by MikeH last updated on 23/Apr/22

Answered by mahdipoor last updated on 23/Apr/22
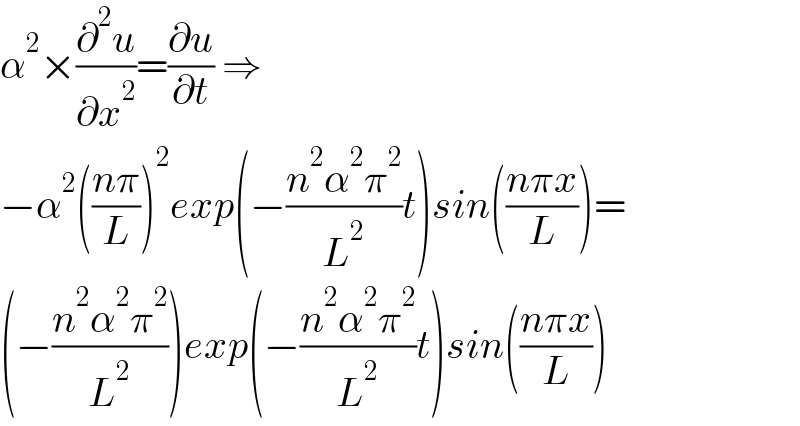
$$\alpha^{\mathrm{2}} ×\frac{\partial^{\mathrm{2}} {u}}{\partial{x}^{\mathrm{2}} }=\frac{\partial{u}}{\partial{t}}\:\Rightarrow \\ $$$$−\alpha^{\mathrm{2}} \left(\frac{{n}\pi}{{L}}\right)^{\mathrm{2}} {exp}\left(−\frac{{n}^{\mathrm{2}} \alpha^{\mathrm{2}} \pi^{\mathrm{2}} }{{L}^{\mathrm{2}} }{t}\right){sin}\left(\frac{{n}\pi{x}}{{L}}\right)= \\ $$$$\left(−\frac{{n}^{\mathrm{2}} \alpha^{\mathrm{2}} \pi^{\mathrm{2}} }{{L}^{\mathrm{2}} }\right){exp}\left(−\frac{{n}^{\mathrm{2}} \alpha^{\mathrm{2}} \pi^{\mathrm{2}} }{{L}^{\mathrm{2}} }{t}\right){sin}\left(\frac{{n}\pi{x}}{{L}}\right) \\ $$
Commented by MikeH last updated on 24/Apr/22

$$\mathrm{perfect} \\ $$
