Question-169211

Question Number 169211 by 0731619 last updated on 26/Apr/22

Commented by infinityaction last updated on 26/Apr/22

\sin (α + β) = \frac{2 m n}{m^{2} + n^{2}}

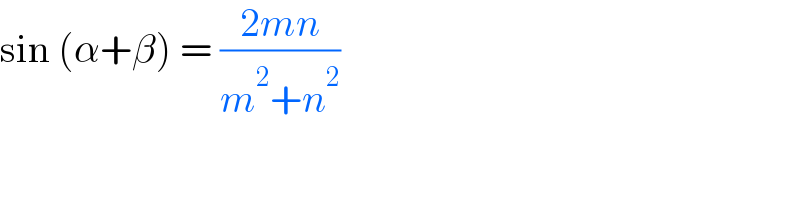
Commented by 0731619 last updated on 26/Apr/22

s o l u t i o n p l z

Commented by infinityaction last updated on 26/Apr/22

\sin^{2} α + \sin^{2} β + 2 \sin (α) \sin (β) = n^{2} . . (1)

\cos^{2} α + \cos^{2} β + 2 \cos (α) \cos (β) = m^{2} \dots . (2)

{e q}^{n} . (1) + {e q}^{n} . (2)

2 + 2 (\sin α \sin β + \cos α \cos β) = n^{2} + m^{2} \dots (3)

2 {1 + \cos (α - β)} = m^{2} + n^{2} \dots . . (4)

{e q}^{n} . (1) \times {e q}^{n} . (2)

(\sin α + \sin β) (\cos α + \cos β) = m n

\cos α \sin α + \cos β \sin β + \cos α \sin β + \cos β \sin α = m n

\sin 2 α + \sin 2 β + 2 \sin (α + β) = 2 m n

2 \sin (α + β) \cdot \cos (α - β) + 2 \sin (α + β) = 2 m n

2 \sin (α + β) {\cos (α - β) + 1} = 2 m n \dots . (5)

\frac{{e q}^{n} . (5)}{{e q}^{n} . (4)}

\frac{2 \sin (α + β) {\cos (α - β) + 1}}{2 {1 + \cos (α - β)}} = \frac{2 m n}{m^{2} + n^{2}}

\sin (α + β) = \frac{2 m n}{m^{2} + n^{2}}

Commented by som(math1967) last updated on 26/Apr/22

s i n α + s i n β = m

\Rightarrow 2 s i n (\frac{α + β}{2}) c o s (\frac{α - β}{2}) = m \dots . i)

c o s α + c o s β = m

2 c o s (\frac{α + β}{2}) c o s (\frac{α - β}{2}) = n \dots . i i)

i) \times i i

2 s i n (\frac{α + β}{2}) c o s (\frac{α + β}{2}) 2 {c o s}^{2} (\frac{α - β}{2}) = m n

\Rightarrow s i n (α + β) = \frac{m n}{2 {c o s}^{2} (\frac{α - β}{2})}

{(i)}^{2} + {(i i)}^{2}

4 {c o s}^{2} (\frac{α - β}{2}) [{s i n}^{2} (\frac{α + β}{2}) + {c o s}^{2} (\frac{α + β}{2})]

= m^{2} + n^{2}

∴ 2 {c o s}^{2} (\frac{α - β}{2}) = \frac{m^{2} + n^{2}}{2}

∴ s i n (α + β) = \frac{m n}{2 {c o s}^{2} (\frac{α - β}{2})}

= \frac{m n}{\frac{m^{2} + n^{2}}{2}}

∴ s i n (α + β) = \frac{2 m n}{m^{2} + n^{2}}

Commented by infinityaction last updated on 26/Apr/22

Answered by PhysicalMath last updated on 26/Apr/22

2 \sin (\frac{α + β}{2}) \cos (\frac{α - β}{2}) = n \dots e q 1

2 \cos (\frac{α + β}{2}) \cos (\frac{α - β}{2}) = m \dots e q 2

=> \frac{e q 1}{e q 2} = \tan (\frac{α + β}{2}) = \frac{n}{m}

\sin (α + β) = \frac{2 \tan (\frac{α + β}{2})}{1 + \tan^{2} (\frac{α + β}{2})}

= \frac{2 (\frac{n}{m})}{1 + {(\frac{n}{m})}^{2}} = \frac{2 m n}{m^{2} + n^{2}}

Commented by peter frank last updated on 29/Apr/22

very short