Question Number 169260 by mathlove last updated on 27/Apr/22

Answered by nurtani last updated on 27/Apr/22
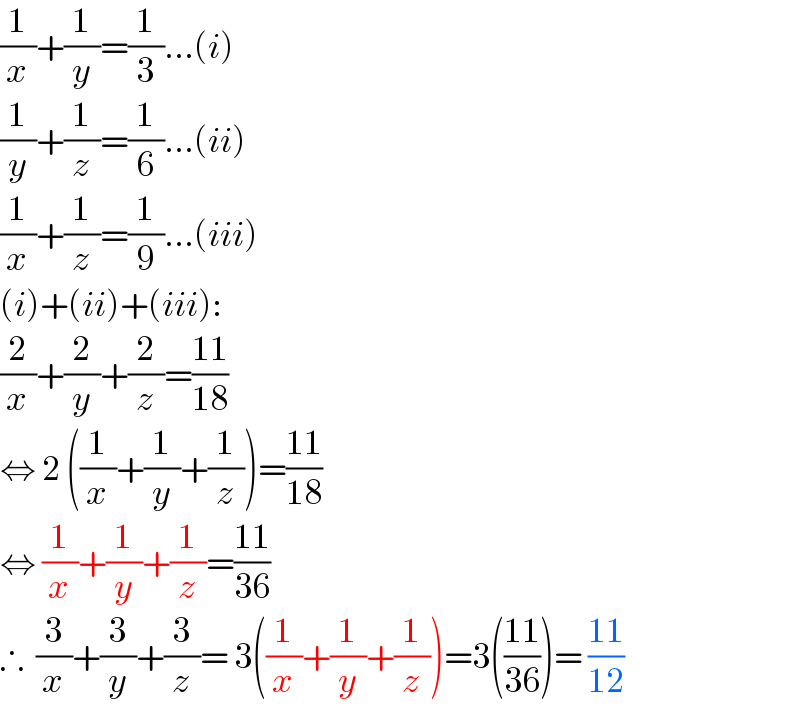
$$\frac{\mathrm{1}}{{x}}+\frac{\mathrm{1}}{{y}}=\frac{\mathrm{1}}{\mathrm{3}}…\left({i}\right) \\ $$$$\frac{\mathrm{1}}{{y}}+\frac{\mathrm{1}}{{z}}=\frac{\mathrm{1}}{\mathrm{6}}…\left({ii}\right) \\ $$$$\frac{\mathrm{1}}{{x}}+\frac{\mathrm{1}}{{z}}=\frac{\mathrm{1}}{\mathrm{9}}…\left({iii}\right) \\ $$$$\left({i}\right)+\left({ii}\right)+\left({iii}\right): \\ $$$$\frac{\mathrm{2}}{{x}}+\frac{\mathrm{2}}{{y}}+\frac{\mathrm{2}}{{z}}=\frac{\mathrm{11}}{\mathrm{18}} \\ $$$$\Leftrightarrow\:\mathrm{2}\:\left(\frac{\mathrm{1}}{{x}}+\frac{\mathrm{1}}{{y}}+\frac{\mathrm{1}}{{z}}\right)=\frac{\mathrm{11}}{\mathrm{18}} \\ $$$$\Leftrightarrow\:\frac{\mathrm{1}}{{x}}+\frac{\mathrm{1}}{{y}}+\frac{\mathrm{1}}{{z}}=\frac{\mathrm{11}}{\mathrm{36}} \\ $$$$\therefore\:\:\frac{\mathrm{3}}{{x}}+\frac{\mathrm{3}}{{y}}+\frac{\mathrm{3}}{{z}}=\:\mathrm{3}\left(\frac{\mathrm{1}}{{x}}+\frac{\mathrm{1}}{{y}}+\frac{\mathrm{1}}{{z}}\right)=\mathrm{3}\left(\frac{\mathrm{11}}{\mathrm{36}}\right)=\:\frac{\mathrm{11}}{\mathrm{12}} \\ $$
Commented by mathlove last updated on 27/Apr/22

$${thanks} \\ $$
