Question-169626

Question Number 169626 by Shrinava last updated on 04/May/22

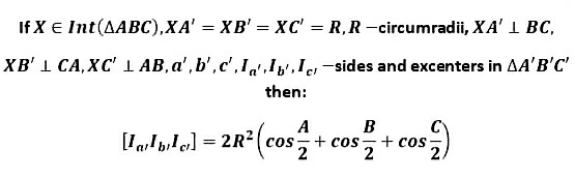
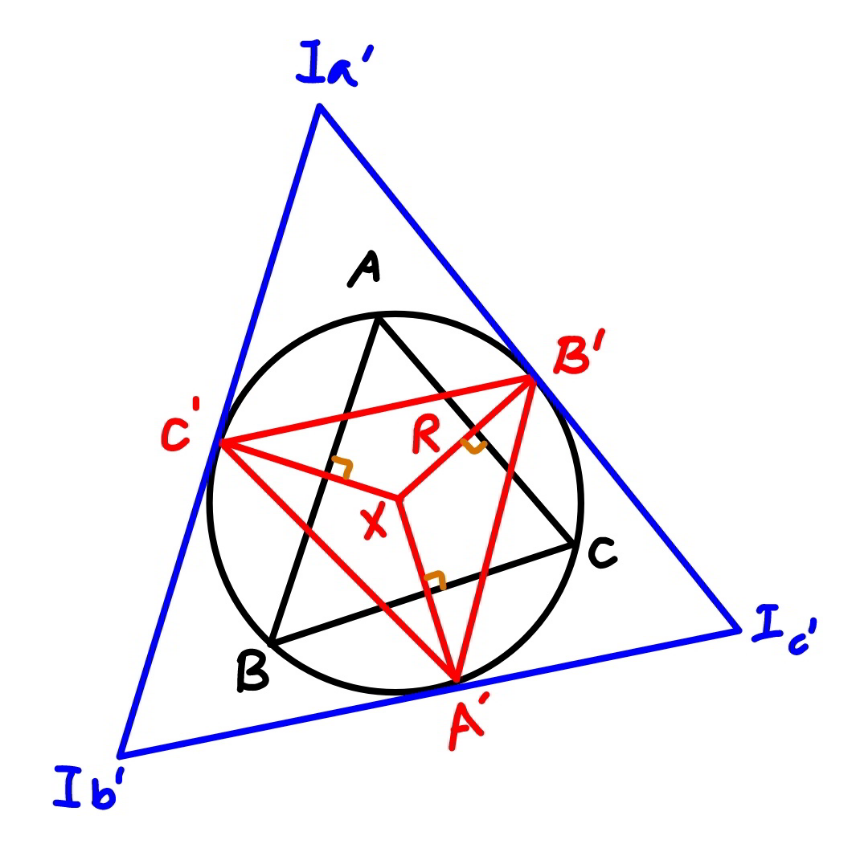
Answered by mr W last updated on 04/May/22

Commented by mr W last updated on 04/May/22

a^{'} = B^{'} C^{'} = 2 R \sin \frac{π - A}{2} = 2 R \sin A^{'}

\Rightarrow A^{'} = \frac{π}{2} - \frac{A}{2} \Rightarrow \sin A^{'} = \cos \frac{A}{2}

s i m i l a r l y

\Rightarrow B^{'} = \frac{π}{2} - \frac{B}{2} \Rightarrow \sin B^{'} = \cos \frac{B}{2}

\Rightarrow C^{'} = \frac{π}{2} - \frac{C}{2} \Rightarrow \sin C^{'} = \cos \frac{C}{2}

[I_{a^{'}} I_{b^{'}} I_{c^{'}}] = 8 R^{2} \cos \frac{A^{'}}{2} \cos \frac{B^{'}}{2} \cos \frac{C^{'}}{2}

[I_{a^{'}} I_{b^{'}} I_{c^{'}}] = 2 R^{2} (\sin A^{'} + \sin B^{'} + \sin C^{'})

[I_{a^{'}} I_{b^{'}} I_{c^{'}}] = 2 R^{2} (\cos \frac{A}{2} + \cos \frac{B}{2} + \cos \frac{C}{2}) ✓

Commented by MaxiMaths last updated on 05/May/22

please sir, which app do u use to make

those figures ?

Commented by mr W last updated on 05/May/22

n o t a s p e c i a l o n e . e a c h s m a r t p h o n e

h a s a n a p p f o r e d i t i n g i m a g e s .

Commented by Shrinava last updated on 05/May/22

Perfect - elegant solution

Thank you so much professor

Commented by Tawa11 last updated on 05/May/22

Great sir

Facebook Tweet Pin