Question-173302

Question Number 173302 by AgniMath last updated on 09/Jul/22

Commented by mr W last updated on 10/Jul/22

$${nice}\:{solution}! \\ $$

Commented by dragan91 last updated on 10/Jul/22

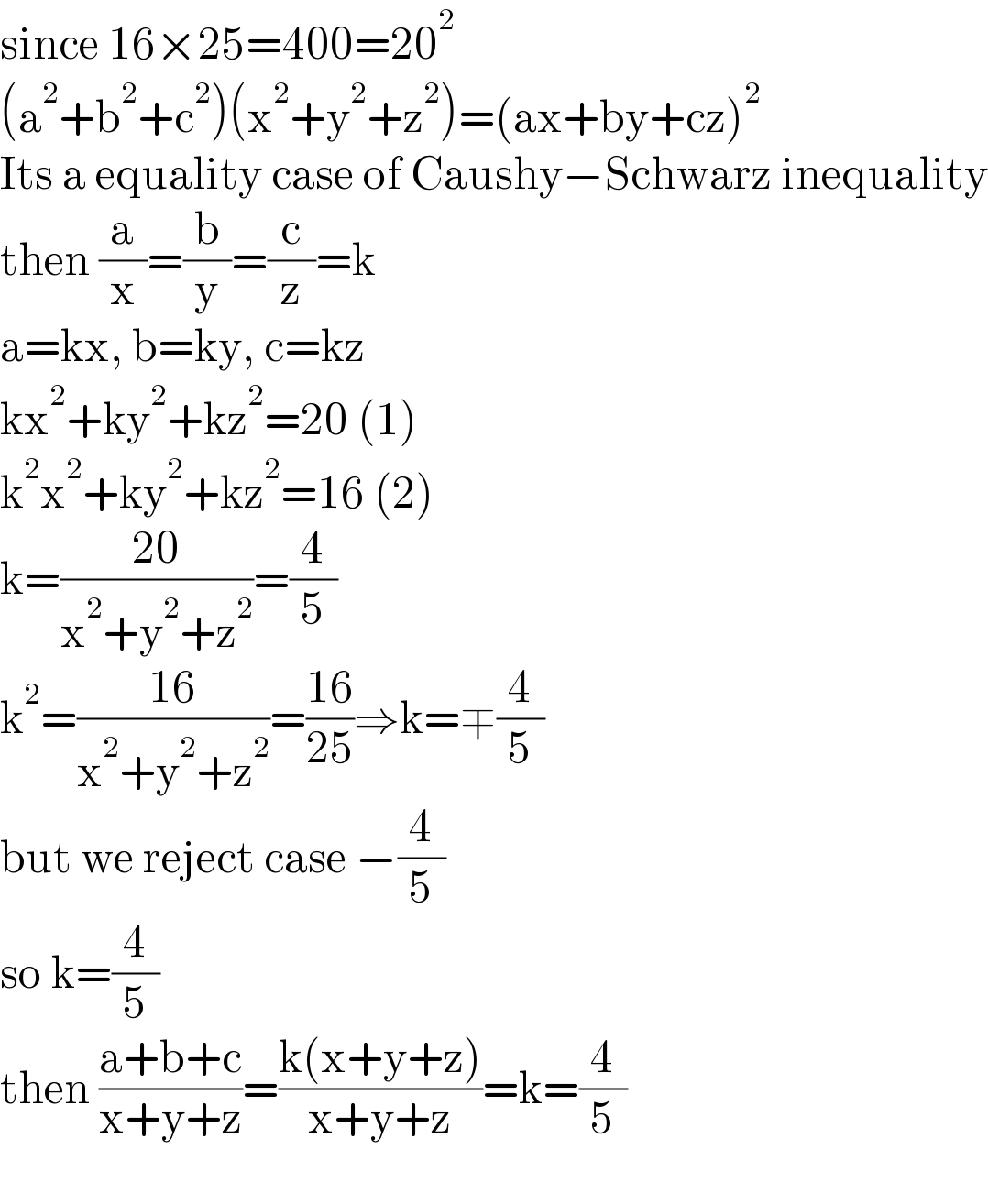
since 16×25=400=20^2 (a^2 +b^2 +c^2 )(x^2 +y^2 +z^2 )=(ax+by+cz)^2 Its a equality case of Caushy−Schwarz inequality then (a/x)=(b/y)=(c/z)=k a=kx, b=ky, c=kz kx^2 +ky^2 +kz^2 =20 (1) k^2 x^2 +ky^2 +kz^2 =16 (2) k=((20)/(x^2 +y^2 +z^2 ))=(4/5) k^2 =((16)/(x^2 +y^2 +z^2 ))=((16)/(25))⇒k=∓(4/5) but we reject case −(4/5) so k=(4/5) then ((a+b+c)/(x+y+z))=((k(x+y+z))/(x+y+z))=k=(4/5)

$$\mathrm{since}\:\mathrm{16}×\mathrm{25}=\mathrm{400}=\mathrm{20}^{\mathrm{2}} \\ $$$$\left(\mathrm{a}^{\mathrm{2}} +\mathrm{b}^{\mathrm{2}} +\mathrm{c}^{\mathrm{2}} \right)\left(\mathrm{x}^{\mathrm{2}} +\mathrm{y}^{\mathrm{2}} +\mathrm{z}^{\mathrm{2}} \right)=\left(\mathrm{ax}+\mathrm{by}+\mathrm{cz}\right)^{\mathrm{2}} \\ $$$$\mathrm{Its}\:\mathrm{a}\:\mathrm{equality}\:\mathrm{case}\:\mathrm{of}\:\mathrm{Caushy}−\mathrm{Schwarz}\:\mathrm{inequality} \\ $$$$\mathrm{then}\:\frac{\mathrm{a}}{\mathrm{x}}=\frac{\mathrm{b}}{\mathrm{y}}=\frac{\mathrm{c}}{\mathrm{z}}=\mathrm{k} \\ $$$$\mathrm{a}=\mathrm{kx},\:\mathrm{b}=\mathrm{ky},\:\mathrm{c}=\mathrm{kz} \\ $$$$\mathrm{kx}^{\mathrm{2}} +\mathrm{ky}^{\mathrm{2}} +\mathrm{kz}^{\mathrm{2}} =\mathrm{20}\:\left(\mathrm{1}\right) \\ $$$$\mathrm{k}^{\mathrm{2}} \mathrm{x}^{\mathrm{2}} +\mathrm{ky}^{\mathrm{2}} +\mathrm{kz}^{\mathrm{2}} =\mathrm{16}\:\left(\mathrm{2}\right) \\ $$$$\mathrm{k}=\frac{\mathrm{20}}{\mathrm{x}^{\mathrm{2}} +\mathrm{y}^{\mathrm{2}} +\mathrm{z}^{\mathrm{2}} }=\frac{\mathrm{4}}{\mathrm{5}} \\ $$$$\mathrm{k}^{\mathrm{2}} =\frac{\mathrm{16}}{\mathrm{x}^{\mathrm{2}} +\mathrm{y}^{\mathrm{2}} +\mathrm{z}^{\mathrm{2}} }=\frac{\mathrm{16}}{\mathrm{25}}\Rightarrow\mathrm{k}=\mp\frac{\mathrm{4}}{\mathrm{5}} \\ $$$$\mathrm{but}\:\mathrm{we}\:\mathrm{reject}\:\mathrm{case}\:−\frac{\mathrm{4}}{\mathrm{5}} \\ $$$$\mathrm{so}\:\mathrm{k}=\frac{\mathrm{4}}{\mathrm{5}} \\ $$$$\mathrm{then}\:\frac{\mathrm{a}+\mathrm{b}+\mathrm{c}}{\mathrm{x}+\mathrm{y}+\mathrm{z}}=\frac{\mathrm{k}\left(\mathrm{x}+\mathrm{y}+\mathrm{z}\right)}{\mathrm{x}+\mathrm{y}+\mathrm{z}}=\mathrm{k}=\frac{\mathrm{4}}{\mathrm{5}} \\ $$$$ \\ $$

Answered by mr W last updated on 10/Jul/22

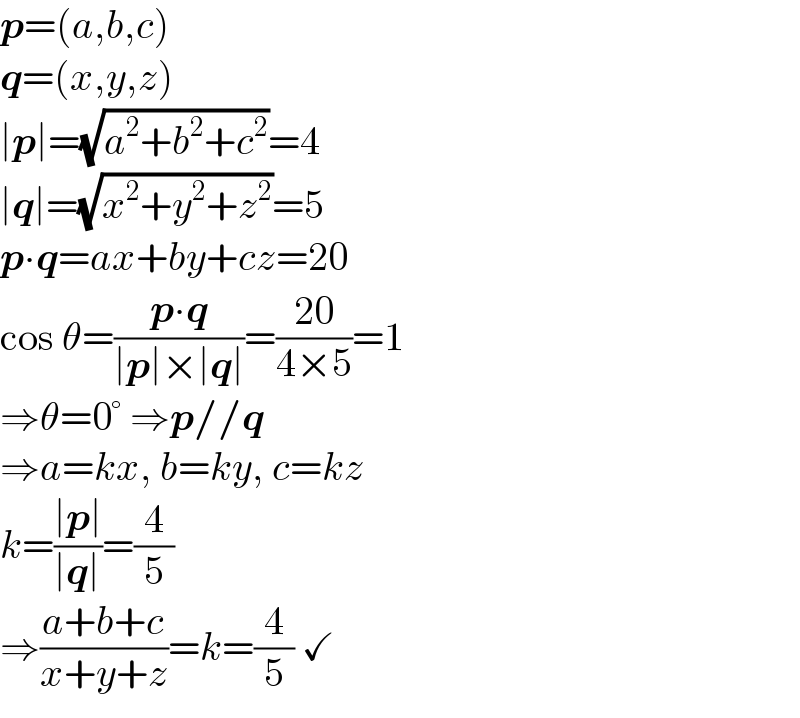
p=(a,b,c) q=(x,y,z) ∣p∣=(√(a^2 +b^2 +c^2 ))=4 ∣q∣=(√(x^2 +y^2 +z^2 ))=5 p∙q=ax+by+cz=20 cos θ=((p∙q)/(∣p∣×∣q∣))=((20)/(4×5))=1 ⇒θ=0° ⇒p//q ⇒a=kx, b=ky, c=kz k=((∣p∣)/(∣q∣))=(4/5) ⇒((a+b+c)/(x+y+z))=k=(4/5) ✓

$$\boldsymbol{{p}}=\left({a},{b},{c}\right) \\ $$$$\boldsymbol{{q}}=\left({x},{y},{z}\right) \\ $$$$\mid\boldsymbol{{p}}\mid=\sqrt{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} +{c}^{\mathrm{2}} }=\mathrm{4} \\ $$$$\mid\boldsymbol{{q}}\mid=\sqrt{{x}^{\mathrm{2}} +{y}^{\mathrm{2}} +{z}^{\mathrm{2}} }=\mathrm{5} \\ $$$$\boldsymbol{{p}}\centerdot\boldsymbol{{q}}={ax}+{by}+{cz}=\mathrm{20}\: \\ $$$$\mathrm{cos}\:\theta=\frac{\boldsymbol{{p}}\centerdot\boldsymbol{{q}}}{\mid\boldsymbol{{p}}\mid×\mid\boldsymbol{{q}}\mid}=\frac{\mathrm{20}}{\mathrm{4}×\mathrm{5}}=\mathrm{1}\: \\ $$$$\Rightarrow\theta=\mathrm{0}°\:\Rightarrow\boldsymbol{{p}}//\boldsymbol{{q}} \\ $$$$\Rightarrow{a}={kx},\:{b}={ky},\:{c}={kz} \\ $$$${k}=\frac{\mid\boldsymbol{{p}}\mid}{\mid\boldsymbol{{q}}\mid}=\frac{\mathrm{4}}{\mathrm{5}} \\ $$$$\Rightarrow\frac{{a}+{b}+{c}}{{x}+{y}+{z}}={k}=\frac{\mathrm{4}}{\mathrm{5}}\:\checkmark \\ $$

Answered by AgniMath last updated on 09/Jul/22

Another way Let b = c = 0 and y = z = 0 a^2 + b^2 + c^2 = 16 or a^2 = 16 ∴ a = 4 or −4 x^2 + y^2 + z^2 = 25 or x^2 = 25 ∴ x = 5 or −5 ax + by + cz = 20 ∴ ax = 20 [ b = c = y = z = 0] so ax can be 4 × 5 or −4 × −5 ((a + b + c)/(x + y + z)) = (4/5) or ((−4)/(−5)) = (4/5) (Ans)

$$\mathrm{Another}\:\mathrm{way} \\ $$$$ \\ $$$$\mathrm{Let}\:\mathrm{b}\:=\:\mathrm{c}\:=\:\mathrm{0}\:\mathrm{and}\:\mathrm{y}\:=\:\mathrm{z}\:=\:\mathrm{0} \\ $$$${a}^{\mathrm{2}} \:+\:{b}^{\mathrm{2}} \:+\:{c}^{\mathrm{2}} \:=\:\mathrm{16} \\ $$$${or}\:{a}^{\mathrm{2}} \:=\:\mathrm{16}\: \\ $$$$\therefore\:{a}\:=\:\mathrm{4}\:{or}\:−\mathrm{4} \\ $$$${x}^{\mathrm{2}} \:+\:{y}^{\mathrm{2}} \:+\:{z}^{\mathrm{2}} \:=\:\mathrm{25} \\ $$$${or}\:{x}^{\mathrm{2}} \:=\:\mathrm{25} \\ $$$$\therefore\:{x}\:=\:\mathrm{5}\:{or}\:−\mathrm{5} \\ $$$${ax}\:+\:{by}\:+\:{cz}\:=\:\mathrm{20} \\ $$$$\therefore\:{ax}\:=\:\mathrm{20}\:\left[\:{b}\:=\:{c}\:=\:{y}\:=\:{z}\:=\:\mathrm{0}\right] \\ $$$${so}\:{ax}\:{can}\:{be}\:\mathrm{4}\:×\:\mathrm{5}\:{or}\:−\mathrm{4}\:×\:−\mathrm{5} \\ $$$$ \\ $$$$\frac{{a}\:+\:{b}\:+\:{c}}{{x}\:+\:{y}\:+\:{z}}\:=\:\frac{\mathrm{4}}{\mathrm{5}}\:{or}\:\frac{\cancel{−}\mathrm{4}}{\cancel{−}\mathrm{5}}\:=\:\frac{\mathrm{4}}{\mathrm{5}}\:\left(\mathrm{Ans}\right) \\ $$

Commented by mr W last updated on 09/Jul/22

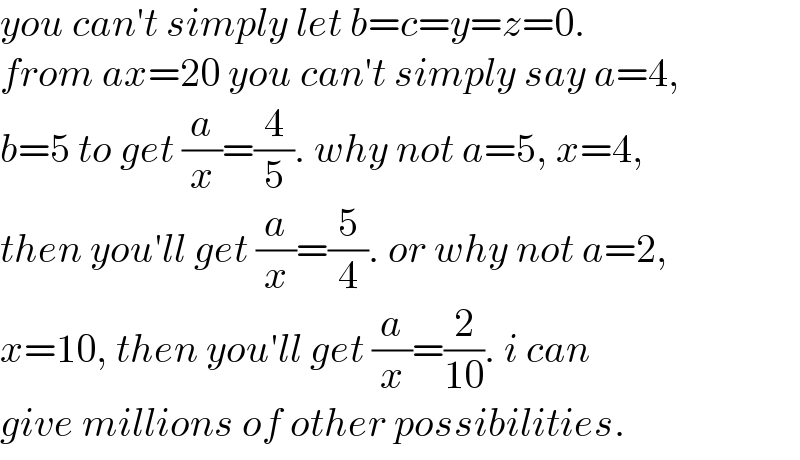
you can′t simply let b=c=y=z=0. from ax=20 you can′t simply say a=4, b=5 to get (a/x)=(4/5). why not a=5, x=4, then you′ll get (a/x)=(5/4). or why not a=2, x=10, then you′ll get (a/x)=(2/(10)). i can give millions of other possibilities.

$${you}\:{can}'{t}\:{simply}\:{let}\:{b}={c}={y}={z}=\mathrm{0}. \\ $$$${from}\:{ax}=\mathrm{20}\:{you}\:{can}'{t}\:{simply}\:{say}\:{a}=\mathrm{4}, \\ $$$${b}=\mathrm{5}\:{to}\:{get}\:\frac{{a}}{{x}}=\frac{\mathrm{4}}{\mathrm{5}}.\:{why}\:{not}\:{a}=\mathrm{5},\:{x}=\mathrm{4}, \\ $$$${then}\:{you}'{ll}\:{get}\:\frac{{a}}{{x}}=\frac{\mathrm{5}}{\mathrm{4}}.\:{or}\:{why}\:{not}\:{a}=\mathrm{2}, \\ $$$${x}=\mathrm{10},\:{then}\:{you}'{ll}\:{get}\:\frac{{a}}{{x}}=\frac{\mathrm{2}}{\mathrm{10}}.\:{i}\:{can} \\ $$$${give}\:{millions}\:{of}\:{other}\:{possibilities}. \\ $$

Commented by AgniMath last updated on 10/Jul/22