Question Number 173523 by Physicien last updated on 13/Jul/22

Answered by Mathspace last updated on 13/Jul/22
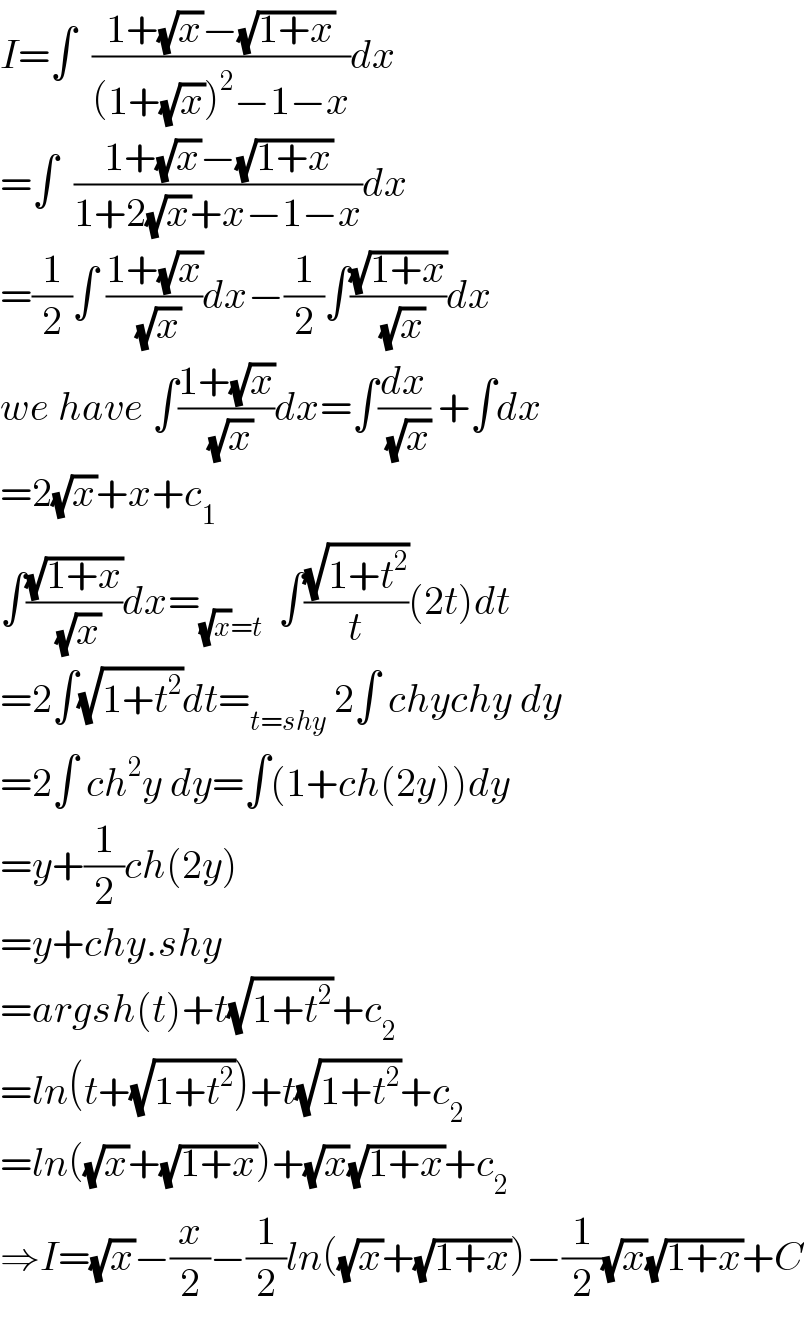
$${I}=\int\:\:\frac{\mathrm{1}+\sqrt{{x}}−\sqrt{\mathrm{1}+{x}}}{\left(\mathrm{1}+\sqrt{{x}}\right)^{\mathrm{2}} −\mathrm{1}−{x}}{dx} \\ $$$$=\int\:\:\frac{\mathrm{1}+\sqrt{{x}}−\sqrt{\mathrm{1}+{x}}}{\mathrm{1}+\mathrm{2}\sqrt{{x}}+{x}−\mathrm{1}−{x}}{dx} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\int\:\frac{\mathrm{1}+\sqrt{{x}}}{\:\sqrt{{x}}}{dx}−\frac{\mathrm{1}}{\mathrm{2}}\int\frac{\sqrt{\mathrm{1}+{x}}}{\:\sqrt{{x}}}{dx} \\ $$$${we}\:{have}\:\int\frac{\mathrm{1}+\sqrt{{x}}}{\:\sqrt{{x}}}{dx}=\int\frac{{dx}}{\:\sqrt{{x}}}\:+\int{dx} \\ $$$$=\mathrm{2}\sqrt{{x}}+{x}+{c}_{\mathrm{1}} \\ $$$$\int\frac{\sqrt{\mathrm{1}+{x}}}{\:\sqrt{{x}}}{dx}=_{\sqrt{{x}}={t}} \:\:\int\frac{\sqrt{\mathrm{1}+{t}^{\mathrm{2}} }}{{t}}\left(\mathrm{2}{t}\right){dt} \\ $$$$=\mathrm{2}\int\sqrt{\mathrm{1}+{t}^{\mathrm{2}} }{dt}=_{{t}={shy}} \:\mathrm{2}\int\:{chychy}\:{dy} \\ $$$$=\mathrm{2}\int\:{ch}^{\mathrm{2}} {y}\:{dy}=\int\left(\mathrm{1}+{ch}\left(\mathrm{2}{y}\right)\right){dy} \\ $$$$={y}+\frac{\mathrm{1}}{\mathrm{2}}{ch}\left(\mathrm{2}{y}\right) \\ $$$$={y}+{chy}.{shy} \\ $$$$={argsh}\left({t}\right)+{t}\sqrt{\mathrm{1}+{t}^{\mathrm{2}} }+{c}_{\mathrm{2}} \\ $$$$={ln}\left({t}+\sqrt{\mathrm{1}+{t}^{\mathrm{2}} }\right)+{t}\sqrt{\mathrm{1}+{t}^{\mathrm{2}} }+{c}_{\mathrm{2}} \\ $$$$={ln}\left(\sqrt{{x}}+\sqrt{\mathrm{1}+{x}}\right)+\sqrt{{x}}\sqrt{\mathrm{1}+{x}}+{c}_{\mathrm{2}} \\ $$$$\Rightarrow{I}=\sqrt{{x}}−\frac{{x}}{\mathrm{2}}−\frac{\mathrm{1}}{\mathrm{2}}{ln}\left(\sqrt{{x}}+\sqrt{\mathrm{1}+{x}}\right)−\frac{\mathrm{1}}{\mathrm{2}}\sqrt{{x}}\sqrt{\mathrm{1}+{x}}+{C} \\ $$
Commented by Tawa11 last updated on 13/Jul/22

$$\mathrm{Great}\:\mathrm{sir}. \\ $$
Commented by Mathspace last updated on 13/Jul/22

$${you}\:{arewelcome} \\ $$
Answered by MJS_new last updated on 13/Jul/22
![∫(dx/(1+(√x)+(√(1+x))))= [t=(√x)+(√(x+1)) → dx=((2(√(x+1))(√x))/t)] =(1/2)∫(((t−1)(t^2 +1))/t^3 )dt= =(1/2)∫(1−(1/t)+(1/t^2 )−(1/t^3 ))dt= ... =((x+2(√x)−(√(x+1))(√x)−ln ((√(x+1))+(√x)))/2)+C](https://www.tinkutara.com/question/Q173570.png)
$$\int\frac{{dx}}{\mathrm{1}+\sqrt{{x}}+\sqrt{\mathrm{1}+{x}}}= \\ $$$$\:\:\:\:\:\left[{t}=\sqrt{{x}}+\sqrt{{x}+\mathrm{1}}\:\rightarrow\:{dx}=\frac{\mathrm{2}\sqrt{{x}+\mathrm{1}}\sqrt{{x}}}{{t}}\right] \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\int\frac{\left({t}−\mathrm{1}\right)\left({t}^{\mathrm{2}} +\mathrm{1}\right)}{{t}^{\mathrm{3}} }{dt}= \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\int\left(\mathrm{1}−\frac{\mathrm{1}}{{t}}+\frac{\mathrm{1}}{{t}^{\mathrm{2}} }−\frac{\mathrm{1}}{{t}^{\mathrm{3}} }\right){dt}= \\ $$$$… \\ $$$$=\frac{{x}+\mathrm{2}\sqrt{{x}}−\sqrt{{x}+\mathrm{1}}\sqrt{{x}}−\mathrm{ln}\:\left(\sqrt{{x}+\mathrm{1}}+\sqrt{{x}}\right)}{\mathrm{2}}+{C} \\ $$
