Question Number 175430 by mathlove last updated on 30/Aug/22
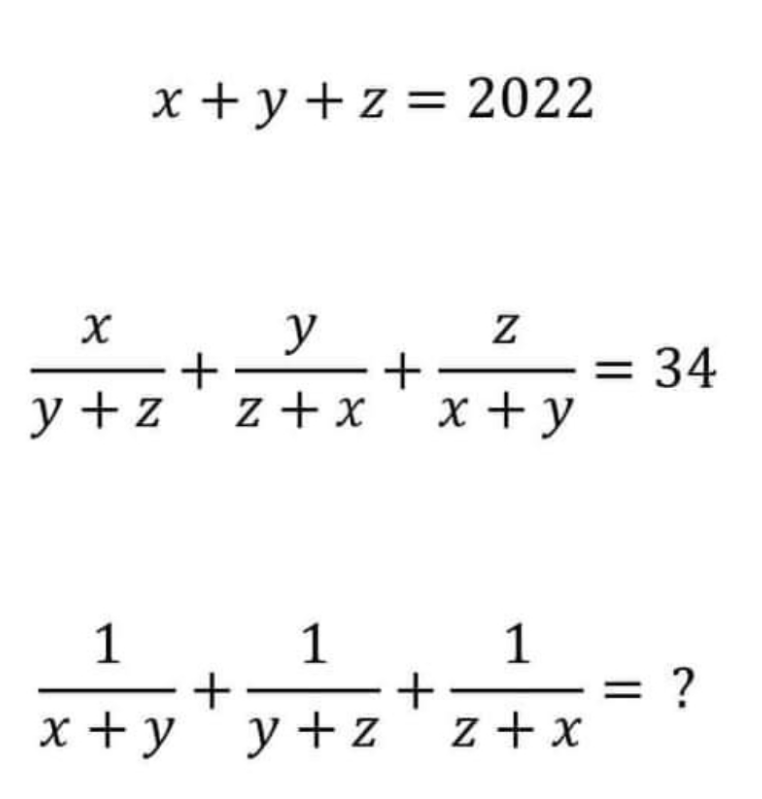
Commented by infinityaction last updated on 30/Aug/22
![(x/(y+z))+1+(y/(z+x))+1+(z/(x+y))+1 =37 x+y+z[(1/(y+z))+(1/(z+x))+(1/(x+y))] = 37 (1/(y+z))+(1/(z+x))+(1/(x+y)) = ((37)/(2022))](https://www.tinkutara.com/question/Q175431.png)
$$\frac{{x}}{{y}+{z}}+\mathrm{1}+\frac{{y}}{{z}+{x}}+\mathrm{1}+\frac{{z}}{{x}+{y}}+\mathrm{1}\:=\mathrm{37} \\ $$$${x}+{y}+{z}\left[\frac{\mathrm{1}}{{y}+{z}}+\frac{\mathrm{1}}{{z}+{x}}+\frac{\mathrm{1}}{{x}+{y}}\right]\:=\:\mathrm{37} \\ $$$$\frac{\mathrm{1}}{{y}+{z}}+\frac{\mathrm{1}}{{z}+{x}}+\frac{\mathrm{1}}{{x}+{y}}\:=\:\frac{\mathrm{37}}{\mathrm{2022}} \\ $$
Commented by mathlove last updated on 30/Aug/22

$${thanks} \\ $$
