Question Number 175588 by alcohol last updated on 03/Sep/22
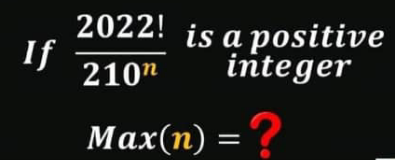
Commented by mahdipoor last updated on 04/Oct/22
![210=2×3×5×7 in 2022!=2^a ×3^b ×5^c ×7^d ×... a≥b≥c≥d≥... ⇒ max(n) if ((2022!)/(210^n ))∈N = max(d) if ((2022!)/7^d )∈N d=[((2022)/7)]+[((2022)/7^2 )]+[((2022)/7^3 )]+[((2022)/7^4 )]+...= 288+41+5+0+...=334](https://www.tinkutara.com/question/Q175590.png)
$$\mathrm{210}=\mathrm{2}×\mathrm{3}×\mathrm{5}×\mathrm{7} \\ $$$${in}\:\mathrm{2022}!=\mathrm{2}^{{a}} ×\mathrm{3}^{{b}} ×\mathrm{5}^{{c}} ×\mathrm{7}^{{d}} ×… \\ $$$${a}\geqslant{b}\geqslant{c}\geqslant{d}\geqslant…\:\Rightarrow \\ $$$${max}\left({n}\right)\:{if}\:\frac{\mathrm{2022}!}{\mathrm{210}^{{n}} }\in{N}\:=\:{max}\left({d}\right)\:{if}\:\frac{\mathrm{2022}!}{\mathrm{7}^{{d}} }\in{N} \\ $$$${d}=\left[\frac{\mathrm{2022}}{\mathrm{7}}\right]+\left[\frac{\mathrm{2022}}{\mathrm{7}^{\mathrm{2}} }\right]+\left[\frac{\mathrm{2022}}{\mathrm{7}^{\mathrm{3}} }\right]+\left[\frac{\mathrm{2022}}{\mathrm{7}^{\mathrm{4}} }\right]+…= \\ $$$$\mathrm{288}+\mathrm{41}+\mathrm{5}+\mathrm{0}+…=\mathrm{334} \\ $$
Answered by BaliramKumar last updated on 13/Mar/23
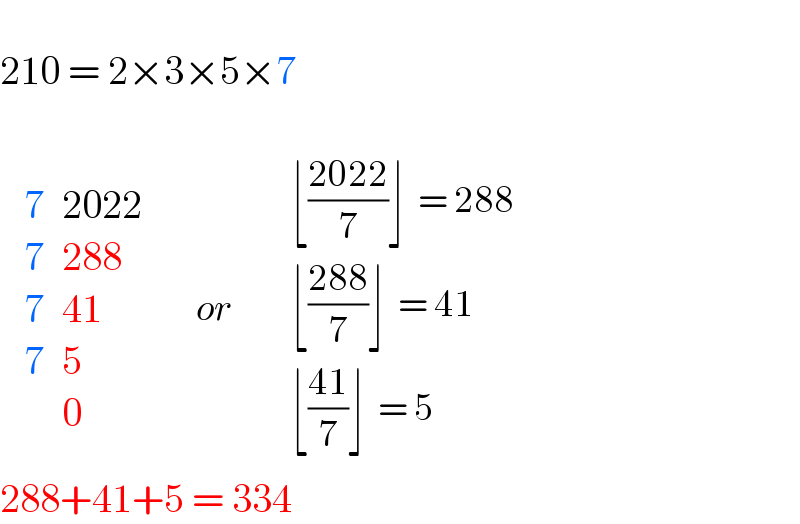
$$ \\ $$$$\mathrm{210}\:=\:\mathrm{2}×\mathrm{3}×\mathrm{5}×\mathrm{7} \\ $$$$ \\ $$$$\:\begin{array}{|c|c|c|c|c|}{\mathrm{7}}&\hline{\mathrm{2022}}\\{\mathrm{7}}&\hline{\mathrm{288}}\\{\mathrm{7}}&\hline{\mathrm{41}}\\{\mathrm{7}}&\hline{\mathrm{5}}\\{}&\hline{\mathrm{0}}\\\hline\end{array}\:\:\:\:\:\:{or}\:\:\:\:\:\:\:\begin{array}{|c|c|c|}{\lfloor\frac{\mathrm{2022}}{\mathrm{7}}\rfloor\:\:=\:\mathrm{288}}\\{\lfloor\frac{\mathrm{288}}{\mathrm{7}}\rfloor\:\:=\:\mathrm{41}}\\{\lfloor\frac{\mathrm{41}}{\mathrm{7}}\rfloor\:\:=\:\mathrm{5}}\\\hline\end{array} \\ $$$$\mathrm{288}+\mathrm{41}+\mathrm{5}\:=\:\mathrm{334} \\ $$
Commented by Tawa11 last updated on 15/Sep/22

$$\mathrm{Great}\:\mathrm{sir} \\ $$
Answered by mr W last updated on 03/Sep/22

$$\frac{\mathrm{2022}!}{\mathrm{210}^{{n}} }={k} \\ $$$$\mathrm{2022}!=\mathrm{210}^{{n}} {k} \\ $$$$\mathrm{2022}!=\mathrm{2}^{{n}} \mathrm{3}^{{n}} \mathrm{5}^{{n}} \mathrm{7}^{{n}} {k} \\ $$$$\mathrm{2022}!=\mathrm{2}^{\mathrm{2014}} ×\mathrm{3}^{\mathrm{1006}} ×\mathrm{5}^{\mathrm{503}} ×\mathrm{7}^{\mathrm{334}} ×… \\ $$$$\Rightarrow{n}_{{max}} =\mathrm{334} \\ $$$${see}\:{also}\:{Q}\mathrm{115294} \\ $$
Commented by Tawa11 last updated on 15/Sep/22

$$\mathrm{Great}\:\mathrm{sir} \\ $$
