Question-175740

Question Number 175740 by daus last updated on 06/Sep/22

Answered by Ar Brandon last updated on 06/Sep/22

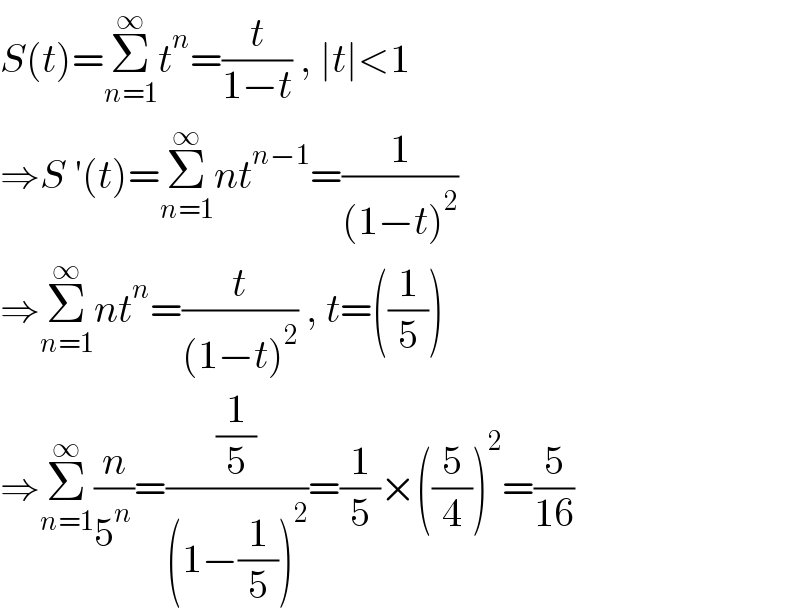
S(t)=Σ_(n=1) ^∞ t^n =(t/(1−t)) , ∣t∣<1 ⇒S ′(t)=Σ_(n=1) ^∞ nt^(n−1) =(1/((1−t)^2 )) ⇒Σ_(n=1) ^∞ nt^n =(t/((1−t)^2 )) , t=((1/5)) ⇒Σ_(n=1) ^∞ (n/5^n )=((1/5)/((1−(1/5))^2 ))=(1/5)×((5/4))^2 =(5/(16))

$${S}\left({t}\right)=\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}{t}^{{n}} =\frac{{t}}{\mathrm{1}−{t}}\:,\:\mid{t}\mid<\mathrm{1} \\ $$$$\Rightarrow{S}\:'\left({t}\right)=\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}{nt}^{{n}−\mathrm{1}} =\frac{\mathrm{1}}{\left(\mathrm{1}−{t}\right)^{\mathrm{2}} } \\ $$$$\Rightarrow\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}{nt}^{{n}} =\frac{{t}}{\left(\mathrm{1}−{t}\right)^{\mathrm{2}} }\:,\:{t}=\left(\frac{\mathrm{1}}{\mathrm{5}}\right) \\ $$$$\Rightarrow\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{{n}}{\mathrm{5}^{{n}} }=\frac{\frac{\mathrm{1}}{\mathrm{5}}}{\left(\mathrm{1}−\frac{\mathrm{1}}{\mathrm{5}}\right)^{\mathrm{2}} }=\frac{\mathrm{1}}{\mathrm{5}}×\left(\frac{\mathrm{5}}{\mathrm{4}}\right)^{\mathrm{2}} =\frac{\mathrm{5}}{\mathrm{16}} \\ $$

Answered by Ar Brandon last updated on 06/Sep/22

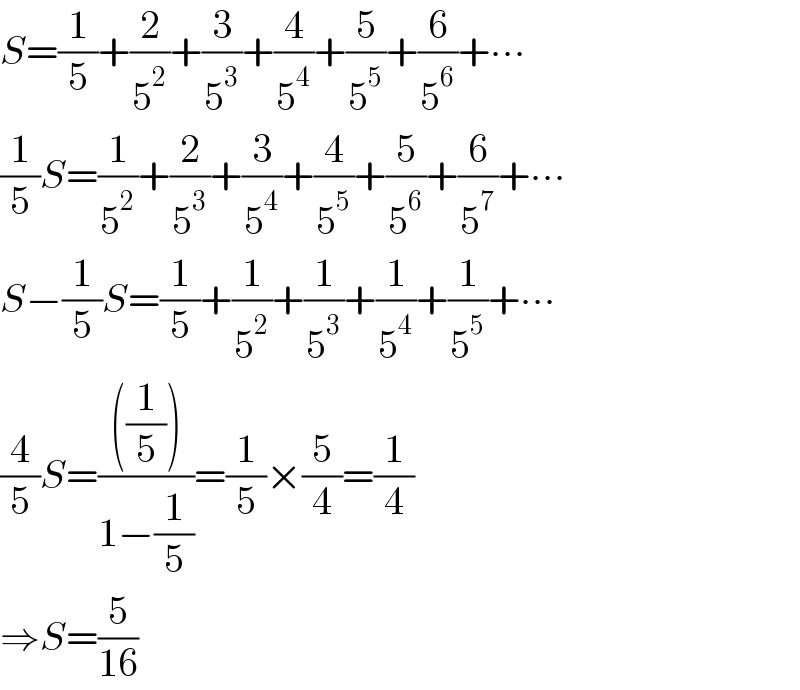
S=(1/5)+(2/5^2 )+(3/5^3 )+(4/5^4 )+(5/5^5 )+(6/5^6 )+∙∙∙ (1/5)S=(1/5^2 )+(2/5^3 )+(3/5^4 )+(4/5^5 )+(5/5^6 )+(6/5^7 )+∙∙∙ S−(1/5)S=(1/5)+(1/5^2 )+(1/5^3 )+(1/5^4 )+(1/5^5 )+∙∙∙ (4/5)S=((((1/5)))/(1−(1/5)))=(1/5)×(5/4)=(1/4) ⇒S=(5/(16))

$${S}=\frac{\mathrm{1}}{\mathrm{5}}+\frac{\mathrm{2}}{\mathrm{5}^{\mathrm{2}} }+\frac{\mathrm{3}}{\mathrm{5}^{\mathrm{3}} }+\frac{\mathrm{4}}{\mathrm{5}^{\mathrm{4}} }+\frac{\mathrm{5}}{\mathrm{5}^{\mathrm{5}} }+\frac{\mathrm{6}}{\mathrm{5}^{\mathrm{6}} }+\centerdot\centerdot\centerdot \\ $$$$\frac{\mathrm{1}}{\mathrm{5}}{S}=\frac{\mathrm{1}}{\mathrm{5}^{\mathrm{2}} }+\frac{\mathrm{2}}{\mathrm{5}^{\mathrm{3}} }+\frac{\mathrm{3}}{\mathrm{5}^{\mathrm{4}} }+\frac{\mathrm{4}}{\mathrm{5}^{\mathrm{5}} }+\frac{\mathrm{5}}{\mathrm{5}^{\mathrm{6}} }+\frac{\mathrm{6}}{\mathrm{5}^{\mathrm{7}} }+\centerdot\centerdot\centerdot \\ $$$${S}−\frac{\mathrm{1}}{\mathrm{5}}{S}=\frac{\mathrm{1}}{\mathrm{5}}+\frac{\mathrm{1}}{\mathrm{5}^{\mathrm{2}} }+\frac{\mathrm{1}}{\mathrm{5}^{\mathrm{3}} }+\frac{\mathrm{1}}{\mathrm{5}^{\mathrm{4}} }+\frac{\mathrm{1}}{\mathrm{5}^{\mathrm{5}} }+\centerdot\centerdot\centerdot \\ $$$$\frac{\mathrm{4}}{\mathrm{5}}{S}=\frac{\left(\frac{\mathrm{1}}{\mathrm{5}}\right)}{\mathrm{1}−\frac{\mathrm{1}}{\mathrm{5}}}=\frac{\mathrm{1}}{\mathrm{5}}×\frac{\mathrm{5}}{\mathrm{4}}=\frac{\mathrm{1}}{\mathrm{4}} \\ $$$$\Rightarrow{S}=\frac{\mathrm{5}}{\mathrm{16}} \\ $$

Commented by daus last updated on 07/Sep/22

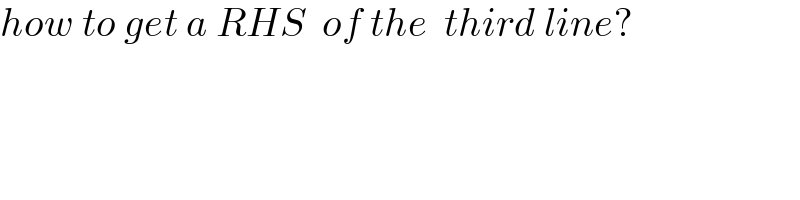
$${how}\:{to}\:{get}\:{a}\:{RHS}\:\:{of}\:{the}\:\:{third}\:{line}? \\ $$

Commented by Ar Brandon last updated on 07/Sep/22

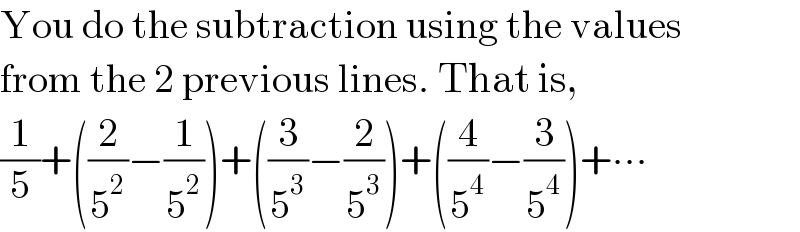
You do the subtraction using the values from the 2 previous lines. That is, (1/5)+((2/5^2 )−(1/5^2 ))+((3/5^3 )−(2/5^3 ))+((4/5^4 )−(3/5^4 ))+∙∙∙

$$\mathrm{You}\:\mathrm{do}\:\mathrm{the}\:\mathrm{subtraction}\:\mathrm{using}\:\mathrm{the}\:\mathrm{values} \\ $$$$\mathrm{from}\:\mathrm{the}\:\mathrm{2}\:\mathrm{previous}\:\mathrm{lines}.\:\mathrm{That}\:\mathrm{is}, \\ $$$$\frac{\mathrm{1}}{\mathrm{5}}+\left(\frac{\mathrm{2}}{\mathrm{5}^{\mathrm{2}} }−\frac{\mathrm{1}}{\mathrm{5}^{\mathrm{2}} }\right)+\left(\frac{\mathrm{3}}{\mathrm{5}^{\mathrm{3}} }−\frac{\mathrm{2}}{\mathrm{5}^{\mathrm{3}} }\right)+\left(\frac{\mathrm{4}}{\mathrm{5}^{\mathrm{4}} }−\frac{\mathrm{3}}{\mathrm{5}^{\mathrm{4}} }\right)+\centerdot\centerdot\centerdot \\ $$

Commented by peter frank last updated on 06/Sep/22

$$\mathrm{thanks} \\ $$

Commented by Tawa11 last updated on 15/Sep/22

$$\mathrm{Great}\:\mathrm{sir} \\ $$

Answered by Rasheed.Sindhi last updated on 06/Sep/22

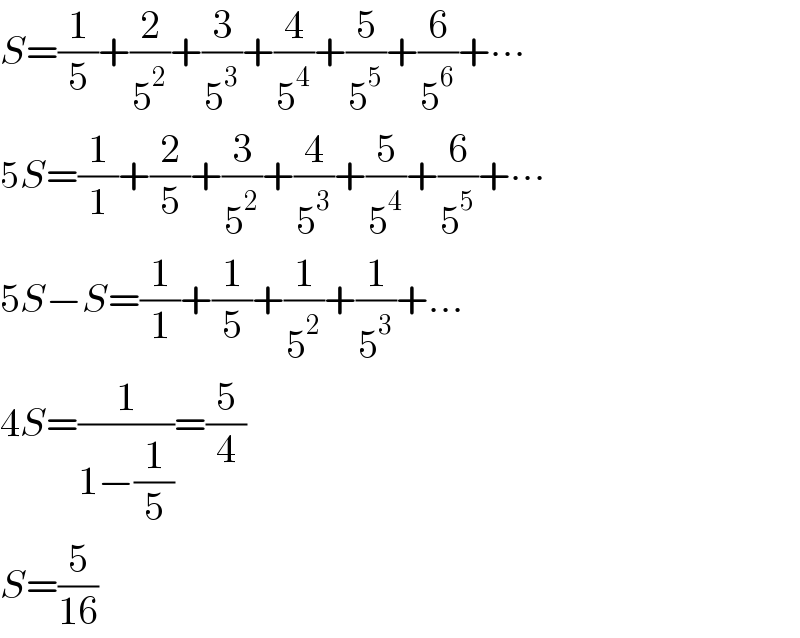
S=(1/5)+(2/5^2 )+(3/5^3 )+(4/5^4 )+(5/5^5 )+(6/5^6 )+∙∙∙ 5S=(1/1)+(2/5)+(3/5^2 )+(4/5^3 )+(5/5^4 )+(6/5^5 )+∙∙∙ 5S−S=(1/1)+(1/5)+(1/5^2 )+(1/5^3 )+... 4S=(1/(1−(1/5)))=(5/4) S=(5/(16))

$${S}=\frac{\mathrm{1}}{\mathrm{5}}+\frac{\mathrm{2}}{\mathrm{5}^{\mathrm{2}} }+\frac{\mathrm{3}}{\mathrm{5}^{\mathrm{3}} }+\frac{\mathrm{4}}{\mathrm{5}^{\mathrm{4}} }+\frac{\mathrm{5}}{\mathrm{5}^{\mathrm{5}} }+\frac{\mathrm{6}}{\mathrm{5}^{\mathrm{6}} }+\centerdot\centerdot\centerdot \\ $$$$\mathrm{5}{S}=\frac{\mathrm{1}}{\mathrm{1}}+\frac{\mathrm{2}}{\mathrm{5}}+\frac{\mathrm{3}}{\mathrm{5}^{\mathrm{2}} }+\frac{\mathrm{4}}{\mathrm{5}^{\mathrm{3}} }+\frac{\mathrm{5}}{\mathrm{5}^{\mathrm{4}} }+\frac{\mathrm{6}}{\mathrm{5}^{\mathrm{5}} }+\centerdot\centerdot\centerdot \\ $$$$\mathrm{5}{S}−{S}=\frac{\mathrm{1}}{\mathrm{1}}+\frac{\mathrm{1}}{\mathrm{5}}+\frac{\mathrm{1}}{\mathrm{5}^{\mathrm{2}} }+\frac{\mathrm{1}}{\mathrm{5}^{\mathrm{3}} }+… \\ $$$$\mathrm{4}{S}=\frac{\mathrm{1}}{\mathrm{1}−\frac{\mathrm{1}}{\mathrm{5}}}=\frac{\mathrm{5}}{\mathrm{4}} \\ $$$${S}=\frac{\mathrm{5}}{\mathrm{16}} \\ $$

Commented by peter frank last updated on 06/Sep/22

$$\mathrm{thanks} \\ $$

Facebook Tweet Pin

Leave a Reply Cancel reply