Question Number 176318 by Matica last updated on 16/Sep/22
Commented by Rasheed.Sindhi last updated on 16/Sep/22

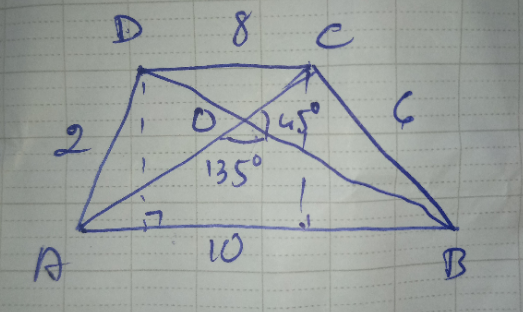
$${find}\:{area}\:{of}\:{quadrilateral}\:\mathrm{ABCD} \\ $$
Commented by mr W last updated on 16/Sep/22
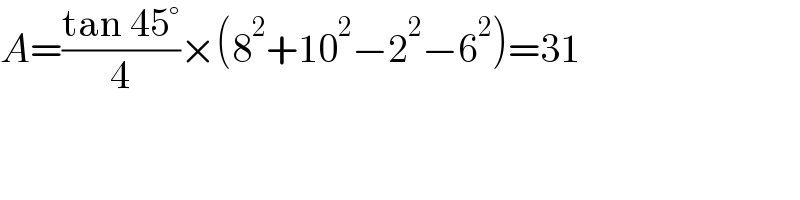
$${A}=\frac{\mathrm{tan}\:\mathrm{45}°}{\mathrm{4}}×\left(\mathrm{8}^{\mathrm{2}} +\mathrm{10}^{\mathrm{2}} −\mathrm{2}^{\mathrm{2}} −\mathrm{6}^{\mathrm{2}} \right)=\mathrm{31} \\ $$
Commented by Matica last updated on 16/Sep/22

$${please}\:{say}\:{more}\:{detail} \\ $$
Answered by mr W last updated on 16/Sep/22
Commented by mr W last updated on 16/Sep/22
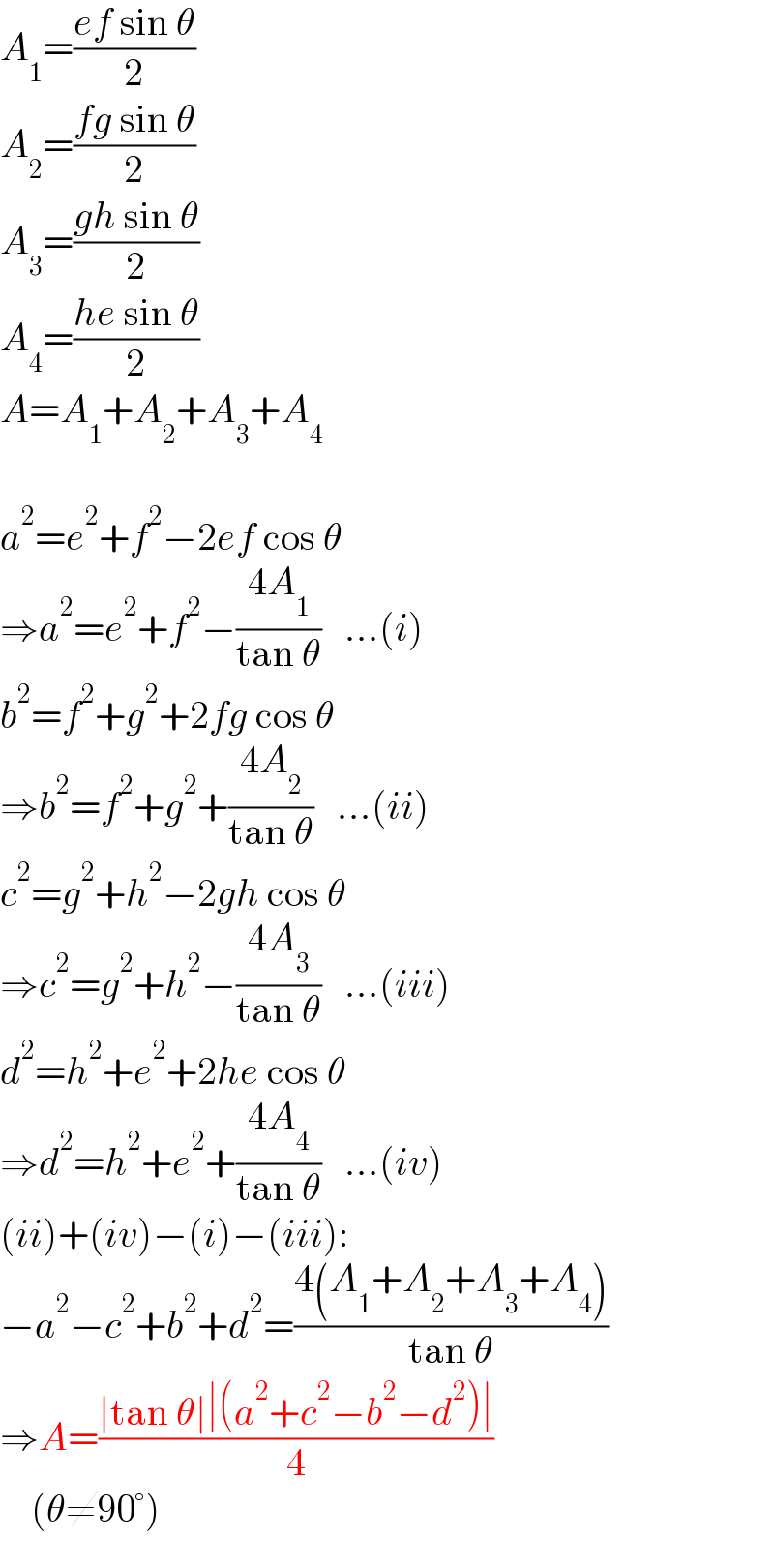
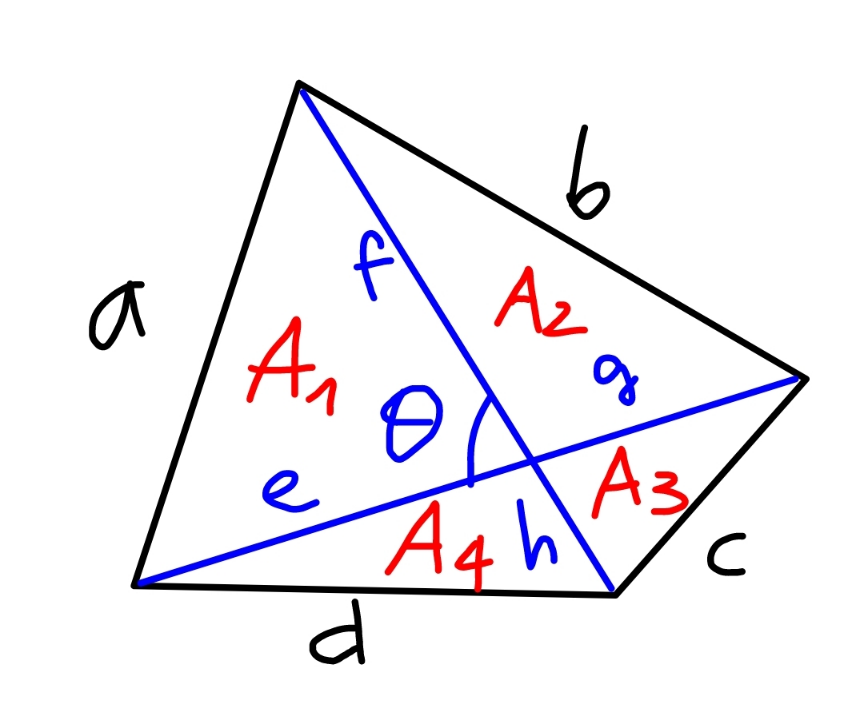
$${A}_{\mathrm{1}} =\frac{{ef}\:\mathrm{sin}\:\theta}{\mathrm{2}} \\ $$$${A}_{\mathrm{2}} =\frac{{fg}\:\mathrm{sin}\:\theta}{\mathrm{2}} \\ $$$${A}_{\mathrm{3}} =\frac{{gh}\:\mathrm{sin}\:\theta}{\mathrm{2}} \\ $$$${A}_{\mathrm{4}} =\frac{{he}\:\mathrm{sin}\:\theta}{\mathrm{2}} \\ $$$${A}={A}_{\mathrm{1}} +{A}_{\mathrm{2}} +{A}_{\mathrm{3}} +{A}_{\mathrm{4}} \\ $$$$ \\ $$$${a}^{\mathrm{2}} ={e}^{\mathrm{2}} +{f}^{\mathrm{2}} −\mathrm{2}{ef}\:\mathrm{cos}\:\theta \\ $$$$\Rightarrow{a}^{\mathrm{2}} ={e}^{\mathrm{2}} +{f}^{\mathrm{2}} −\frac{\mathrm{4}{A}_{\mathrm{1}} }{\mathrm{tan}\:\theta}\:\:\:…\left({i}\right) \\ $$$${b}^{\mathrm{2}} ={f}^{\mathrm{2}} +{g}^{\mathrm{2}} +\mathrm{2}{fg}\:\mathrm{cos}\:\theta \\ $$$$\Rightarrow{b}^{\mathrm{2}} ={f}^{\mathrm{2}} +{g}^{\mathrm{2}} +\frac{\mathrm{4}{A}_{\mathrm{2}} }{\mathrm{tan}\:\theta}\:\:\:…\left({ii}\right) \\ $$$${c}^{\mathrm{2}} ={g}^{\mathrm{2}} +{h}^{\mathrm{2}} −\mathrm{2}{gh}\:\mathrm{cos}\:\theta \\ $$$$\Rightarrow{c}^{\mathrm{2}} ={g}^{\mathrm{2}} +{h}^{\mathrm{2}} −\frac{\mathrm{4}{A}_{\mathrm{3}} }{\mathrm{tan}\:\theta}\:\:\:…\left({iii}\right) \\ $$$${d}^{\mathrm{2}} ={h}^{\mathrm{2}} +{e}^{\mathrm{2}} +\mathrm{2}{he}\:\mathrm{cos}\:\theta \\ $$$$\Rightarrow{d}^{\mathrm{2}} ={h}^{\mathrm{2}} +{e}^{\mathrm{2}} +\frac{\mathrm{4}{A}_{\mathrm{4}} }{\mathrm{tan}\:\theta}\:\:\:…\left({iv}\right) \\ $$$$\left({ii}\right)+\left({iv}\right)−\left({i}\right)−\left({iii}\right): \\ $$$$−{a}^{\mathrm{2}} −{c}^{\mathrm{2}} +{b}^{\mathrm{2}} +{d}^{\mathrm{2}} =\frac{\mathrm{4}\left({A}_{\mathrm{1}} +{A}_{\mathrm{2}} +{A}_{\mathrm{3}} +{A}_{\mathrm{4}} \right)}{\mathrm{tan}\:\theta} \\ $$$$\Rightarrow{A}=\frac{\mid\mathrm{tan}\:\theta\mid\mid\left({a}^{\mathrm{2}} +{c}^{\mathrm{2}} −{b}^{\mathrm{2}} −{d}^{\mathrm{2}} \right)\mid}{\mathrm{4}} \\ $$$$\:\:\:\:\left(\theta\neq\mathrm{90}°\right) \\ $$
Commented by Matica last updated on 16/Sep/22

$${Thank}\:{you} \\ $$
Commented by Tawa11 last updated on 18/Sep/22

$$\mathrm{Great}\:\mathrm{sir} \\ $$
