Menu Close

Question-176423

Tinku Tara 0 Comments
Pin




Question Number 176423 by Shrinava last updated on 18/Sep/22
Answered by a.lgnaoui last updated on 21/Sep/22
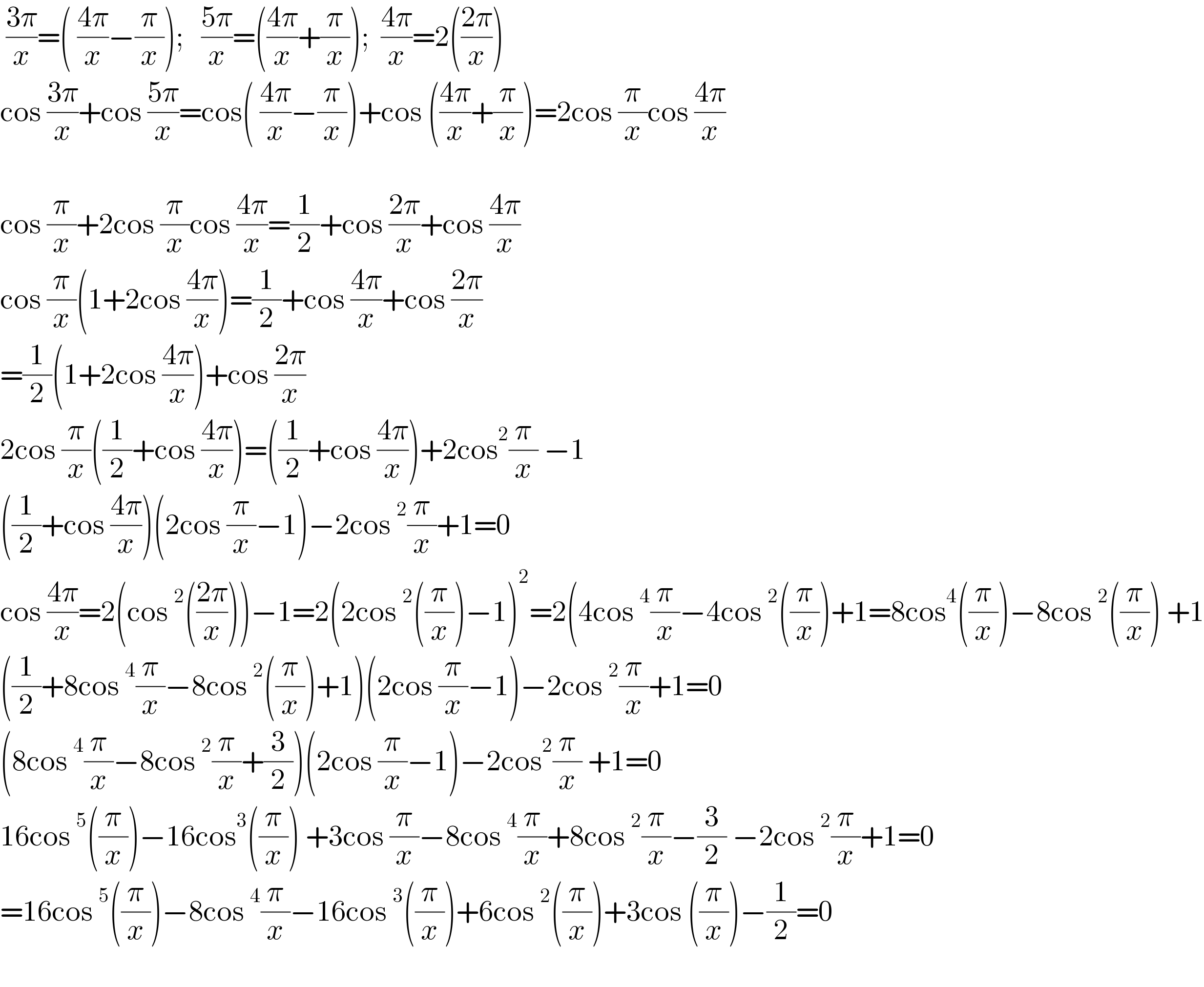
((3π)/x)=( ((4π)/x)−(π/x)); ((5π)/x)=(((4π)/x)+(π/x)); ((4π)/x)=2(((2π)/x)) cos ((3π)/x)+cos ((5π)/x)=cos( ((4π)/x)−(π/x))+cos (((4π)/x)+(π/x))=2cos (π/x)cos ((4π)/x) cos (π/x)+2cos (π/x)cos ((4π)/x)=(1/2)+cos ((2π)/x)+cos ((4π)/x) cos (π/x)(1+2cos ((4π)/x))=(1/2)+cos ((4π)/x)+cos ((2π)/x) =(1/2)(1+2cos ((4π)/x))+cos ((2π)/x) 2cos (π/x)((1/2)+cos ((4π)/x))=((1/2)+cos ((4π)/x))+2cos^2 (π/x) −1 ((1/2)+cos ((4π)/x))(2cos (π/x)−1)−2cos^2 (π/x)+1=0 cos ((4π)/x)=2(cos^2 (((2π)/x)))−1=2(2cos^2 ((π/x))−1)^2 =2(4cos^4 (π/x)−4cos^2 ((π/x))+1=8cos^4 ((π/x))−8cos^2 ((π/x)) +1 ((1/2)+8cos^4 (π/x)−8cos^2 ((π/x))+1)(2cos (π/x)−1)−2cos^2 (π/x)+1=0 (8cos^4 (π/x)−8cos^2 (π/x)+(3/2))(2cos (π/x)−1)−2cos^2 (π/x) +1=0 16cos^5 ((π/x))−16cos^3 ((π/x)) +3cos (π/x)−8cos^4 (π/x)+8cos^2 (π/x)−(3/2) −2cos^2 (π/x)+1=0 =16cos^5 ((π/x))−8cos^4 (π/x)−16cos^3 ((π/x))+6cos^2 ((π/x))+3cos ((π/x))−(1/2)=0
$$\:\frac{\mathrm{3}\pi}{{x}}=\left(\:\frac{\mathrm{4}\pi}{{x}}−\frac{\pi}{{x}}\right);\:\:\:\frac{\mathrm{5}\pi}{{x}}=\left(\frac{\mathrm{4}\pi}{{x}}+\frac{\pi}{{x}}\right);\:\:\frac{\mathrm{4}\pi}{{x}}=\mathrm{2}\left(\frac{\mathrm{2}\pi}{{x}}\right)\:\:\: \\ $$$$\mathrm{cos}\:\frac{\mathrm{3}\pi}{{x}}+\mathrm{cos}\:\frac{\mathrm{5}\pi}{{x}}=\mathrm{cos}\left(\:\frac{\mathrm{4}\pi}{{x}}−\frac{\pi}{{x}}\right)+\mathrm{cos}\:\left(\frac{\mathrm{4}\pi}{{x}}+\frac{\pi}{{x}}\right)=\mathrm{2cos}\:\frac{\pi}{{x}}\mathrm{cos}\:\frac{\mathrm{4}\pi}{{x}} \\ $$$$ \\ $$$$\mathrm{cos}\:\frac{\pi}{{x}}+\mathrm{2cos}\:\frac{\pi}{{x}}\mathrm{cos}\:\frac{\mathrm{4}\pi}{{x}}=\frac{\mathrm{1}}{\mathrm{2}}+\mathrm{cos}\:\frac{\mathrm{2}\pi}{{x}}+\mathrm{cos}\:\frac{\mathrm{4}\pi}{{x}} \\ $$$$\mathrm{cos}\:\frac{\pi}{{x}}\left(\mathrm{1}+\mathrm{2cos}\:\frac{\mathrm{4}\pi}{{x}}\right)=\frac{\mathrm{1}}{\mathrm{2}}+\mathrm{cos}\:\frac{\mathrm{4}\pi}{{x}}+\mathrm{cos}\:\frac{\mathrm{2}\pi}{{x}} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\left(\mathrm{1}+\mathrm{2cos}\:\frac{\mathrm{4}\pi}{{x}}\right)+\mathrm{cos}\:\frac{\mathrm{2}\pi}{{x}} \\ $$$$\mathrm{2cos}\:\frac{\pi}{{x}}\left(\frac{\mathrm{1}}{\mathrm{2}}+\mathrm{cos}\:\frac{\mathrm{4}\pi}{{x}}\right)=\left(\frac{\mathrm{1}}{\mathrm{2}}+\mathrm{cos}\:\frac{\mathrm{4}\pi}{{x}}\right)+\mathrm{2cos}^{\mathrm{2}} \frac{\pi}{{x}}\:−\mathrm{1} \\ $$$$\left(\frac{\mathrm{1}}{\mathrm{2}}+\mathrm{cos}\:\frac{\mathrm{4}\pi}{{x}}\right)\left(\mathrm{2cos}\:\frac{\pi}{{x}}−\mathrm{1}\right)−\mathrm{2cos}\:^{\mathrm{2}} \frac{\pi}{{x}}+\mathrm{1}=\mathrm{0} \\ $$$$\mathrm{cos}\:\frac{\mathrm{4}\pi}{{x}}=\mathrm{2}\left(\mathrm{cos}\:^{\mathrm{2}} \left(\frac{\mathrm{2}\pi}{{x}}\right)\right)−\mathrm{1}=\mathrm{2}\left(\mathrm{2cos}\:^{\mathrm{2}} \left(\frac{\pi}{{x}}\right)−\mathrm{1}\right)^{\mathrm{2}} =\mathrm{2}\left(\mathrm{4cos}\:^{\mathrm{4}} \frac{\pi}{{x}}−\mathrm{4cos}\:^{\mathrm{2}} \left(\frac{\pi}{{x}}\right)+\mathrm{1}=\mathrm{8cos}^{\mathrm{4}} \left(\frac{\pi}{{x}}\right)−\mathrm{8cos}\:^{\mathrm{2}} \left(\frac{\pi}{{x}}\right)\:+\mathrm{1}\right. \\ $$$$\left(\frac{\mathrm{1}}{\mathrm{2}}+\mathrm{8cos}\:^{\mathrm{4}} \frac{\pi}{{x}}−\mathrm{8cos}\:^{\mathrm{2}} \left(\frac{\pi}{{x}}\right)+\mathrm{1}\right)\left(\mathrm{2cos}\:\frac{\pi}{{x}}−\mathrm{1}\right)−\mathrm{2cos}\:^{\mathrm{2}} \frac{\pi}{{x}}+\mathrm{1}=\mathrm{0} \\ $$$$\left(\mathrm{8cos}\:^{\mathrm{4}} \frac{\pi}{{x}}−\mathrm{8cos}\:^{\mathrm{2}} \frac{\pi}{{x}}+\frac{\mathrm{3}}{\mathrm{2}}\right)\left(\mathrm{2cos}\:\frac{\pi}{{x}}−\mathrm{1}\right)−\mathrm{2cos}^{\mathrm{2}} \frac{\pi}{{x}}\:+\mathrm{1}=\mathrm{0} \\ $$$$\mathrm{16cos}\:^{\mathrm{5}} \left(\frac{\pi}{{x}}\right)−\mathrm{16cos}^{\mathrm{3}} \left(\frac{\pi}{{x}}\right)\:+\mathrm{3cos}\:\frac{\pi}{{x}}−\mathrm{8cos}\:^{\mathrm{4}} \frac{\pi}{{x}}+\mathrm{8cos}\:^{\mathrm{2}} \frac{\pi}{{x}}−\frac{\mathrm{3}}{\mathrm{2}}\:−\mathrm{2cos}\:^{\mathrm{2}} \frac{\pi}{{x}}+\mathrm{1}=\mathrm{0} \\ $$$$=\mathrm{16cos}\:^{\mathrm{5}} \left(\frac{\pi}{{x}}\right)−\mathrm{8cos}\:^{\mathrm{4}} \frac{\pi}{{x}}−\mathrm{16cos}\:^{\mathrm{3}} \left(\frac{\pi}{{x}}\right)+\mathrm{6cos}\:^{\mathrm{2}} \left(\frac{\pi}{{x}}\right)+\mathrm{3cos}\:\left(\frac{\pi}{{x}}\right)−\frac{\mathrm{1}}{\mathrm{2}}=\mathrm{0} \\ $$$$ \\ $$
Commented by a.lgnaoui last updated on 21/Sep/22
Commented by a.lgnaoui last updated on 21/Sep/22
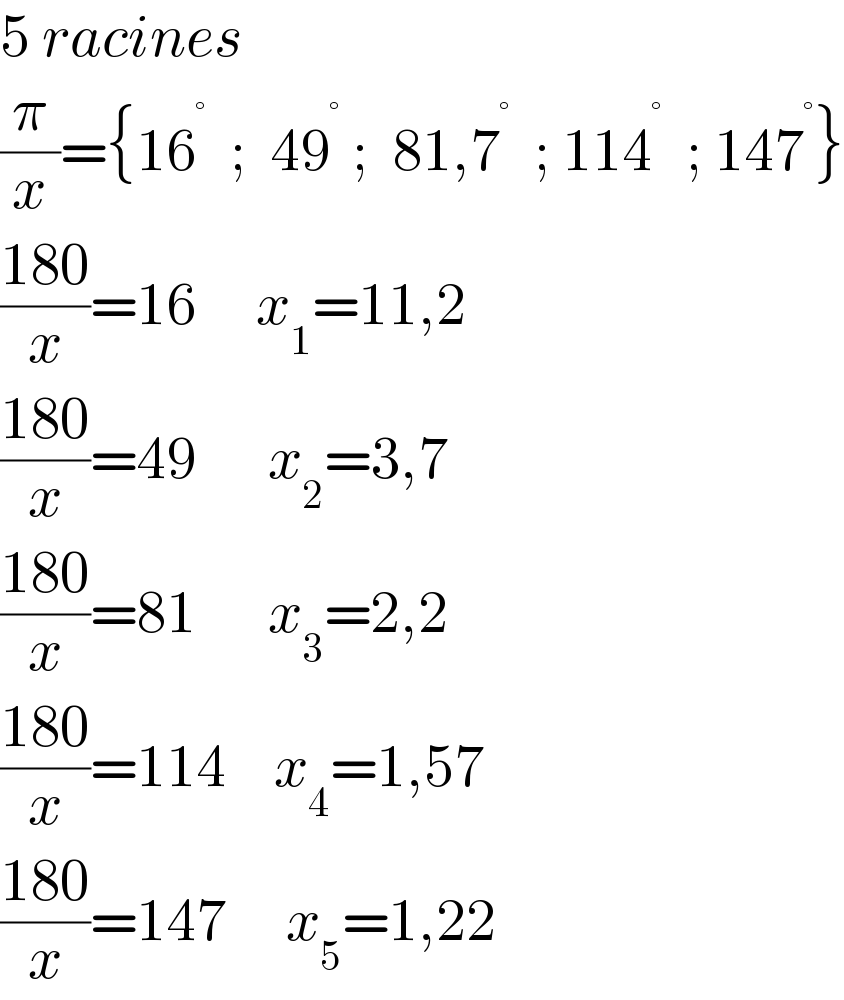
5 racines (π/x)={16^° ; 49^° ; 81,7^° ; 114^° ; 147^° } ((180)/x)=16 x_1 =11,2 ((180)/x)=49 x_2 =3,7 ((180)/x)=81 x_3 =2,2 ((180)/x)=114 x_4 =1,57 ((180)/x)=147 x_5 =1,22
$$\mathrm{5}\:{racines} \\ $$$$\frac{\pi}{{x}}=\left\{\mathrm{16}^{°} \:\:;\:\:\mathrm{49}^{°} \:;\:\:\mathrm{81},\mathrm{7}^{°} \:\:;\:\mathrm{114}^{°} \:\:;\:\mathrm{147}^{°} \right\} \\ $$$$\frac{\mathrm{180}}{{x}}=\mathrm{16}\:\:\:\:\:{x}_{\mathrm{1}} =\mathrm{11},\mathrm{2} \\ $$$$\frac{\mathrm{180}}{{x}}=\mathrm{49}\:\:\:\:\:\:{x}_{\mathrm{2}} =\mathrm{3},\mathrm{7} \\ $$$$\frac{\mathrm{180}}{{x}}=\mathrm{81}\:\:\:\:\:\:{x}_{\mathrm{3}} =\mathrm{2},\mathrm{2} \\ $$$$\frac{\mathrm{180}}{{x}}=\mathrm{114}\:\:\:\:{x}_{\mathrm{4}} =\mathrm{1},\mathrm{57} \\ $$$$\frac{\mathrm{180}}{{x}}=\mathrm{147}\:\:\:\:\:{x}_{\mathrm{5}} =\mathrm{1},\mathrm{22} \\ $$
Commented by Shrinava last updated on 23/Sep/22
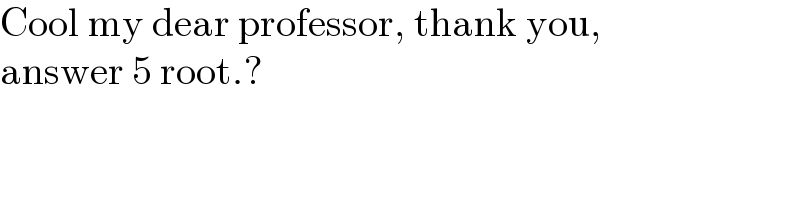
Cool my dear professor, thank you, answer 5 root.?
$$\mathrm{Cool}\:\mathrm{my}\:\mathrm{dear}\:\mathrm{professor},\:\mathrm{thank}\:\mathrm{you}, \\ $$$$\mathrm{answer}\:\mathrm{5}\:\mathrm{root}.? \\ $$
Commented by a.lgnaoui last updated on 23/Sep/22
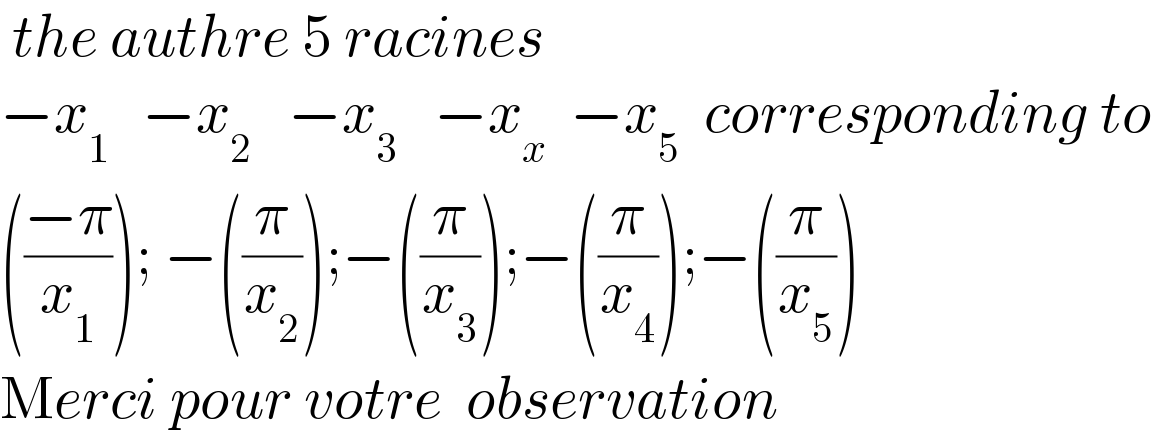
the authre 5 racines −x_(1 ) −x_2 −x_3 −x_x −x_5 corresponding to (((−π)/x_1 )); −((π/x_2 ));−((π/x_3 ));−((π/x_4 ));−((π/x_5 )) Merci pour votre observation
$$\:{the}\:{authre}\:\mathrm{5}\:{racines} \\ $$$$−{x}_{\mathrm{1}\:} \:\:−{x}_{\mathrm{2}} \:\:\:−{x}_{\mathrm{3}} \:\:\:−{x}_{{x}} \:\:−{x}_{\mathrm{5}} \:\:{corresponding}\:{to} \\ $$$$\left(\frac{−\pi}{{x}_{\mathrm{1}} }\right);\:−\left(\frac{\pi}{{x}_{\mathrm{2}} }\right);−\left(\frac{\pi}{{x}_{\mathrm{3}} }\right);−\left(\frac{\pi}{{x}_{\mathrm{4}} }\right);−\left(\frac{\pi}{{x}_{\mathrm{5}} }\right) \\ $$$$\mathrm{M}{erci}\:{pour}\:{votre}\:\:{observation} \\ $$
Commented by Shrinava last updated on 25/Sep/22
Thankyou dear professor, answer? please
$$\mathrm{Thankyou}\:\mathrm{dear}\:\mathrm{professor},\:\mathrm{answer}?\:\mathrm{please} \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *