Question Number 177005 by Ar Brandon last updated on 29/Sep/22
Answered by Rasheed.Sindhi last updated on 29/Sep/22
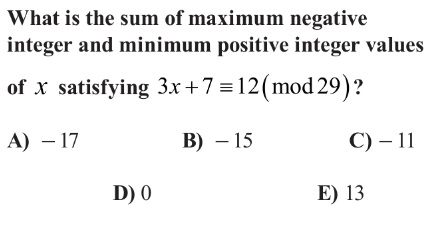
![3x+7≡12[29] 3x≡5+29×2[29] 3x≡63[29] x=21[29] x=21+29k max negative value:x=21+29(−1)=−8 min positive value:x=21+29(0)=21 Total=13 (Option E)](https://www.tinkutara.com/question/Q177018.png)
$$\mathrm{3}{x}+\mathrm{7}\equiv\mathrm{12}\left[\mathrm{29}\right] \\ $$$$\mathrm{3}{x}\equiv\mathrm{5}+\mathrm{29}×\mathrm{2}\left[\mathrm{29}\right] \\ $$$$\mathrm{3}{x}\equiv\mathrm{63}\left[\mathrm{29}\right] \\ $$$${x}=\mathrm{21}\left[\mathrm{29}\right] \\ $$$${x}=\mathrm{21}+\mathrm{29}{k} \\ $$$${max}\:{negative}\:{value}:{x}=\mathrm{21}+\mathrm{29}\left(−\mathrm{1}\right)=−\mathrm{8} \\ $$$${min}\:{positive}\:{value}:{x}=\mathrm{21}+\mathrm{29}\left(\mathrm{0}\right)=\mathrm{21} \\ $$$$\mathcal{T}{otal}=\mathrm{13}\:\:\left({Option}\:{E}\right) \\ $$
Commented by Ar Brandon last updated on 29/Sep/22
Thank you Sir
Commented by Tawa11 last updated on 02/Oct/22

$$\mathrm{Great}\:\mathrm{sir} \\ $$
