Question-178833

Question Number 178833 by Spillover last updated on 22/Oct/22

Answered by cortano1 last updated on 22/Oct/22

(b) L_{3} \equiv L_{2} + k (L_{1} - L_{2}) = 0

\Rightarrow x^{2} + y^{2} - 10 x - 12 y + 40 + k (8 x + 8 y - 44) = 0

\Rightarrow x^{2} + y^{2} + 2 (4 k - 5) x + 2 (4 k - 6) y + 40 - 44 k = 0

whose radius = 4

\Rightarrow \sqrt{{(4 k - 5)}^{2} + {(4 k - 6)}^{2} + 44 k - 40} = 4

let 4 k = λ

\Rightarrow {(λ - 5)}^{2} + {(λ - 6)}^{2} + 11 λ - 40 = 16

\Rightarrow 2 λ^{2} - 11 λ + 5 = 0

\Rightarrow λ_{1} = 5 = 4 k & λ_{2} = \frac{1}{2} = 4 k

\Rightarrow L_{3} \equiv x^{2} + y^{2} - 2 y - 15 = 0 or

\Rightarrow L_{3} \equiv {2 x}^{2} + {2 y}^{2} - 18 x - 22 y + 69 = 0

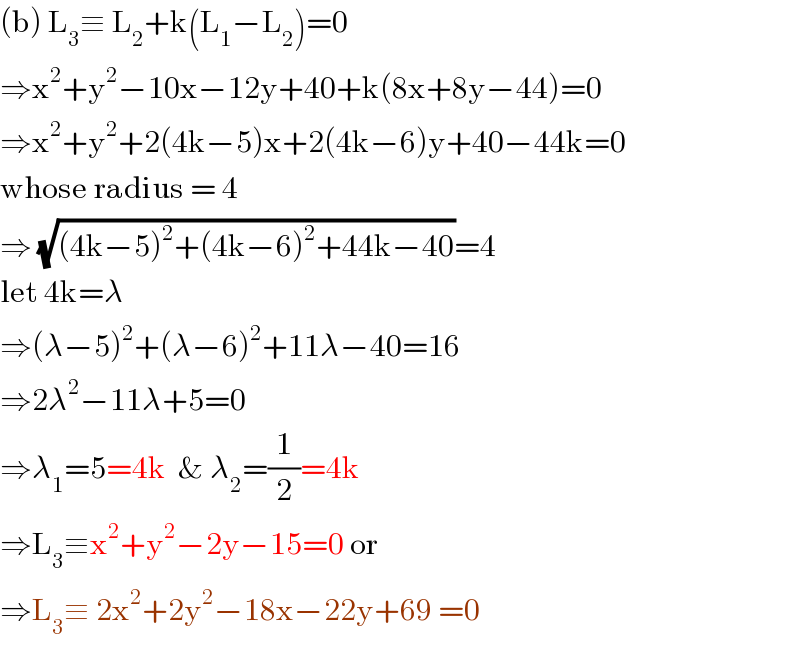
Commented by cortano1 last updated on 22/Oct/22

Answered by Spillover last updated on 26/Dec/22

Answered by Spillover last updated on 26/Dec/22

Answered by Spillover last updated on 26/Dec/22

Answered by Spillover last updated on 26/Dec/22

Answered by Spillover last updated on 26/Dec/22