Question-178839

Question Number 178839 by ARUNG_Brandon_MBU last updated on 22/Oct/22

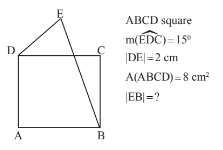
Answered by HeferH last updated on 22/Oct/22

S i d e o f t h e s q u a r e = \sqrt{8} = 2 \sqrt{2}

l_{1} = 2 \sqrt{2} - (\frac{\sqrt{6} + \sqrt{2}}{2}) = \frac{3 \sqrt{2} - \sqrt{6}}{2}

l_{2} = 2 \sqrt{2} + (\frac{\sqrt{6} - \sqrt{2}}{2}) = \frac{3 \sqrt{2} + \sqrt{6}}{2}

l_{1}^{2} + l_{2}^{2} = {E B}^{2}

E B = \sqrt{12} = 2 \sqrt{3}

Commented by HeferH last updated on 22/Oct/22

Commented by ARUNG_Brandon_MBU last updated on 22/Oct/22

Thank you Sir

Commented by ARUNG_Brandon_MBU last updated on 22/Oct/22

Any explanations, please?

Commented by HeferH last updated on 22/Oct/22

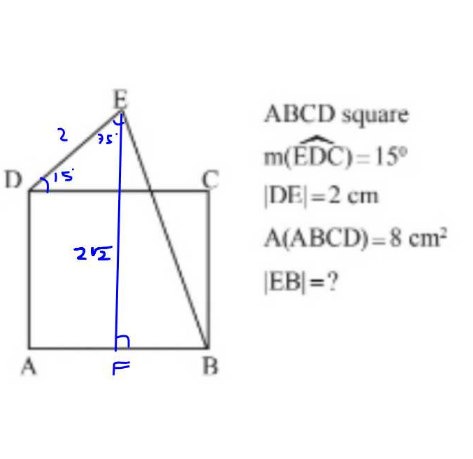
Commented by ARUNG_Brandon_MBU last updated on 22/Oct/22

OK thanks. I get it now.

Commented by Rasheed.Sindhi last updated on 22/Oct/22

I {d i d n}^{'} t g e t i t s i r, p l e a s e e x p l a i n i n

s o m e d e t a i l .

Commented by HeferH last updated on 22/Oct/22

F E : l_{2}

F B : l_{1}

Commented by Rasheed.Sindhi last updated on 23/Oct/22

P l e a s e e x e c u s e m e f o r l a c k o f f u n d a m e n t a l s .

A c t u a l l y I {c a n}^{'} t u n d e r s t a n d w h e r e t h e

t h e v a l u e s \sqrt{6} - \sqrt{2} & \sqrt{6} + \sqrt{2} h a v e

c o m e f r o m ? A n d w h y y o u r & m y

a n s w e r s a r e d i f f e r e n t ?

Commented by Ar Brandon last updated on 23/Oct/22

They are the values of \sin 15 ° and \cos 15 °, Sir .

\sin 15 ° = \frac{\sqrt{6} - \sqrt{2}}{4}, \cos 15 ° = \frac{\sqrt{6} + \sqrt{2}}{4}

Commented by Rasheed.Sindhi last updated on 24/Oct/22

Ok, thanx Brandon sir!

Answered by Rasheed.Sindhi last updated on 22/Oct/22

= s^{2} = 8; B D = \sqrt{s^{2} + s^{2}} = \sqrt{8^{2} + 8^{2}} = 8 \sqrt{2}

∠ B D E = 45 + 15 = 60

△ B D E :

E B = \sqrt{{B D}^{2} + {D E}^{2} - 2 B D \cdot D E \cdot \cos ∠ B D E}

= \sqrt{{(8 \sqrt{2})}^{2} + 2^{2} - 2 (8 \sqrt{2}) (2) \cos 60}

= \sqrt{128 + 4 - 2 (8 \sqrt{2}) (2) (1 / 2)}

= \sqrt{132 - 16 \sqrt{2}}

= 2 \sqrt{33 - 4 \sqrt{2}}

Commented by ARUNG_Brandon_MBU last updated on 22/Oct/22

Thank you Sir