Menu Close

Question-178867

Tinku Tara 0 Comments
Pin




Question Number 178867 by Spillover last updated on 22/Oct/22
Answered by Spillover last updated on 15/Jan/23
f(x)=x^4 −7x^3 +1 requare to show f(x_o )×f(x_1 )<0 given 0<x<1 f(x)=x^4 −7x^3 +1 f(0)=1 f(1)=−5 f(x_o )×f(x_1 )<0 1×−5<0 hence the root lies btn 0<x<1
$${f}\left({x}\right)={x}^{\mathrm{4}} −\mathrm{7}{x}^{\mathrm{3}} +\mathrm{1} \\ $$$${requare}\:{to}\:{show} \\ $$$${f}\left({x}_{{o}} \right)×{f}\left({x}_{\mathrm{1}} \right)<\mathrm{0} \\ $$$${given}\:\:\mathrm{0}<{x}<\mathrm{1} \\ $$$${f}\left({x}\right)={x}^{\mathrm{4}} −\mathrm{7}{x}^{\mathrm{3}} +\mathrm{1} \\ $$$${f}\left(\mathrm{0}\right)=\mathrm{1}\:\:\:{f}\left(\mathrm{1}\right)=−\mathrm{5} \\ $$$${f}\left({x}_{{o}} \right)×{f}\left({x}_{\mathrm{1}} \right)<\mathrm{0} \\ $$$$\mathrm{1}×−\mathrm{5}<\mathrm{0} \\ $$$${hence}\:{the}\:{root}\:{lies}\:{btn}\:\mathrm{0}<{x}<\mathrm{1} \\ $$$$ \\ $$
Answered by Spillover last updated on 15/Jan/23
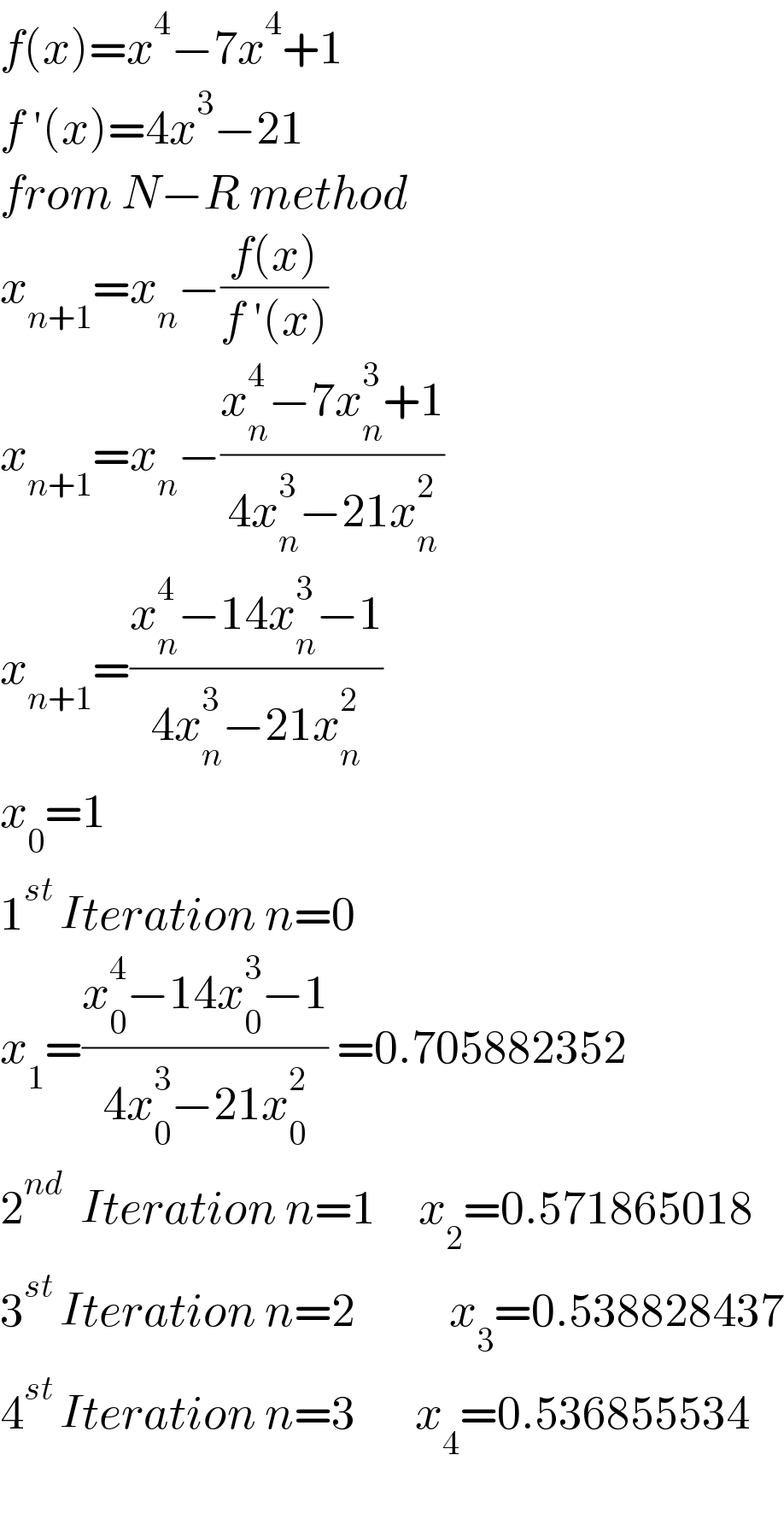
f(x)=x^4 −7x^4 +1 f ′(x)=4x^3 −21 from N−R method x_(n+1) =x_n −((f(x))/(f ′(x))) x_(n+1) =x_n −((x_n ^4 −7x_n ^3 +1)/(4x_n ^3 −21x_n ^2 )) x_(n+1) =((x_n ^4 −14x_n ^3 −1)/(4x_n ^3 −21x_n ^2 )) x_0 =1 1^(st ) Iteration n=0 x_1 =((x_0 ^4 −14x_0 ^3 −1)/(4x_0 ^3 −21x_0 ^2 )) =0.705882352 2^(nd) Iteration n=1 x_2 =0.571865018 3^(st ) Iteration n=2 x_3 =0.538828437 4^(st ) Iteration n=3 x_4 =0.536855534
$${f}\left({x}\right)={x}^{\mathrm{4}} −\mathrm{7}{x}^{\mathrm{4}} +\mathrm{1} \\ $$$${f}\:'\left({x}\right)=\mathrm{4}{x}^{\mathrm{3}} −\mathrm{21} \\ $$$${from}\:{N}−{R}\:{method} \\ $$$${x}_{{n}+\mathrm{1}} ={x}_{{n}} −\frac{{f}\left({x}\right)}{{f}\:'\left({x}\right)} \\ $$$${x}_{{n}+\mathrm{1}} ={x}_{{n}} −\frac{{x}_{{n}} ^{\mathrm{4}} −\mathrm{7}{x}_{{n}} ^{\mathrm{3}} +\mathrm{1}}{\mathrm{4}{x}_{{n}} ^{\mathrm{3}} −\mathrm{21}{x}_{{n}} ^{\mathrm{2}} } \\ $$$${x}_{{n}+\mathrm{1}} =\frac{{x}_{{n}} ^{\mathrm{4}} −\mathrm{14}{x}_{{n}} ^{\mathrm{3}} −\mathrm{1}}{\mathrm{4}{x}_{{n}} ^{\mathrm{3}} −\mathrm{21}{x}_{{n}} ^{\mathrm{2}} } \\ $$$${x}_{\mathrm{0}} =\mathrm{1} \\ $$$$\mathrm{1}^{{st}\:} {Iteration}\:{n}=\mathrm{0} \\ $$$${x}_{\mathrm{1}} =\frac{{x}_{\mathrm{0}} ^{\mathrm{4}} −\mathrm{14}{x}_{\mathrm{0}} ^{\mathrm{3}} −\mathrm{1}}{\mathrm{4}{x}_{\mathrm{0}} ^{\mathrm{3}} −\mathrm{21}{x}_{\mathrm{0}} ^{\mathrm{2}} }\:=\mathrm{0}.\mathrm{705882352} \\ $$$$\mathrm{2}^{{nd}} \:\:{Iteration}\:{n}=\mathrm{1}\:\:\:\:\:{x}_{\mathrm{2}} =\mathrm{0}.\mathrm{571865018} \\ $$$$\mathrm{3}^{{st}\:} {Iteration}\:{n}=\mathrm{2}\:\:\:\:\:\:\:\:\:\:\:{x}_{\mathrm{3}} =\mathrm{0}.\mathrm{538828437} \\ $$$$\mathrm{4}^{{st}\:} {Iteration}\:{n}=\mathrm{3}\:\:\:\:\:\:\:{x}_{\mathrm{4}} =\mathrm{0}.\mathrm{536855534} \\ $$$$ \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *