Question Number 180107 by yaslm last updated on 07/Nov/22
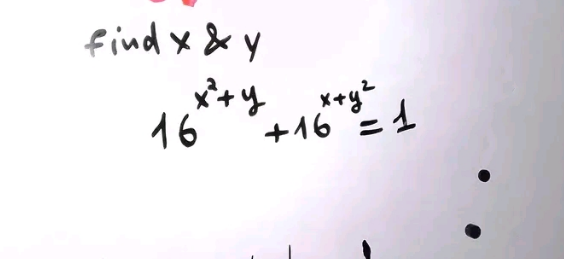
Commented by MJS_new last updated on 07/Nov/22

$$\mathrm{only}\:\mathrm{real}\:\mathrm{solution} \\ $$$${x}={y}=−\frac{\mathrm{1}}{\mathrm{2}} \\ $$
Commented by Rasheed.Sindhi last updated on 08/Nov/22
![16^(x^2 +y) +16^(x+y^2 ) =1 16^(x+y) (16^x +16^y )=1 16^(x+y) =(1/(16^x +16^y )) ab=(1/(a+b)) ; [16^x =a,16^y =b] .... ...](https://www.tinkutara.com/question/Q180166.png)
$$\mathrm{16}^{{x}^{\mathrm{2}} +{y}} +\mathrm{16}^{{x}+{y}^{\mathrm{2}} } =\mathrm{1} \\ $$$$\mathrm{16}^{{x}+{y}} \left(\mathrm{16}^{{x}} +\mathrm{16}^{{y}} \right)=\mathrm{1} \\ $$$$\mathrm{16}^{{x}+{y}} =\frac{\mathrm{1}}{\mathrm{16}^{{x}} +\mathrm{16}^{{y}} } \\ $$$${ab}=\frac{\mathrm{1}}{{a}+{b}}\:\:;\:\left[\mathrm{16}^{{x}} ={a},\mathrm{16}^{{y}} ={b}\right] \\ $$$$…. \\ $$$$… \\ $$
Commented by MJS_new last updated on 08/Nov/22
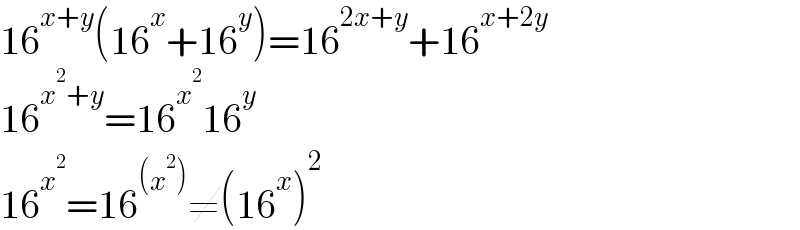
$$\mathrm{16}^{{x}+{y}} \left(\mathrm{16}^{{x}} +\mathrm{16}^{{y}} \right)=\mathrm{16}^{\mathrm{2}{x}+{y}} +\mathrm{16}^{{x}+\mathrm{2}{y}} \\ $$$$\mathrm{16}^{{x}^{\mathrm{2}} +{y}} =\mathrm{16}^{{x}^{\mathrm{2}} } \mathrm{16}^{{y}} \\ $$$$\mathrm{16}^{{x}^{\mathrm{2}} } =\mathrm{16}^{\left({x}^{\mathrm{2}} \right)} \neq\left(\mathrm{16}^{{x}} \right)^{\mathrm{2}} \\ $$
Commented by Rasheed.Sindhi last updated on 08/Nov/22
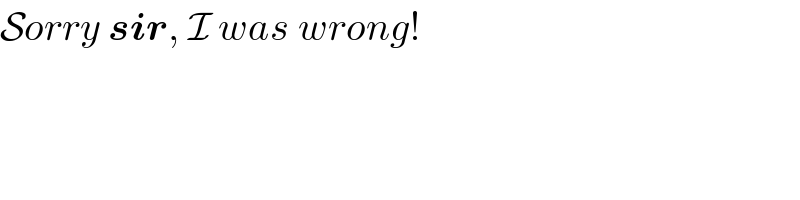
$$\mathcal{S}{orry}\:\boldsymbol{{sir}},\:\mathcal{I}\:{was}\:{wrong}! \\ $$
Commented by MJS_new last updated on 08/Nov/22
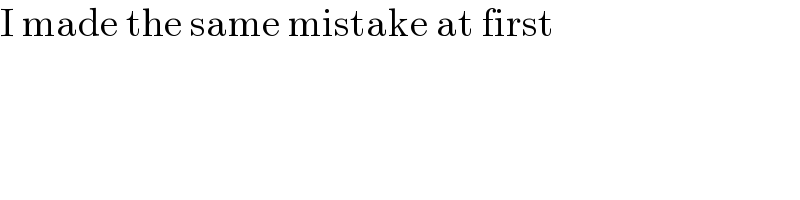
$$\mathrm{I}\:\mathrm{made}\:\mathrm{the}\:\mathrm{same}\:\mathrm{mistake}\:\mathrm{at}\:\mathrm{first} \\ $$
Commented by Rasheed.Sindhi last updated on 08/Nov/22
So I'm not alone! ��
Answered by manxsol last updated on 08/Nov/22

