Question Number 180547 by mnjuly1970 last updated on 13/Nov/22
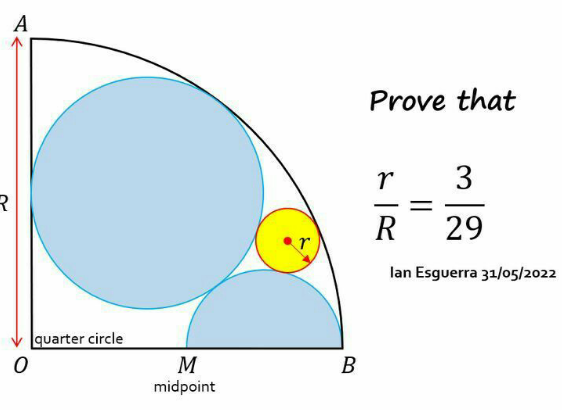
Answered by mr W last updated on 13/Nov/22
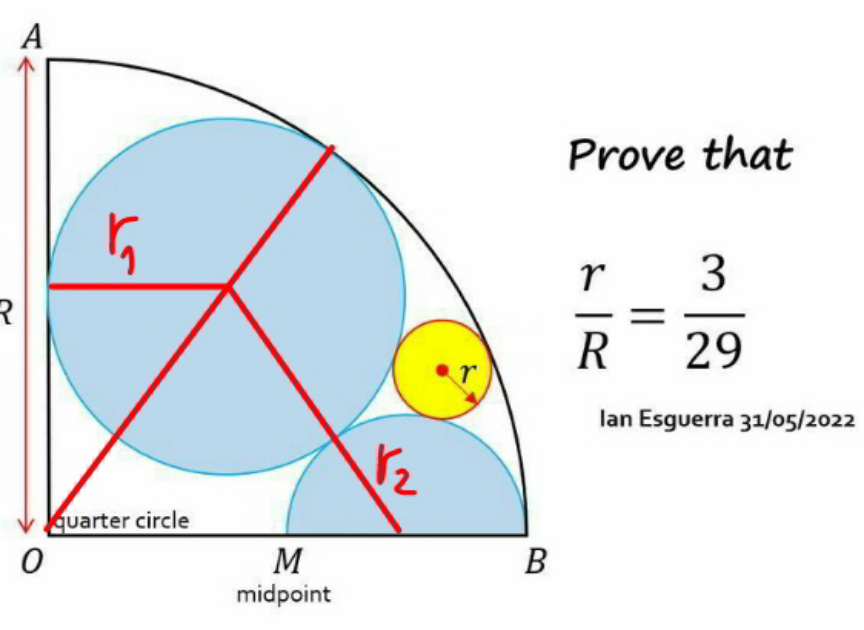
Commented by mr W last updated on 13/Nov/22
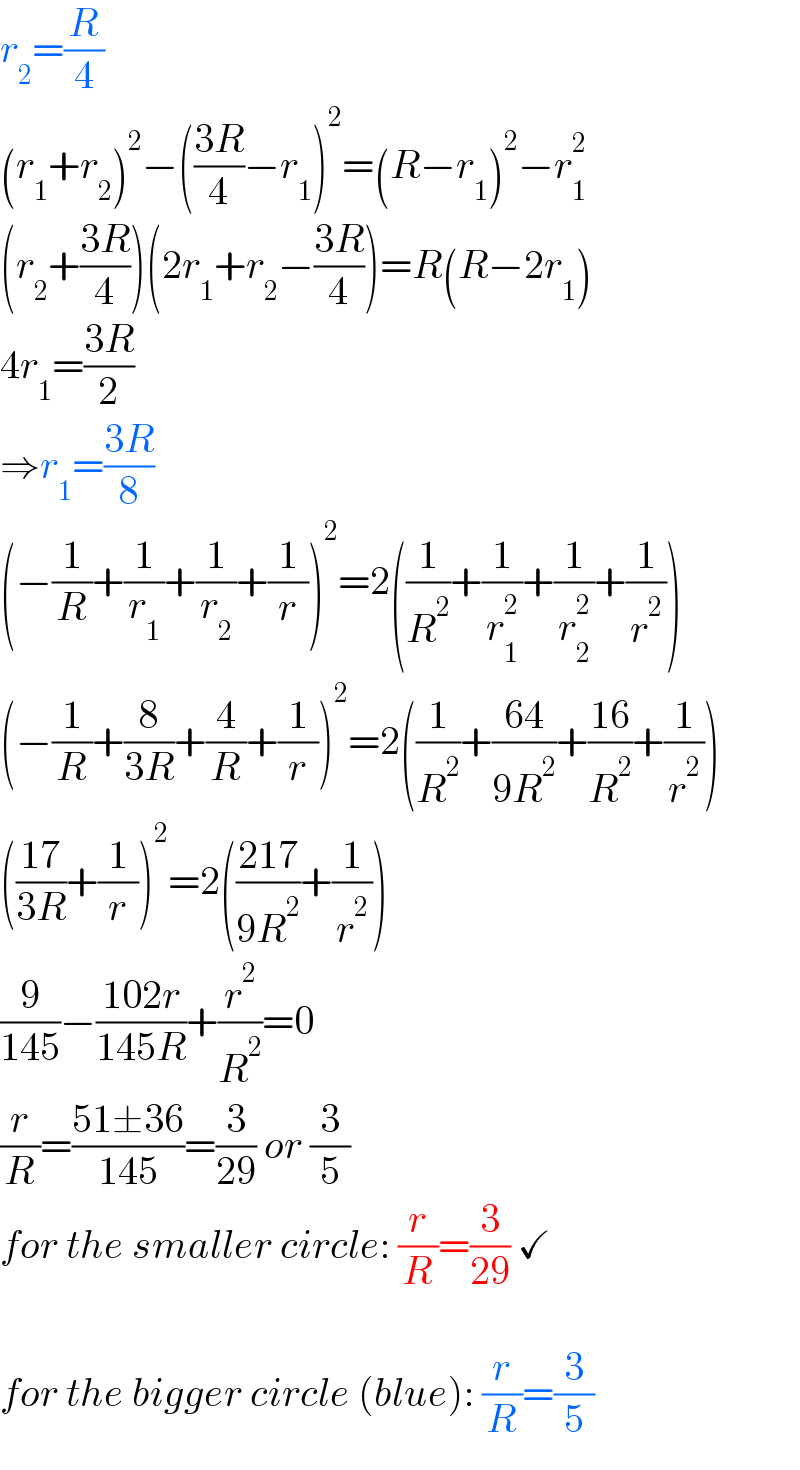
$${r}_{\mathrm{2}} =\frac{{R}}{\mathrm{4}} \\ $$$$\left({r}_{\mathrm{1}} +{r}_{\mathrm{2}} \right)^{\mathrm{2}} −\left(\frac{\mathrm{3}{R}}{\mathrm{4}}−{r}_{\mathrm{1}} \right)^{\mathrm{2}} =\left({R}−{r}_{\mathrm{1}} \right)^{\mathrm{2}} −{r}_{\mathrm{1}} ^{\mathrm{2}} \\ $$$$\left({r}_{\mathrm{2}} +\frac{\mathrm{3}{R}}{\mathrm{4}}\right)\left(\mathrm{2}{r}_{\mathrm{1}} +{r}_{\mathrm{2}} −\frac{\mathrm{3}{R}}{\mathrm{4}}\right)={R}\left({R}−\mathrm{2}{r}_{\mathrm{1}} \right) \\ $$$$\mathrm{4}{r}_{\mathrm{1}} =\frac{\mathrm{3}{R}}{\mathrm{2}} \\ $$$$\Rightarrow{r}_{\mathrm{1}} =\frac{\mathrm{3}{R}}{\mathrm{8}} \\ $$$$\left(−\frac{\mathrm{1}}{{R}}+\frac{\mathrm{1}}{{r}_{\mathrm{1}} }+\frac{\mathrm{1}}{{r}_{\mathrm{2}} }+\frac{\mathrm{1}}{{r}}\right)^{\mathrm{2}} =\mathrm{2}\left(\frac{\mathrm{1}}{{R}^{\mathrm{2}} }+\frac{\mathrm{1}}{{r}_{\mathrm{1}} ^{\mathrm{2}} }+\frac{\mathrm{1}}{{r}_{\mathrm{2}} ^{\mathrm{2}} }+\frac{\mathrm{1}}{{r}^{\mathrm{2}} }\right) \\ $$$$\left(−\frac{\mathrm{1}}{{R}}+\frac{\mathrm{8}}{\mathrm{3}{R}}+\frac{\mathrm{4}}{{R}}+\frac{\mathrm{1}}{{r}}\right)^{\mathrm{2}} =\mathrm{2}\left(\frac{\mathrm{1}}{{R}^{\mathrm{2}} }+\frac{\mathrm{64}}{\mathrm{9}{R}^{\mathrm{2}} }+\frac{\mathrm{16}}{{R}^{\mathrm{2}} }+\frac{\mathrm{1}}{{r}^{\mathrm{2}} }\right) \\ $$$$\left(\frac{\mathrm{17}}{\mathrm{3}{R}}+\frac{\mathrm{1}}{{r}}\right)^{\mathrm{2}} =\mathrm{2}\left(\frac{\mathrm{217}}{\mathrm{9}{R}^{\mathrm{2}} }+\frac{\mathrm{1}}{{r}^{\mathrm{2}} }\right) \\ $$$$\frac{\mathrm{9}}{\mathrm{145}}−\frac{\mathrm{102}{r}}{\mathrm{145}{R}}+\frac{{r}^{\mathrm{2}} }{{R}^{\mathrm{2}} }=\mathrm{0} \\ $$$$\frac{{r}}{{R}}=\frac{\mathrm{51}\pm\mathrm{36}}{\mathrm{145}}=\frac{\mathrm{3}}{\mathrm{29}}\:{or}\:\frac{\mathrm{3}}{\mathrm{5}} \\ $$$${for}\:{the}\:{smaller}\:{circle}:\:\frac{{r}}{{R}}=\frac{\mathrm{3}}{\mathrm{29}}\:\checkmark \\ $$$$ \\ $$$${for}\:{the}\:{bigger}\:{circle}\:\left({blue}\right):\:\frac{{r}}{{R}}=\frac{\mathrm{3}}{\mathrm{5}} \\ $$
Commented by mr W last updated on 13/Nov/22
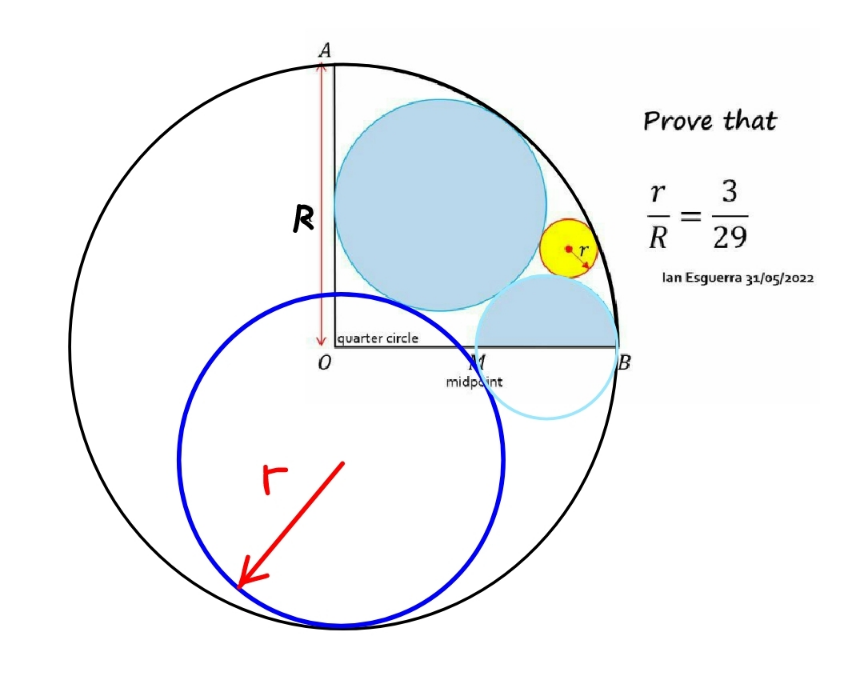
Commented by mnjuly1970 last updated on 14/Nov/22
$${grateful}\:{sir}\:{W}\: \\ $$$${excellent}\:{as}\:{always} \\ $$
