Question Number 181651 by mr W last updated on 28/Nov/22
Commented by Acem last updated on 05/Dec/22

$${Did}\:{you}\:{both}\:{believe}\:{him}\:{with}\:{all}\:{your}\:{minds}? \\ $$$${He}\:{just}\:{trying}\:{to}\:{make}\:{you}\:{feel}\:{remorse}\:{and} \\ $$$$\:{give}\:{the}\:{goat}\:{eternal}\:{rest}\:{and}\:{then}\:{invite}\:{him} \\ $$$$\:{to}\:{a}\:{barbecue} \\ $$
Commented by mr W last updated on 28/Nov/22

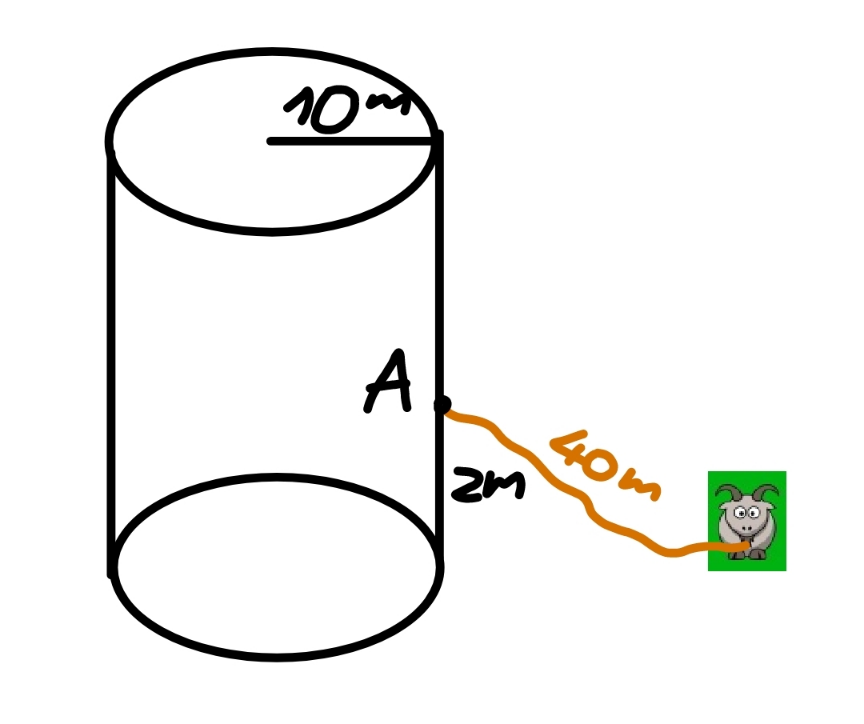
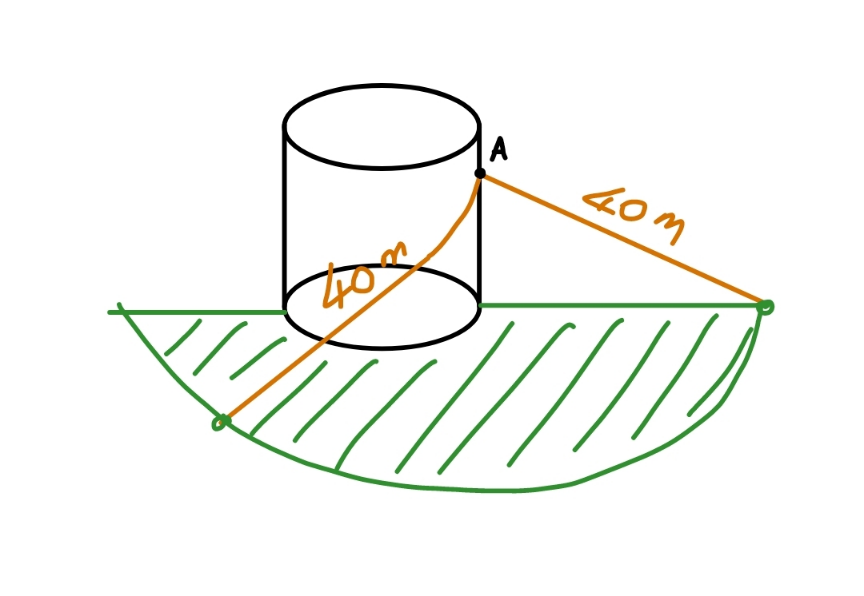
$${a}\:{goat}\:{is}\:{tied}\:{with}\:{a}\:\mathrm{40}{m}\:{long}\:{rope} \\ $$$${on}\:{a}\:{silo}\:{at}\:{a}\:{height}\:{of}\:\mathrm{2}{m}.\:{the}\:{radius} \\ $$$${of}\:{the}\:{silo}\:{is}\:\mathrm{10}{m}.\:{find}\:{the}\:{area}\: \\ $$$${where}\:{the}\:{goat}\:{can}\:{graze}\:{around}\:{the} \\ $$$${silo}. \\ $$
Commented by Frix last updated on 28/Nov/22

$$\mathrm{What}\:\mathrm{about}\:\mathrm{the}\:\mathrm{Animal}\:\mathrm{Welfare}?! \\ $$
Commented by mr W last updated on 28/Nov/22
$${are}\:{you}\:{seeing}\:{that}\:{the}\:{goat}\:{is}\: \\ $$$${suffering}? \\ $$
Commented by Frix last updated on 28/Nov/22
Ask the goat... ��
Commented by mr W last updated on 29/Nov/22

$${when}\:{you}\:{know}\:{that}\:{the}\:{goat}\:{is} \\ $$$${suffering},\:{then}\:{either}\:{you}\:{have}\:{asked} \\ $$$${the}\:{goat}\:{or}\:\:…\: \\ $$$${otherwise}\:{how}\:{could}\:{you}\:{know}\:{that}? \\ $$
Commented by MJS_new last updated on 30/Nov/22

$$\mathrm{my}\:\mathrm{grandmother}'\mathrm{s}\:\mathrm{goat}\:\mathrm{would}\:\mathrm{have}\:\mathrm{eaten} \\ $$$$\mathrm{the}\:\mathrm{rope}… \\ $$
Answered by a.lgnaoui last updated on 28/Nov/22
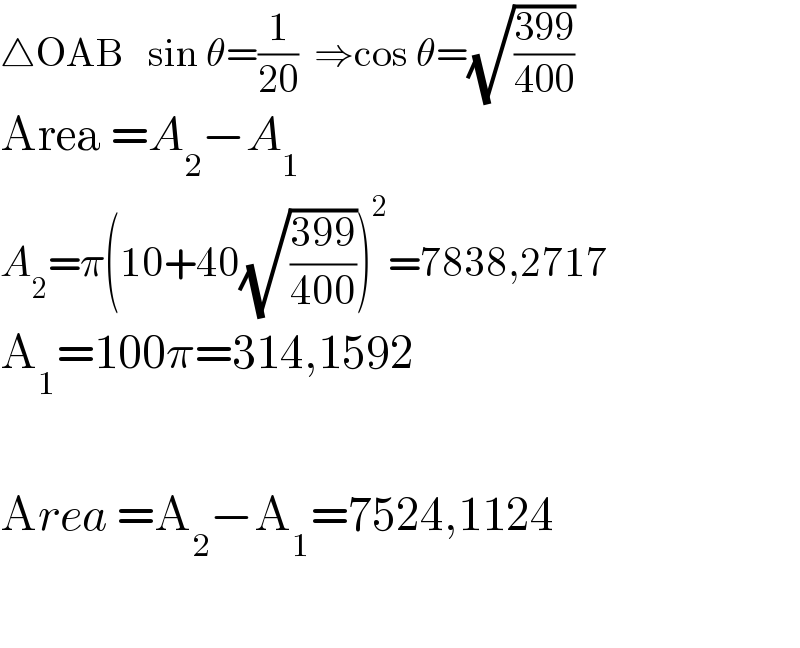
$$\bigtriangleup\mathrm{OAB}\:\:\:\mathrm{sin}\:\theta=\frac{\mathrm{1}}{\mathrm{20}}\:\:\Rightarrow\mathrm{cos}\:\theta=\sqrt{\frac{\mathrm{399}}{\mathrm{400}}} \\ $$$$\mathrm{Area}\:={A}_{\mathrm{2}} −{A}_{\mathrm{1}} \\ $$$${A}_{\mathrm{2}} =\pi\left(\mathrm{10}+\mathrm{40}\sqrt{\frac{\mathrm{399}}{\mathrm{400}}}\right)^{\mathrm{2}} =\mathrm{7838},\mathrm{2717} \\ $$$$\mathrm{A}_{\mathrm{1}} =\mathrm{100}\pi=\mathrm{314},\mathrm{1592} \\ $$$$ \\ $$$$\mathrm{A}{rea}\:=\mathrm{A}_{\mathrm{2}} −\mathrm{A}_{\mathrm{1}} =\mathrm{7524},\mathrm{1124} \\ $$$$ \\ $$
Commented by a.lgnaoui last updated on 28/Nov/22

Commented by mr W last updated on 28/Nov/22
$${wrong}!\: \\ $$$${the}\:{rope}\:{is}\:{fixed}\:{on}\:{the}\:{silo}. \\ $$
Commented by mr W last updated on 28/Nov/22
Answered by Acem last updated on 28/Nov/22
Commented by Acem last updated on 28/Nov/22

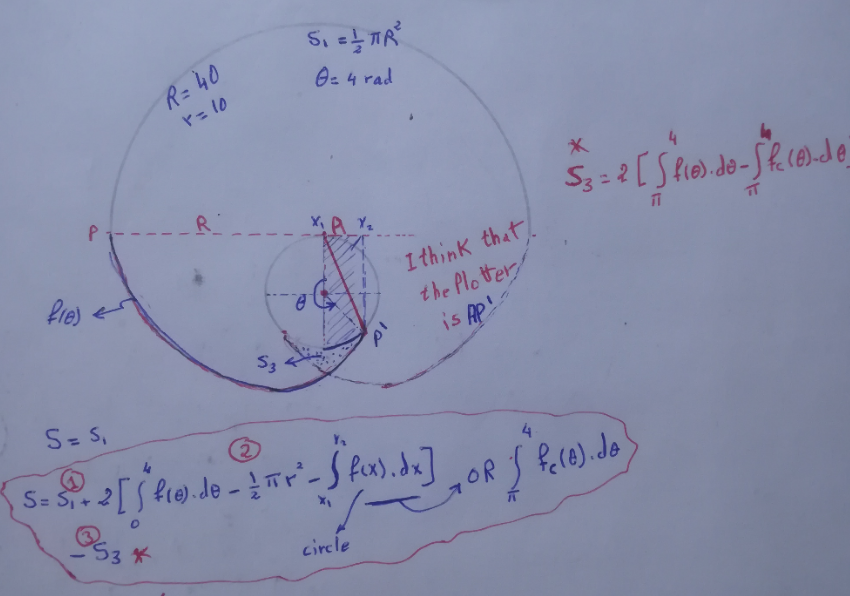
$${Deem}\:{that}\:{the}\:{cilo}\:{as}\:{a}\:{wheel}\:{is}\:{spinning}\:{without} \\ $$$$\:{rolling},\:{and}\:{here}\:{i}\:{think}\:{that}\:{the}\:{plotter}\:{is}\:{the} \\ $$$$\:{segment}\:{AP}\:' \\ $$$$\:{Now}\:{we}\:{need}\:{a}\:{function}\:{in}\:{terms} \\ $$$$\:{of}\:{the}\:{variable}\:\theta \\ $$$$\boldsymbol{{Important}}\:\boldsymbol{{Note}},\:{i}\:{didn}'{t}\:{notice}\:{the}\:{fixed}\:{point} \\ $$$$\:{which}\:{has}\:\mathrm{2}{m}\:{height},\:{i}\:{will}\:{modify}\:{the}\:{graph}\: \\ $$$$\:{and}\:{some}\:{values}\:{later} \\ $$$$ \\ $$
Answered by mahdipoor last updated on 28/Nov/22

$$ \\ $$
Commented by mahdipoor last updated on 28/Nov/22
$${i}\:{cant}\:{send}\:{image}\::\left(\right. \\ $$
Commented by mahdipoor last updated on 28/Nov/22
![so F.S. is: π[((f^2 ((π/2)))/2)−R^2 +2∣∫_θ_1 ^( θ_2 ) g(θ)dθ∣] ⇒⇒ f(θ)=(√(L^2 −H^2 ))−R((π/2)−θ) g(θ)=(R^2 +f^2 (θ))(−1+(d/dθ)[tan^(−1) (((f(θ))/R))]) { ((θ_2 =(π/2))),((0>θ_1 ≥((−π)/2)=Answer {Rcos(θ)+f(θ)sinθ=0})) :} H=2m R=10m L=40m](https://www.tinkutara.com/question/Q181695.png)
$${so}\:{F}.{S}.\:{is}: \\ $$$$\pi\left[\frac{{f}^{\mathrm{2}} \left(\frac{\pi}{\mathrm{2}}\right)}{\mathrm{2}}−{R}^{\mathrm{2}} +\mathrm{2}\mid\int_{\theta_{\mathrm{1}} } ^{\:\theta_{\mathrm{2}} } {g}\left(\theta\right){d}\theta\mid\right] \\ $$$$\Rightarrow\Rightarrow \\ $$$${f}\left(\theta\right)=\sqrt{{L}^{\mathrm{2}} −{H}^{\mathrm{2}} }−{R}\left(\frac{\pi}{\mathrm{2}}−\theta\right) \\ $$$${g}\left(\theta\right)=\left({R}^{\mathrm{2}} +{f}^{\mathrm{2}} \left(\theta\right)\right)\left(−\mathrm{1}+\frac{{d}}{{d}\theta}\left[{tan}^{−\mathrm{1}} \left(\frac{{f}\left(\theta\right)}{{R}}\right)\right]\right) \\ $$$$\begin{cases}{\theta_{\mathrm{2}} =\frac{\pi}{\mathrm{2}}}\\{\mathrm{0}>\theta_{\mathrm{1}} \geqslant\frac{−\pi}{\mathrm{2}}={Answer}\:\left\{{Rcos}\left(\theta\right)+{f}\left(\theta\right){sin}\theta=\mathrm{0}\right\}}\end{cases} \\ $$$${H}=\mathrm{2}{m}\:\:\:\:\:{R}=\mathrm{10}{m}\:\:\:\:\:\:{L}=\mathrm{40}{m} \\ $$
Answered by mr W last updated on 28/Nov/22

Commented by mr W last updated on 28/Nov/22
![l=rope length r=radius of silo h=height of anchor point of rope R=(√(l^2 −h^2 )) let λ=(R/r) PB=a (a+rθ)^2 +h^2 =l^2 ⇒a=(√(l^2 −h^2 ))−rθ=R−rθ x_P =r cos θ−a sin θ =r cos θ−(R−rθ) sin θ =r[cos θ−(λ−θ) sin θ] y_P =r sin θ+a cos θ =r sin θ+(R−rθ) cos θ =r[sin θ+(λ−θ) cos θ] at y_P =0: θ_1 −tan θ_1 =λ A_1 =2∫_0 ^R (r−x)dy A_1 =2r^2 ∫_θ_1 ^0 [1−cos θ+(λ−θ) sin θ][−(λ−θ) sin θ]dθ A_1 =2r^2 ∫_0 ^θ_1 (λ−θ) sin θ [1−cos θ+(λ−θ) sin θ]dθ A=((πR^2 )/2)+A_1 −πr^2 ⇒A=πr^2 {(λ^2 /2)−1+(2/π)∫_0 ^θ_1 (λ−θ) sin θ [1 +(λ−θ) sin θ−cos θ]dθ} example: r=10, l=40, h=2 λ=((√(l^2 −h^2 ))/r)=((√(40^2 −2^2 ))/(10))=((√(399))/5) θ_1 ≈2.0446 A≈π×10^2 ×(((399)/(50))−1+7.249688150) ≈4470.4 m^2](https://www.tinkutara.com/question/Q181697.png)
$${l}={rope}\:{length} \\ $$$${r}={radius}\:{of}\:{silo} \\ $$$${h}={height}\:{of}\:{anchor}\:{point}\:{of}\:{rope} \\ $$$${R}=\sqrt{{l}^{\mathrm{2}} −{h}^{\mathrm{2}} } \\ $$$${let}\:\lambda=\frac{{R}}{{r}} \\ $$$${PB}={a} \\ $$$$\left({a}+{r}\theta\right)^{\mathrm{2}} +{h}^{\mathrm{2}} ={l}^{\mathrm{2}} \\ $$$$\Rightarrow{a}=\sqrt{{l}^{\mathrm{2}} −{h}^{\mathrm{2}} }−{r}\theta={R}−{r}\theta \\ $$$${x}_{{P}} ={r}\:\mathrm{cos}\:\theta−{a}\:\mathrm{sin}\:\theta \\ $$$$\:\:\:\:\:={r}\:\mathrm{cos}\:\theta−\left({R}−{r}\theta\right)\:\mathrm{sin}\:\theta \\ $$$$\:\:\:\:\:={r}\left[\mathrm{cos}\:\theta−\left(\lambda−\theta\right)\:\mathrm{sin}\:\theta\right] \\ $$$${y}_{{P}} ={r}\:\mathrm{sin}\:\theta+{a}\:\mathrm{cos}\:\theta \\ $$$$\:\:\:\:\:={r}\:\mathrm{sin}\:\theta+\left({R}−{r}\theta\right)\:\mathrm{cos}\:\theta \\ $$$$\:\:\:\:\:={r}\left[\mathrm{sin}\:\theta+\left(\lambda−\theta\right)\:\mathrm{cos}\:\theta\right] \\ $$$${at}\:{y}_{{P}} =\mathrm{0}: \\ $$$$\theta_{\mathrm{1}} −\mathrm{tan}\:\theta_{\mathrm{1}} =\lambda \\ $$$$ \\ $$$${A}_{\mathrm{1}} =\mathrm{2}\int_{\mathrm{0}} ^{{R}} \left({r}−{x}\right){dy} \\ $$$${A}_{\mathrm{1}} =\mathrm{2}{r}^{\mathrm{2}} \int_{\theta_{\mathrm{1}} } ^{\mathrm{0}} \left[\mathrm{1}−\mathrm{cos}\:\theta+\left(\lambda−\theta\right)\:\mathrm{sin}\:\theta\right]\left[−\left(\lambda−\theta\right)\:\mathrm{sin}\:\theta\right]{d}\theta \\ $$$${A}_{\mathrm{1}} =\mathrm{2}{r}^{\mathrm{2}} \int_{\mathrm{0}} ^{\theta_{\mathrm{1}} } \left(\lambda−\theta\right)\:\mathrm{sin}\:\theta\:\left[\mathrm{1}−\mathrm{cos}\:\theta+\left(\lambda−\theta\right)\:\mathrm{sin}\:\theta\right]{d}\theta \\ $$$${A}=\frac{\pi{R}^{\mathrm{2}} }{\mathrm{2}}+{A}_{\mathrm{1}} −\pi{r}^{\mathrm{2}} \\ $$$$\Rightarrow{A}=\pi{r}^{\mathrm{2}} \left\{\frac{\lambda^{\mathrm{2}} }{\mathrm{2}}−\mathrm{1}+\frac{\mathrm{2}}{\pi}\int_{\mathrm{0}} ^{\theta_{\mathrm{1}} } \left(\lambda−\theta\right)\:\mathrm{sin}\:\theta\:\left[\mathrm{1}\:+\left(\lambda−\theta\right)\:\mathrm{sin}\:\theta−\mathrm{cos}\:\theta\right]{d}\theta\right\} \\ $$$${example}:\:{r}=\mathrm{10},\:{l}=\mathrm{40},\:{h}=\mathrm{2} \\ $$$$\lambda=\frac{\sqrt{{l}^{\mathrm{2}} −{h}^{\mathrm{2}} }}{{r}}=\frac{\sqrt{\mathrm{40}^{\mathrm{2}} −\mathrm{2}^{\mathrm{2}} }}{\mathrm{10}}=\frac{\sqrt{\mathrm{399}}}{\mathrm{5}} \\ $$$$\theta_{\mathrm{1}} \approx\mathrm{2}.\mathrm{0446} \\ $$$${A}\approx\pi×\mathrm{10}^{\mathrm{2}} ×\left(\frac{\mathrm{399}}{\mathrm{50}}−\mathrm{1}+\mathrm{7}.\mathrm{249688150}\right) \\ $$$$\:\:\:\approx\mathrm{4470}.\mathrm{4}\:{m}^{\mathrm{2}} \\ $$
Commented by mr W last updated on 28/Nov/22