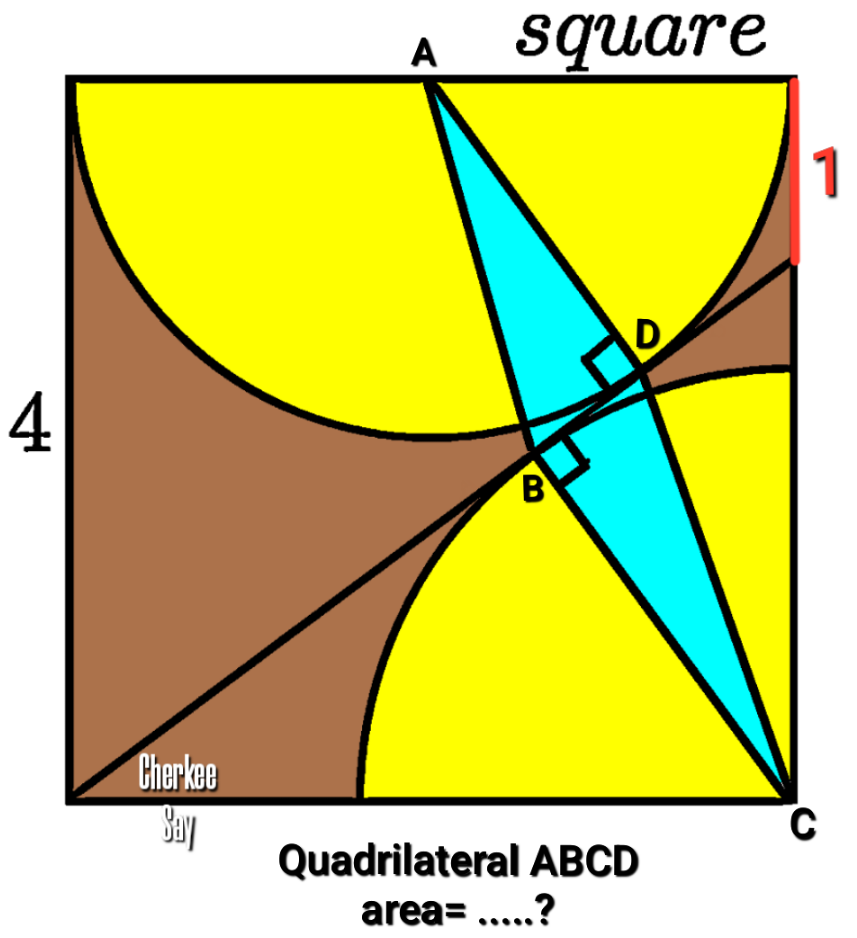
Question Number 181673 by cherokeesay last updated on 28/Nov/22
Answered by HeferH last updated on 28/Nov/22
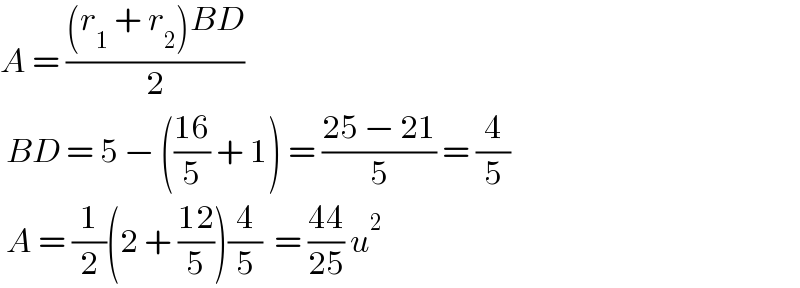
Commented by cherokeesay last updated on 29/Nov/22

Answered by a.lgnaoui last updated on 29/Nov/22

Answered by a.lgnaoui last updated on 29/Nov/22
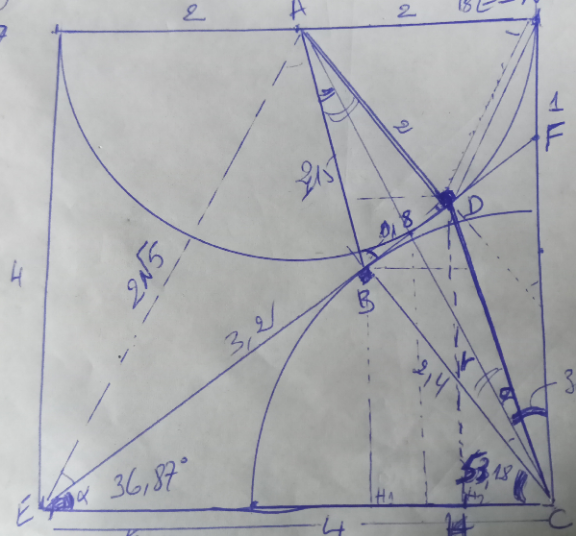
Answered by a.lgnaoui last updated on 29/Nov/22
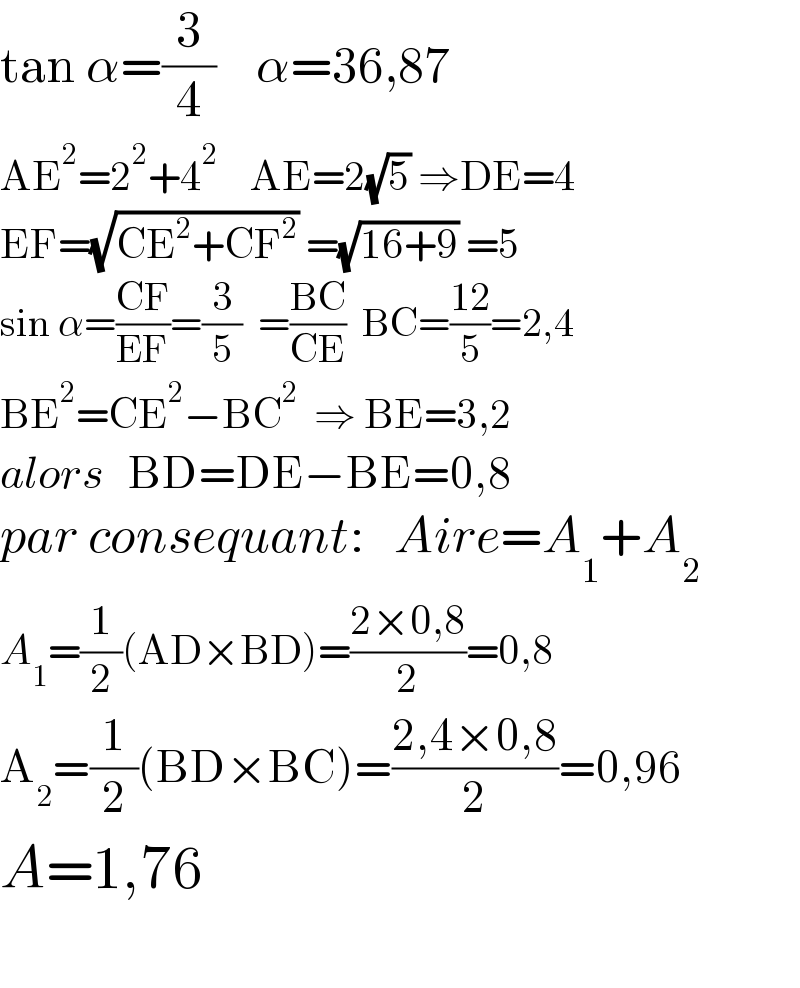
Commented by cherokeesay last updated on 29/Nov/22