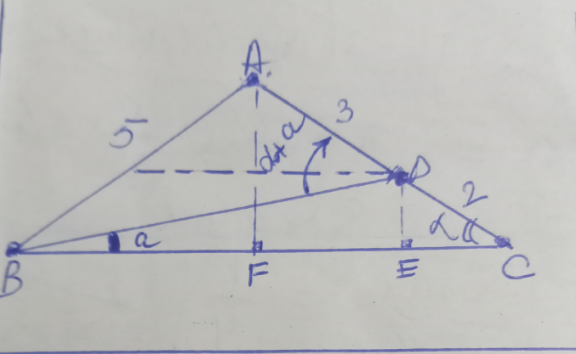
Question Number 181839 by amin96 last updated on 01/Dec/22
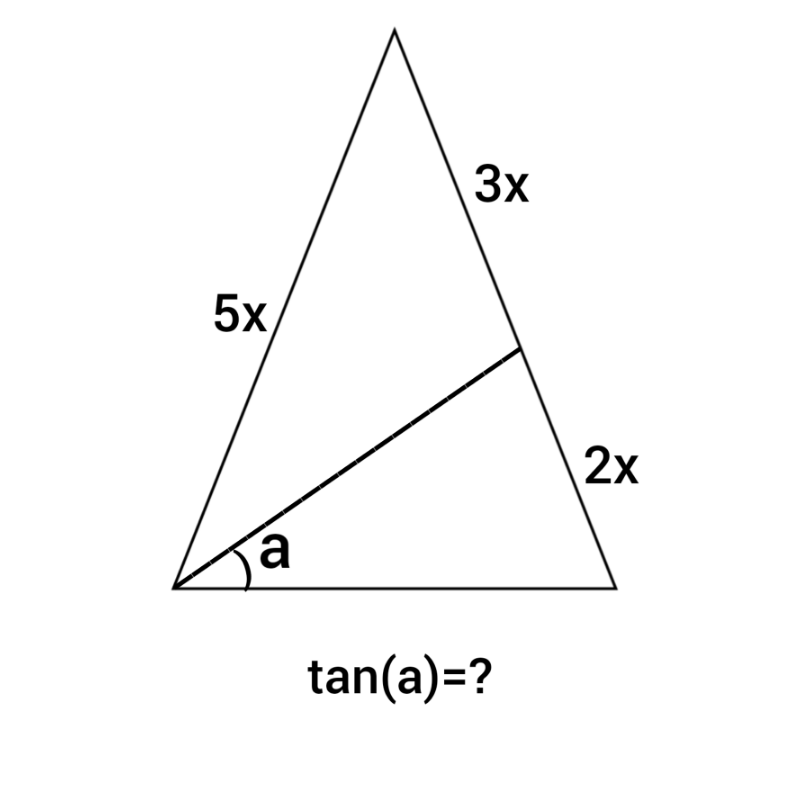
Commented by mr W last updated on 01/Dec/22
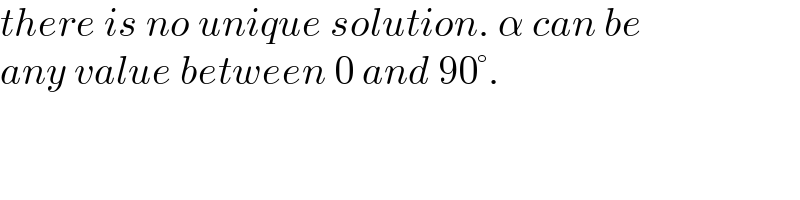
$${there}\:{is}\:{no}\:{unique}\:{solution}.\:\alpha\:{can}\:{be} \\ $$$${any}\:{value}\:{between}\:\mathrm{0}\:{and}\:\mathrm{90}°. \\ $$
Commented by Acem last updated on 02/Dec/22
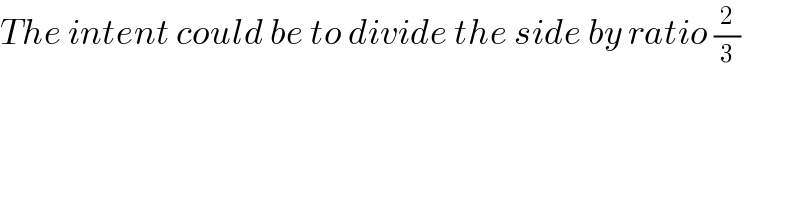
$${The}\:{intent}\:{could}\:{be}\:{to}\:{divide}\:{the}\:{side}\:{by}\:{ratio}\:\frac{\mathrm{2}}{\mathrm{3}} \\ $$
Commented by mr W last updated on 02/Dec/22
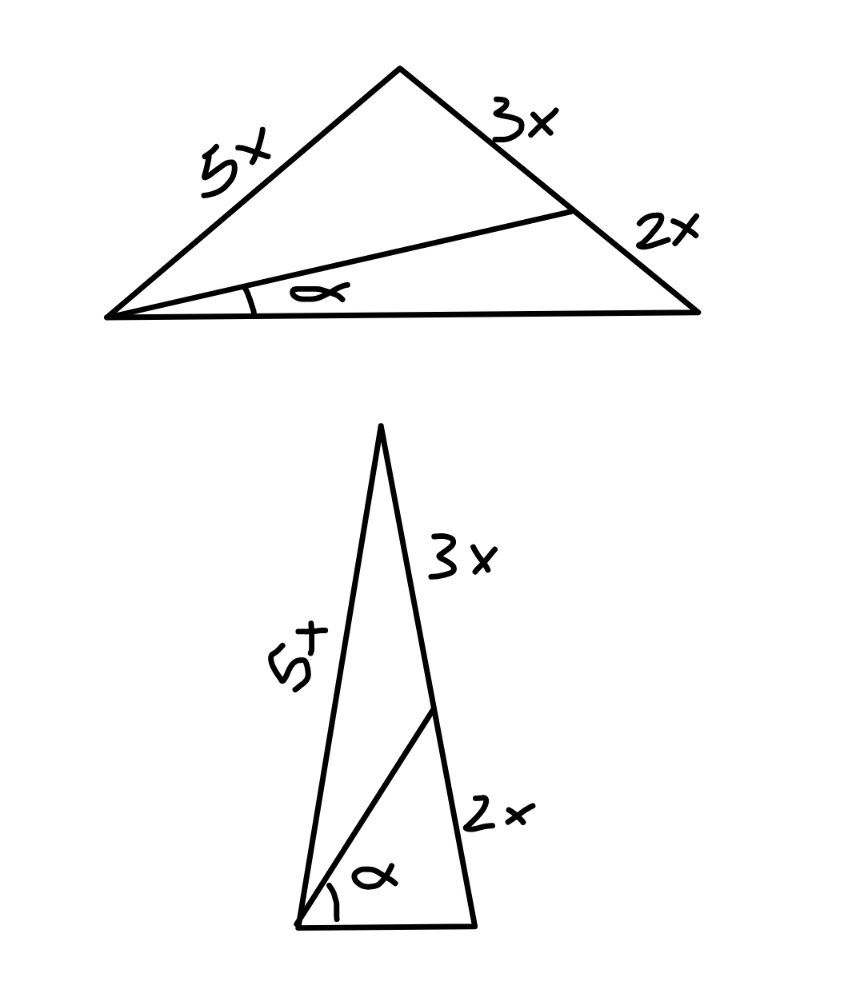
Commented by mr W last updated on 02/Dec/22
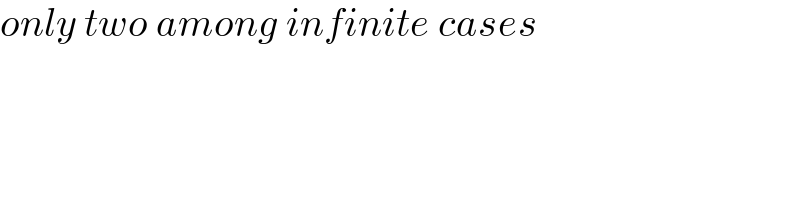
$${only}\:{two}\:{among}\:{infinite}\:{cases} \\ $$
Commented by Acem last updated on 02/Dec/22

$${You}'{re}\:{right}! \\ $$
Answered by a.lgnaoui last updated on 02/Dec/22
$$\mathrm{Triangle}\:\mathrm{ABD}\: \\ $$$$\:\frac{\mathrm{sin}\:\measuredangle\mathrm{ABD}}{\mathrm{3}}=\frac{\mathrm{sin}\:\measuredangle\mathrm{ADB}}{\mathrm{5}}=\frac{\mathrm{sin}\:\measuredangle\mathrm{BAD}}{\mathrm{BD}} \\ $$$$\frac{\mathrm{sin}\:\left(\alpha−{a}\right)}{\mathrm{3}}=\frac{\mathrm{sin}\left(\alpha+{a}\right)}{\mathrm{5}} \\ $$$$\mathrm{5sin}\:\left(\alpha−{a}\right)=\mathrm{3sin}\:\left(\alpha+{a}\right) \\ $$$$\mathrm{5}\left(\mathrm{sin}\:\alpha\mathrm{cos}\:{a}−\mathrm{cos}\:\alpha\mathrm{sin}\:{a}\right)=\mathrm{3}\left(\mathrm{sin}\:\alpha\mathrm{cos}\:{a}+\mathrm{cos}\:\alpha\mathrm{sin}\:{a}\right) \\ $$$$\mathrm{2sin}\:\alpha\mathrm{cos}\:{a}=\mathrm{8cos}\:\alpha\mathrm{sin}\:{a} \\ $$$$\frac{\mathrm{1}}{\mathrm{4}}=\mathrm{tan}\:{a}×\mathrm{cot}\:\alpha=\frac{\mathrm{tan}\:{a}}{\mathrm{tan}\:\alpha}\:\:\:\:\:\left(\mathrm{1}\right) \\ $$$$\mathrm{Triangle}\:\mathrm{ABC} \\ $$$$\mathrm{BC}^{\mathrm{2}} =\mathrm{AB}^{\mathrm{2}} +\mathrm{AC}^{\mathrm{2}} −\mathrm{2AB}×\mathrm{ACcos}\:\mathrm{2}\alpha \\ $$$$\:\:\:\:\mathrm{BC}^{\mathrm{2}} \:\:\:\:=\mathrm{50}−\mathrm{50cos}\:\mathrm{2}\alpha\:\:\:\:\left(\mathrm{2}\right) \\ $$$$\mathrm{tan}\:\alpha=\frac{\mathrm{AF}}{\mathrm{BF}}=\frac{\mathrm{2}×\mathrm{AF}}{\mathrm{BC}}\:\:\:\:\mathrm{AF}=\mathrm{5sin}\:\alpha \\ $$$$\mathrm{tan}\:\alpha=\frac{\mathrm{10sin}\:\alpha}{\mathrm{BC}} \\ $$$$\mathrm{5}^{\mathrm{2}} =\mathrm{AF}^{\mathrm{2}} +\frac{\mathrm{BC}^{\mathrm{2}} }{\mathrm{4}}=\mathrm{5}^{\mathrm{2}} \mathrm{sin}^{\mathrm{2}} \alpha+\:\mathrm{50}\left(\mathrm{1}−\mathrm{cos}\:\mathrm{2}\alpha\right) \\ $$$$\mathrm{sin}\:\alpha=\frac{\mathrm{AF}}{\mathrm{5}}\Rightarrow\:\:\:\mathrm{AF}=\mathrm{5sin}\:\alpha \\ $$$$\mathrm{25}=\mathrm{25sin}\:^{\mathrm{2}} \alpha+\frac{\mathrm{50}}{\mathrm{4}}\left(\mathrm{1}−\mathrm{cos}\:\mathrm{2}\alpha\right) \\ $$$$\mathrm{1}=\mathrm{sin}\:^{\mathrm{2}} \alpha+\frac{\mathrm{1}}{\mathrm{2}}\left(\mathrm{1}−\mathrm{cos}\:^{\mathrm{2}} \alpha+\mathrm{sin}^{\mathrm{2}} \alpha\right)\: \\ $$$$\mathrm{1}=\mathrm{2sin}\:^{\mathrm{2}} \alpha+\frac{\mathrm{1}}{\mathrm{2}}\Rightarrow\mathrm{sin}\:\alpha=\frac{\mathrm{1}}{\mathrm{2}}\:\left(\alpha=\frac{\pi}{\mathrm{6}}\right) \\ $$$$\mathrm{tan}\:{a}=\frac{\mathrm{tan}\:\alpha}{\mathrm{4}}\:=\frac{\mathrm{1}}{\mathrm{4}\sqrt{\mathrm{3}}}=\frac{\sqrt{\mathrm{3}}}{\mathrm{12}} \\ $$$$\:\:{Angle}\:{a}=\mathrm{8},\mathrm{21}^{°} \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$
Commented by a.lgnaoui last updated on 02/Dec/22
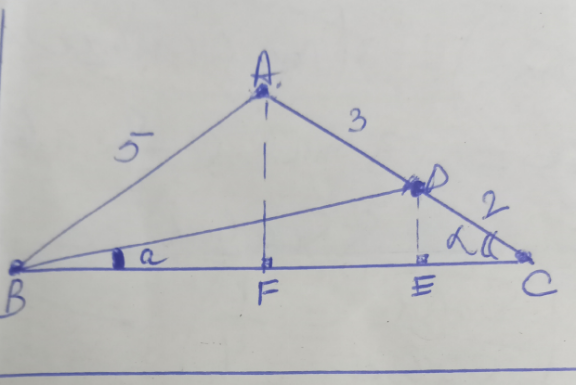
Commented by a.lgnaoui last updated on 02/Dec/22