Question Number 182407 by Acem last updated on 09/Dec/22

Answered by a.lgnaoui last updated on 09/Dec/22
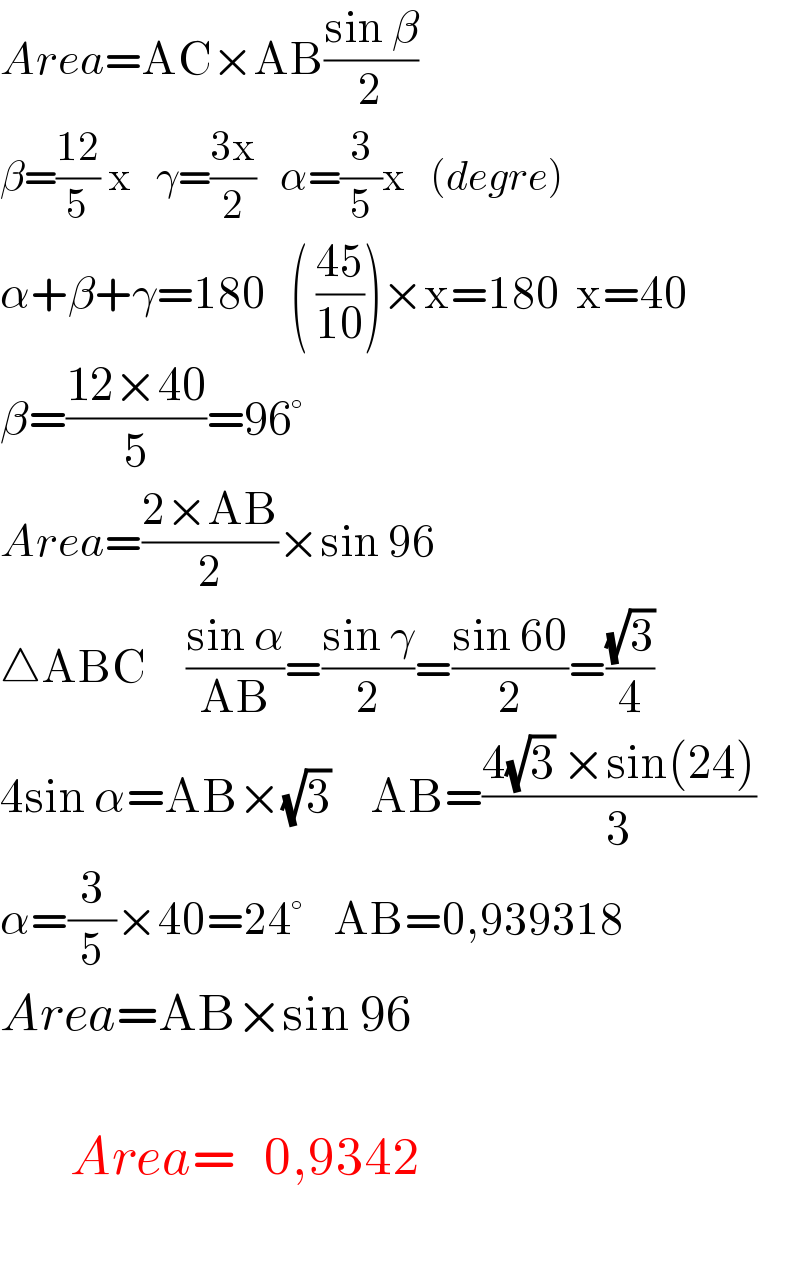
$${Area}=\mathrm{AC}×\mathrm{AB}\frac{\mathrm{sin}\:\beta}{\mathrm{2}} \\ $$$$\beta=\frac{\mathrm{12}}{\mathrm{5}}\:\mathrm{x}\:\:\:\gamma=\frac{\mathrm{3x}}{\mathrm{2}}\:\:\:\alpha=\frac{\mathrm{3}}{\mathrm{5}}\mathrm{x}\:\:\:\left({degre}\right) \\ $$$$\alpha+\beta+\gamma=\mathrm{180}\:\:\:\left(\:\frac{\mathrm{45}}{\mathrm{10}}\right)×\mathrm{x}=\mathrm{180}\:\:\mathrm{x}=\mathrm{40} \\ $$$$\beta=\frac{\mathrm{12}×\mathrm{40}}{\mathrm{5}}=\mathrm{96}° \\ $$$${Area}=\frac{\mathrm{2}×\mathrm{AB}}{\mathrm{2}}×\mathrm{sin}\:\mathrm{96} \\ $$$$\bigtriangleup\mathrm{ABC}\:\:\:\:\:\frac{\mathrm{sin}\:\alpha}{\mathrm{AB}}=\frac{\mathrm{sin}\:\gamma}{\mathrm{2}}=\frac{\mathrm{sin}\:\mathrm{60}}{\mathrm{2}}=\frac{\sqrt{\mathrm{3}}}{\mathrm{4}} \\ $$$$\mathrm{4sin}\:\alpha=\mathrm{AB}×\sqrt{\mathrm{3}}\:\:\:\:\:\mathrm{AB}=\frac{\mathrm{4}\sqrt{\mathrm{3}}\:×\mathrm{sin}\left(\mathrm{24}\right)}{\mathrm{3}} \\ $$$$\alpha=\frac{\mathrm{3}}{\mathrm{5}}×\mathrm{40}=\mathrm{24}°\:\:\:\:\mathrm{AB}=\mathrm{0},\mathrm{939318} \\ $$$${Area}=\mathrm{AB}×\mathrm{sin}\:\mathrm{96} \\ $$$$ \\ $$$$\:\:\:\:\:\:\:{Area}=\:\:\:\mathrm{0},\mathrm{9342} \\ $$$$ \\ $$
Commented by Acem last updated on 10/Dec/22

$${Tous}\:{merci}\:{et}\:{respect}! \\ $$
