Menu Close

Question-182436

Tinku Tara 0 Comments
Pin




Question Number 182436 by akolade last updated on 09/Dec/22
Answered by Rasheed.Sindhi last updated on 09/Dec/22
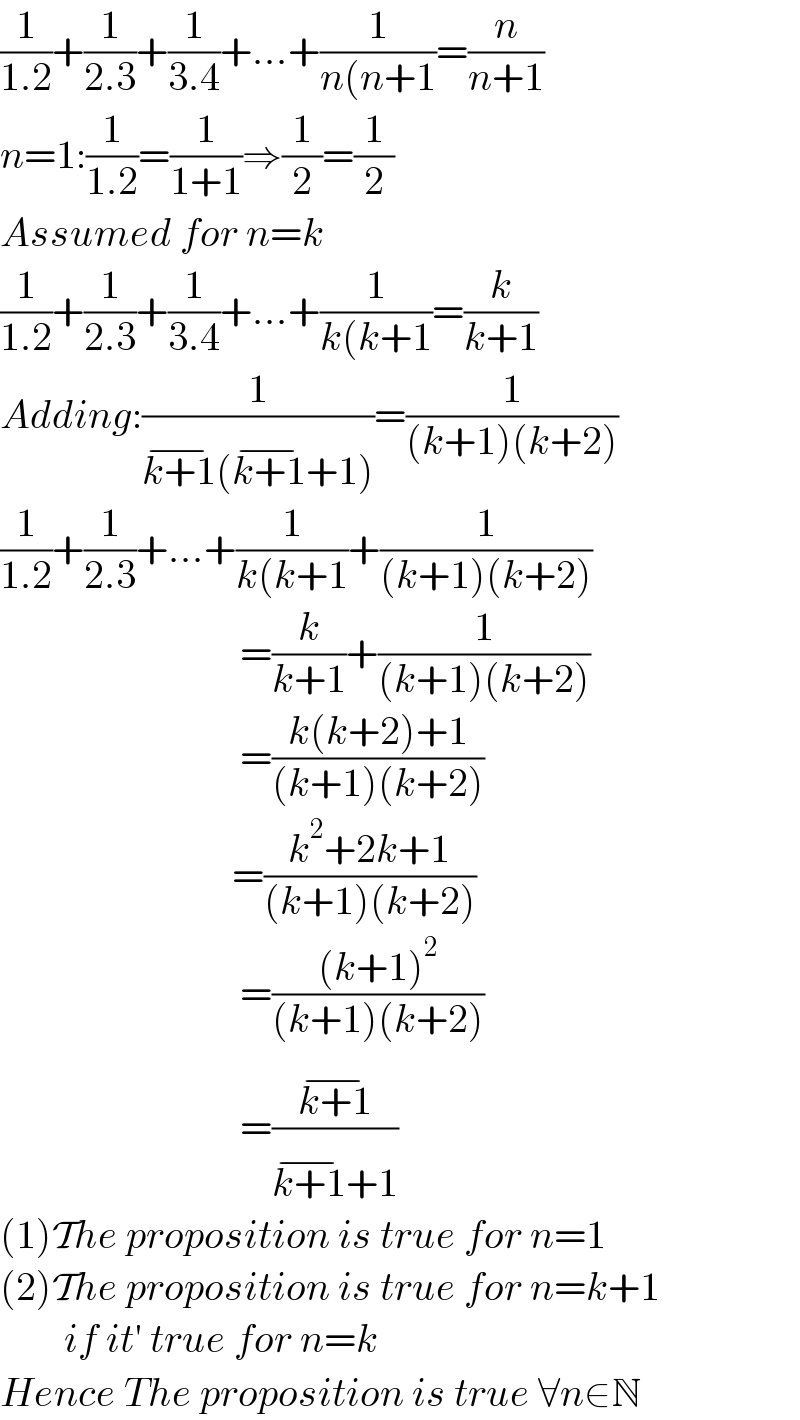
(1/(1.2))+(1/(2.3))+(1/(3.4))+...+(1/(n(n+1))=(n/(n+1)) n=1:(1/(1.2))=(1/(1+1))⇒(1/2)=(1/2) Assumed for n=k (1/(1.2))+(1/(2.3))+(1/(3.4))+...+(1/(k(k+1))=(k/(k+1)) Adding:(1/(k+1^(−) (k+1^(−) +1)))=(1/((k+1)(k+2))) (1/(1.2))+(1/(2.3))+...+(1/(k(k+1))+(1/((k+1)(k+2))) =(k/(k+1))+(1/((k+1)(k+2))) =((k(k+2)+1)/((k+1)(k+2))) =((k^2 +2k+1)/((k+1)(k+2))) =(((k+1)^2 )/((k+1)(k+2))) =((k+1^(−) )/(k+1^(−) +1)) (1)The proposition is true for n=1 (2)The proposition is true for n=k+1 if it′ true for n=k Hence The proposition is true ∀n∈N
$$\frac{\mathrm{1}}{\mathrm{1}.\mathrm{2}}+\frac{\mathrm{1}}{\mathrm{2}.\mathrm{3}}+\frac{\mathrm{1}}{\mathrm{3}.\mathrm{4}}+…+\frac{\mathrm{1}}{{n}\left({n}+\mathrm{1}\right.}=\frac{{n}}{{n}+\mathrm{1}} \\ $$$${n}=\mathrm{1}:\frac{\mathrm{1}}{\mathrm{1}.\mathrm{2}}=\frac{\mathrm{1}}{\mathrm{1}+\mathrm{1}}\Rightarrow\frac{\mathrm{1}}{\mathrm{2}}=\frac{\mathrm{1}}{\mathrm{2}} \\ $$$${Assumed}\:{for}\:{n}={k} \\ $$$$\frac{\mathrm{1}}{\mathrm{1}.\mathrm{2}}+\frac{\mathrm{1}}{\mathrm{2}.\mathrm{3}}+\frac{\mathrm{1}}{\mathrm{3}.\mathrm{4}}+…+\frac{\mathrm{1}}{{k}\left({k}+\mathrm{1}\right.}=\frac{{k}}{{k}+\mathrm{1}} \\ $$$${Adding}:\frac{\mathrm{1}}{\overline {{k}+\mathrm{1}}\left(\overline {{k}+\mathrm{1}}+\mathrm{1}\right)}=\frac{\mathrm{1}}{\left({k}+\mathrm{1}\right)\left({k}+\mathrm{2}\right)} \\ $$$$\frac{\mathrm{1}}{\mathrm{1}.\mathrm{2}}+\frac{\mathrm{1}}{\mathrm{2}.\mathrm{3}}+…+\frac{\mathrm{1}}{{k}\left({k}+\mathrm{1}\right.}+\frac{\mathrm{1}}{\left({k}+\mathrm{1}\right)\left({k}+\mathrm{2}\right)} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\frac{{k}}{{k}+\mathrm{1}}+\frac{\mathrm{1}}{\left({k}+\mathrm{1}\right)\left({k}+\mathrm{2}\right)} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\frac{{k}\left({k}+\mathrm{2}\right)+\mathrm{1}}{\left({k}+\mathrm{1}\right)\left({k}+\mathrm{2}\right)} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\frac{{k}^{\mathrm{2}} +\mathrm{2}{k}+\mathrm{1}}{\left({k}+\mathrm{1}\right)\left({k}+\mathrm{2}\right)} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\frac{\left({k}+\mathrm{1}\right)^{\mathrm{2}} }{\left({k}+\mathrm{1}\right)\left({k}+\mathrm{2}\right)} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\frac{\overline {{k}+\mathrm{1}}}{\overline {{k}+\mathrm{1}}+\mathrm{1}} \\ $$$$\left(\mathrm{1}\right)\mathcal{T}{he}\:{proposition}\:{is}\:{true}\:{for}\:{n}=\mathrm{1} \\ $$$$\left(\mathrm{2}\right)\mathcal{T}{he}\:{proposition}\:{is}\:{true}\:{for}\:{n}={k}+\mathrm{1} \\ $$$$\:\:\:\:\:\:\:\:{if}\:{it}'\:{true}\:{for}\:{n}={k} \\ $$$${Hence}\:{The}\:{proposition}\:{is}\:{true}\:\forall{n}\in\mathbb{N} \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *