Question Number 183307 by ajfour last updated on 24/Dec/22
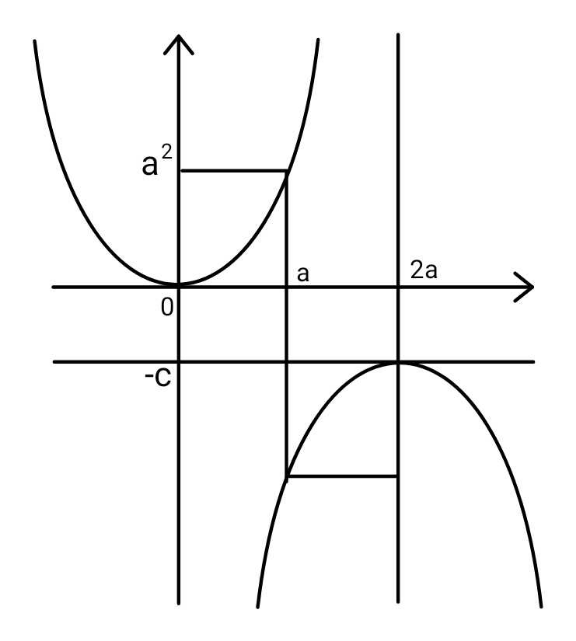
Commented by ajfour last updated on 24/Dec/22
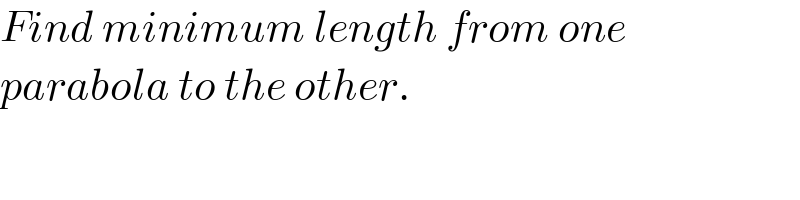
$${Find}\:{minimum}\:{length}\:{from}\:{one} \\ $$$${parabola}\:{to}\:{the}\:{other}. \\ $$
Answered by mr W last updated on 24/Dec/22

Commented by mr W last updated on 25/Dec/22
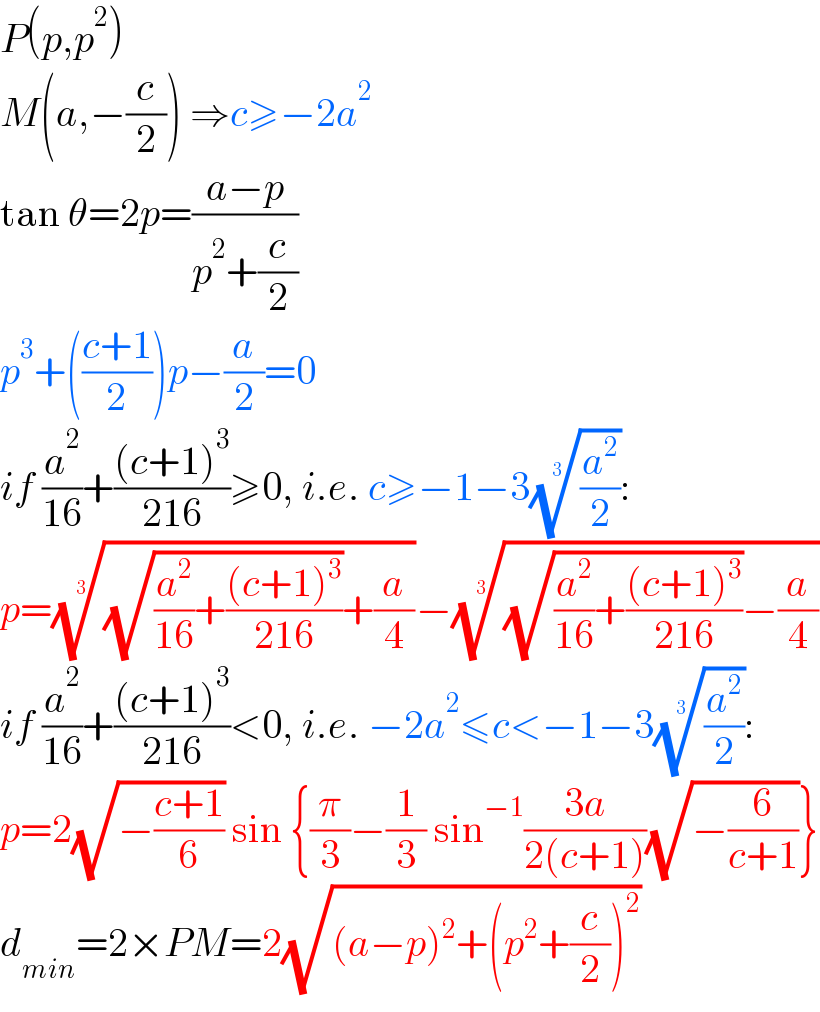
$${P}\left({p},{p}^{\mathrm{2}} \right) \\ $$$${M}\left({a},−\frac{{c}}{\mathrm{2}}\right)\:\Rightarrow{c}\geqslant−\mathrm{2}{a}^{\mathrm{2}} \\ $$$$\mathrm{tan}\:\theta=\mathrm{2}{p}=\frac{{a}−{p}}{{p}^{\mathrm{2}} +\frac{{c}}{\mathrm{2}}} \\ $$$${p}^{\mathrm{3}} +\left(\frac{{c}+\mathrm{1}}{\mathrm{2}}\right){p}−\frac{{a}}{\mathrm{2}}=\mathrm{0} \\ $$$${if}\:\frac{{a}^{\mathrm{2}} }{\mathrm{16}}+\frac{\left({c}+\mathrm{1}\right)^{\mathrm{3}} }{\mathrm{216}}\geqslant\mathrm{0},\:{i}.{e}.\:{c}\geqslant−\mathrm{1}−\mathrm{3}\sqrt[{\mathrm{3}}]{\frac{{a}^{\mathrm{2}} }{\mathrm{2}}}: \\ $$$${p}=\sqrt[{\mathrm{3}}]{\sqrt{\frac{{a}^{\mathrm{2}} }{\mathrm{16}}+\frac{\left({c}+\mathrm{1}\right)^{\mathrm{3}} }{\mathrm{216}}}+\frac{{a}}{\mathrm{4}}}−\sqrt[{\mathrm{3}}]{\sqrt{\frac{{a}^{\mathrm{2}} }{\mathrm{16}}+\frac{\left({c}+\mathrm{1}\right)^{\mathrm{3}} }{\mathrm{216}}}−\frac{{a}}{\mathrm{4}}} \\ $$$${if}\:\frac{{a}^{\mathrm{2}} }{\mathrm{16}}+\frac{\left({c}+\mathrm{1}\right)^{\mathrm{3}} }{\mathrm{216}}<\mathrm{0},\:{i}.{e}.\:−\mathrm{2}{a}^{\mathrm{2}} \leqslant{c}<−\mathrm{1}−\mathrm{3}\sqrt[{\mathrm{3}}]{\frac{{a}^{\mathrm{2}} }{\mathrm{2}}}: \\ $$$${p}=\mathrm{2}\sqrt{−\frac{{c}+\mathrm{1}}{\mathrm{6}}}\:\mathrm{sin}\:\left\{\frac{\pi}{\mathrm{3}}−\frac{\mathrm{1}}{\mathrm{3}}\:\mathrm{sin}^{−\mathrm{1}} \frac{\mathrm{3}{a}}{\mathrm{2}\left({c}+\mathrm{1}\right)}\sqrt{−\frac{\mathrm{6}}{{c}+\mathrm{1}}}\right\} \\ $$$${d}_{{min}} =\mathrm{2}×{PM}=\mathrm{2}\sqrt{\left({a}−{p}\right)^{\mathrm{2}} +\left({p}^{\mathrm{2}} +\frac{{c}}{\mathrm{2}}\right)^{\mathrm{2}} } \\ $$
Commented by mr W last updated on 24/Dec/22

Commented by mr W last updated on 24/Dec/22

Commented by mr W last updated on 25/Dec/22

Commented by mr W last updated on 25/Dec/22

Commented by ajfour last updated on 25/Dec/22
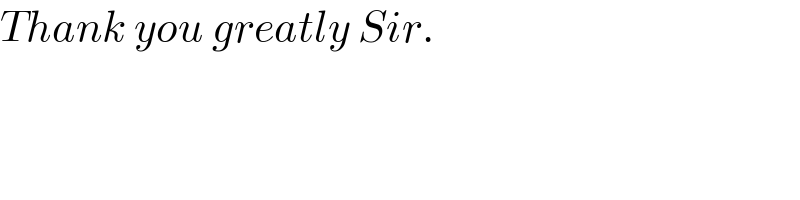
$${Thank}\:{you}\:{greatly}\:{Sir}.\: \\ $$
