Question-184441

Question Number 184441 by ajfour last updated on 06/Jan/23

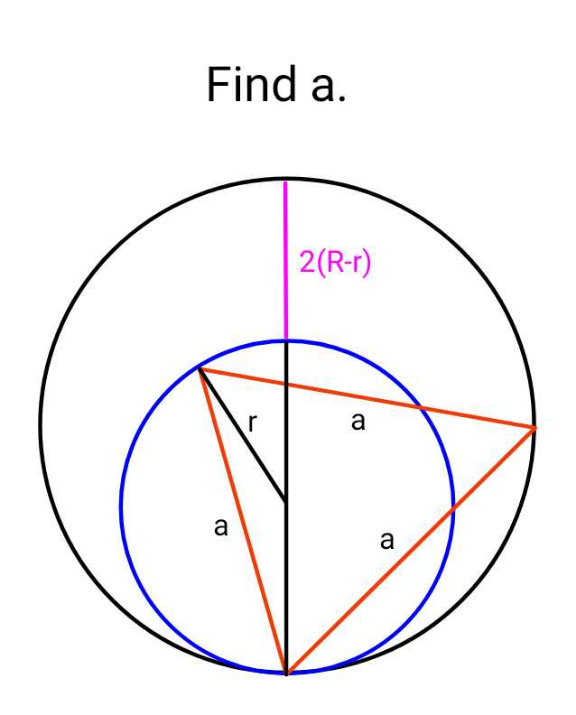
Commented by mr W last updated on 07/Jan/23

a = \frac{\sqrt{3} R r}{\sqrt{R^{2} + r^{2} - R r}}

Answered by ajfour last updated on 07/Jan/23

a = 2 r \cos θ = 2 R \cos ϕ

θ + ϕ = \frac{π}{3}

\Rightarrow {(\cos θ \cos ϕ - \frac{1}{2})}^{2}

= (1 - \cos^{2} θ) (1 - \cos^{2} ϕ)

\Rightarrow {(\frac{a^{2}}{4 r R} - \frac{1}{2})}^{2} = (1 - \frac{a^{2}}{4 r^{2}}) (1 - \frac{a^{2}}{4 R^{2}})

\Rightarrow \frac{a^{2}}{4} (\frac{1}{r^{2}} + \frac{1}{R^{2}} - \frac{1}{r R}) = \frac{3}{4}

\Rightarrow a^{2} = \frac{3 r^{2} R^{2}}{r^{2} + R^{2} - r R}

\Rightarrow a = \frac{\sqrt{3} r R}{\sqrt{r^{2} + R^{2} - r R}}

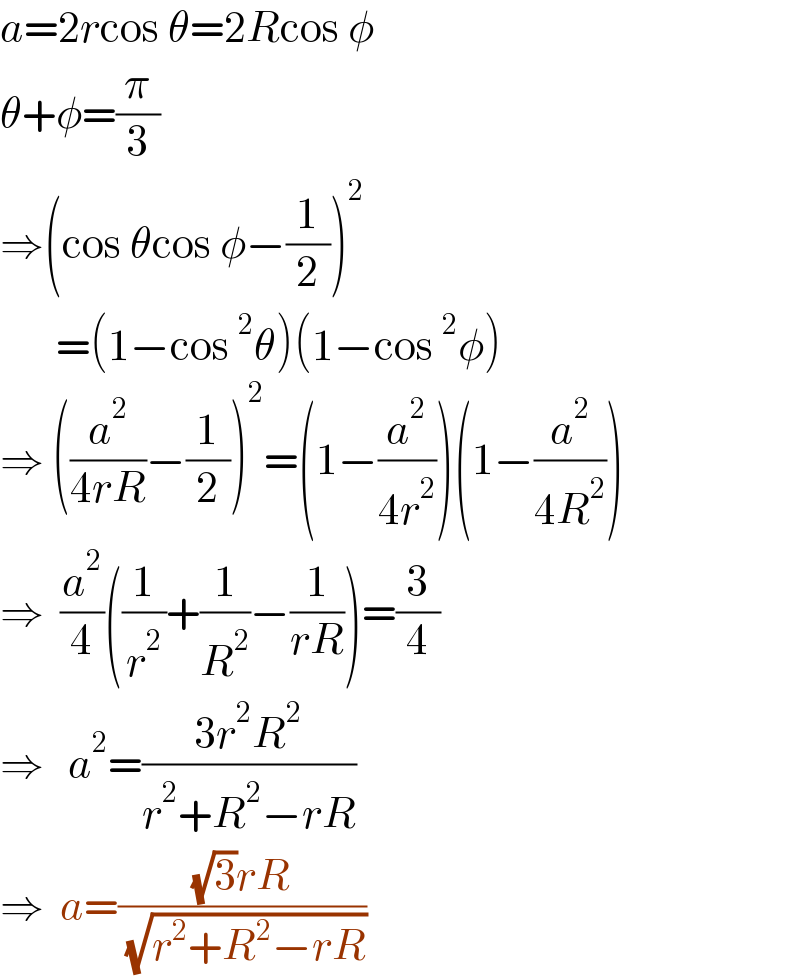
Commented by mr W last updated on 07/Jan/23

��

Answered by mr W last updated on 07/Jan/23

\cos α = \frac{a}{2 r}

\cos β = \frac{a}{2 R}

α + β = \frac{π}{3}

\cos (α + β) = \frac{a^{2}}{4 R r} - \frac{\sqrt{(4 R^{2} - a^{2}) (4 r^{2} - a^{2})}}{4 R r} = \frac{1}{2}

a^{2} - 2 R r = \sqrt{(4 R^{2} - a^{2}) (4 r^{2} - a^{2})}

(R^{2} + r^{2} - R r) a^{2} = 3 R^{2} r^{2}

\Rightarrow a = R r \sqrt{\frac{3}{R^{2} + r^{2} - R r}}

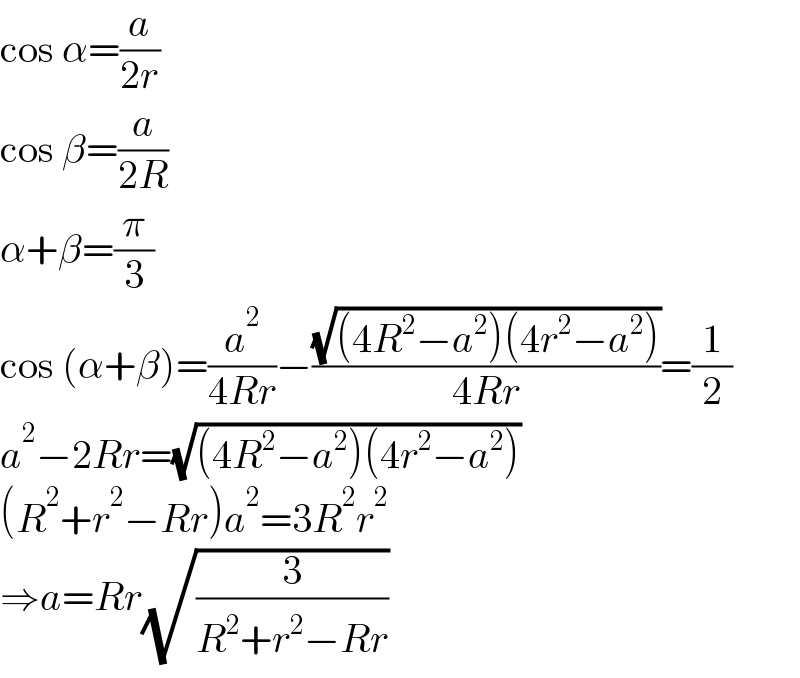
Commented by mr W last updated on 07/Jan/23

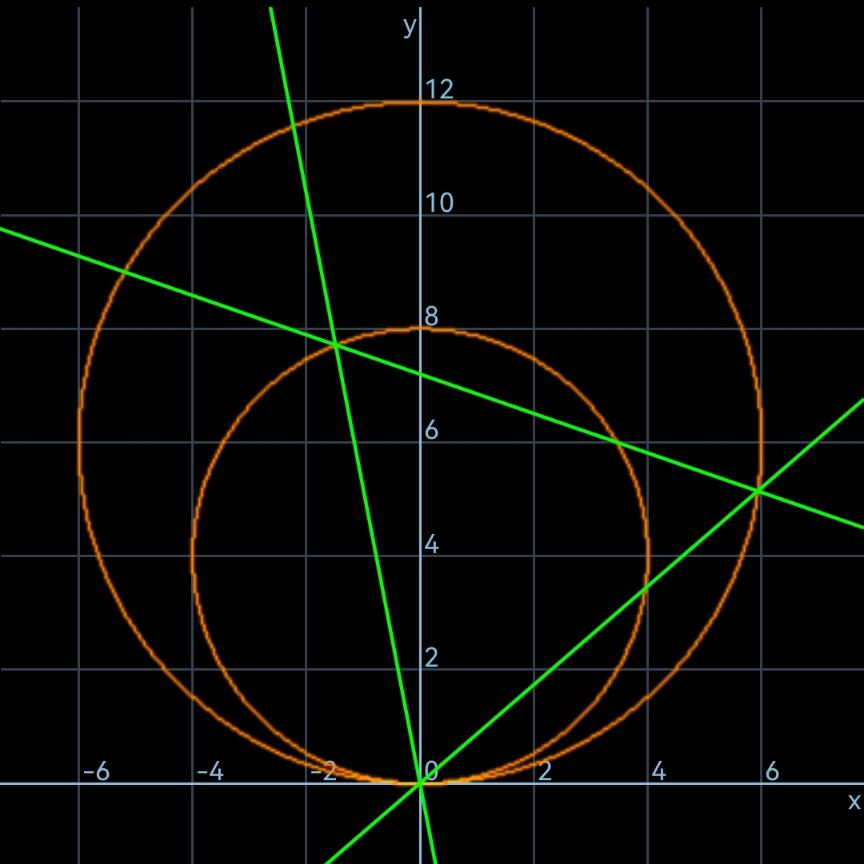
Commented by mr W last updated on 07/Jan/23

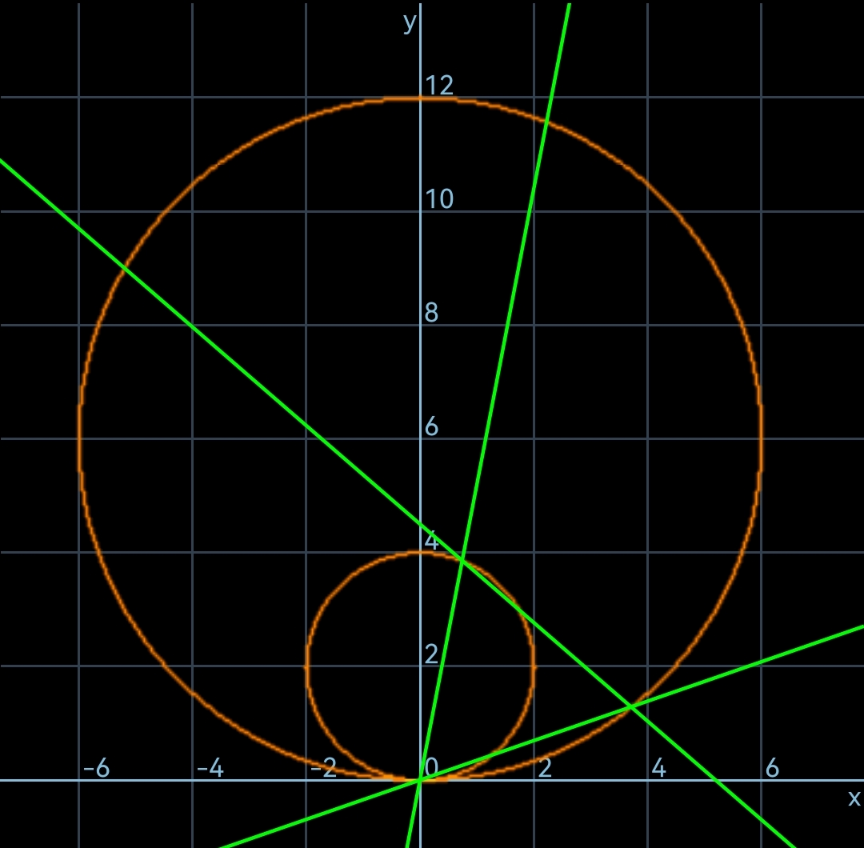
Commented by ajfour last updated on 08/Jan/23

T h a n k s s i r, {w e}^{'} v e g o t s a m e w a y s h e r e!

Facebook Tweet Pin