Question Number 185128 by Shrinava last updated on 17/Jan/23
Answered by Frix last updated on 17/Jan/23

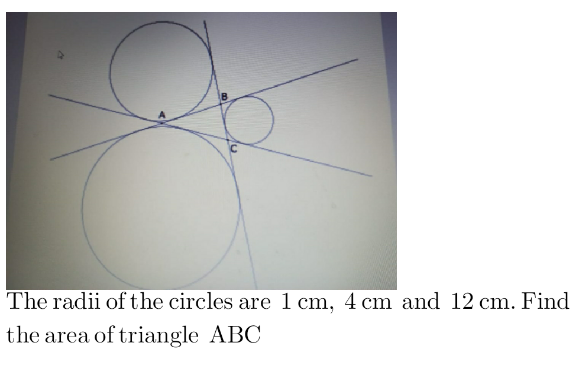
$$\mathrm{Formulas}\:\mathrm{for}\:\mathrm{incircle}\:\mathrm{and}\:\mathrm{3}\:\mathrm{excircles}: \\ $$$${r}=\frac{\mathrm{2}{A}}{{a}+{b}+{c}} \\ $$$${r}_{{a}} =\frac{\mathrm{2}{A}}{{b}+{c}−{a}} \\ $$$${r}_{{b}} =\frac{\mathrm{2}{A}}{{a}+{c}−{b}} \\ $$$${r}_{{c}} =\frac{\mathrm{2}{A}}{{a}+{b}−{c}} \\ $$$${A}^{\mathrm{2}} ={rr}_{{a}} {r}_{{b}} {r}_{{c}} \\ $$$$\Rightarrow \\ $$$$−{a}+{b}+{c}=\frac{\mathrm{2}{A}}{\mathrm{1}}=\mathrm{2}{A} \\ $$$${a}−{b}+{c}=\frac{\mathrm{2}{A}}{\mathrm{4}}=\frac{{A}}{\mathrm{2}} \\ $$$${a}+{b}−{c}=\frac{\mathrm{2}{A}}{\mathrm{12}}=\frac{{A}}{\mathrm{6}} \\ $$$$\Rightarrow \\ $$$${a}=\frac{{A}}{\mathrm{3}}\wedge{b}=\frac{\mathrm{13}{A}}{\mathrm{12}}\wedge{c}=\frac{\mathrm{5}{A}}{\mathrm{4}} \\ $$$$\Rightarrow\:{r}=\frac{\mathrm{3}}{\mathrm{4}} \\ $$$$\Rightarrow\:{A}=\mathrm{6} \\ $$
Commented by Shrinava last updated on 17/Jan/23

$$\mathrm{dear}\:\mathrm{ser}\:\mathrm{answer}:\:\mathrm{6}\:\:\mathrm{or}\:\:\mathrm{36}? \\ $$
Commented by Shrinava last updated on 17/Jan/23

$$\mathrm{but}\:\mathrm{answer}:\:\mathrm{6} \\ $$
Commented by Frix last updated on 17/Jan/23

$$\mathrm{I}\:\mathrm{corrected}\:\mathrm{it} \\ $$
