Question Number 185229 by Mingma last updated on 18/Jan/23
Answered by Rasheed.Sindhi last updated on 21/Jan/23
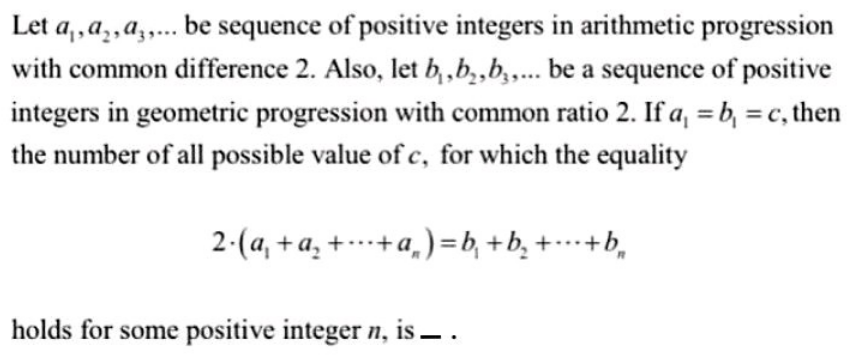
![A_S =(n/2)[2c+2(n−1)] G_S =((c(1−2^n ))/(1−2)) 2∙A_S =G_S 2((n/2)[2c+2(n−1)])=((c(1−2^n ))/(1−2)) 2cn+2n(n−1)=c(2^n −1) c(2^n −1)−2cn=2n(n−1) c(2^n −2n−1)=2n(n−1) c=((2n(n−1))/(2^n −2n−1)) For c to be +ve integer 2^n −2n−1 ∣ 2n(n−1) n=1:c=((2(1)(1−1))/(2^1 −2(1)−1))=0∉Z^+ n=2: c=((2(2)(2−1))/(2^2 −2(2)−1))=(4/(−1))=−4∉Z^+ n=3:c=((2(3)(3−1))/(2^3 −2(3)−1))=((12)/(8−6−1))=12✓ n=4:c=((2(4)(4−1))/(2^4 −2(4)−1))=((24)/7)∉Z^+ n=5:c=((2(5)(5−1))/(2^5 −2(5)−1))=((40)/(21))∉Z^+ n=6:c=((2(6)(6−1))/(2^6 −2(6)−1))=((60)/(51))∉Z^+ n>6: 2^n −2n−1>2n(n−1) and hence 2^n −2n−1 ∤ 2n(n−1) c=12 when n=3 Verification: 2(12+14+16)=^(?) 12+24+48 84=84](https://www.tinkutara.com/question/Q185255.png)
$${A}_{{S}} =\frac{{n}}{\mathrm{2}}\left[\mathrm{2}{c}+\mathrm{2}\left({n}−\mathrm{1}\right)\right] \\ $$$${G}_{{S}} =\frac{{c}\left(\mathrm{1}−\mathrm{2}^{{n}} \right)}{\mathrm{1}−\mathrm{2}} \\ $$$$\mathrm{2}\centerdot{A}_{{S}} ={G}_{{S}} \\ $$$$\mathrm{2}\left(\frac{{n}}{\mathrm{2}}\left[\mathrm{2}{c}+\mathrm{2}\left({n}−\mathrm{1}\right)\right]\right)=\frac{{c}\left(\mathrm{1}−\mathrm{2}^{{n}} \right)}{\mathrm{1}−\mathrm{2}} \\ $$$$\mathrm{2}{cn}+\mathrm{2}{n}\left({n}−\mathrm{1}\right)={c}\left(\mathrm{2}^{{n}} −\mathrm{1}\right) \\ $$$${c}\left(\mathrm{2}^{{n}} −\mathrm{1}\right)−\mathrm{2}{cn}=\mathrm{2}{n}\left({n}−\mathrm{1}\right) \\ $$$${c}\left(\mathrm{2}^{{n}} −\mathrm{2}{n}−\mathrm{1}\right)=\mathrm{2}{n}\left({n}−\mathrm{1}\right) \\ $$$${c}=\frac{\mathrm{2}{n}\left({n}−\mathrm{1}\right)}{\mathrm{2}^{{n}} −\mathrm{2}{n}−\mathrm{1}} \\ $$$${For}\:{c}\:{to}\:{be}\:+{ve}\:{integer} \\ $$$$\:\mathrm{2}^{{n}} −\mathrm{2}{n}−\mathrm{1}\:\mid\:\mathrm{2}{n}\left({n}−\mathrm{1}\right) \\ $$$${n}=\mathrm{1}:{c}=\frac{\mathrm{2}\left(\mathrm{1}\right)\left(\mathrm{1}−\mathrm{1}\right)}{\mathrm{2}^{\mathrm{1}} −\mathrm{2}\left(\mathrm{1}\right)−\mathrm{1}}=\mathrm{0}\notin\mathbb{Z}^{+} \\ $$$${n}=\mathrm{2}:\:{c}=\frac{\mathrm{2}\left(\mathrm{2}\right)\left(\mathrm{2}−\mathrm{1}\right)}{\mathrm{2}^{\mathrm{2}} −\mathrm{2}\left(\mathrm{2}\right)−\mathrm{1}}=\frac{\mathrm{4}}{−\mathrm{1}}=−\mathrm{4}\notin\mathbb{Z}^{+} \\ $$$${n}=\mathrm{3}:{c}=\frac{\mathrm{2}\left(\mathrm{3}\right)\left(\mathrm{3}−\mathrm{1}\right)}{\mathrm{2}^{\mathrm{3}} −\mathrm{2}\left(\mathrm{3}\right)−\mathrm{1}}=\frac{\mathrm{12}}{\mathrm{8}−\mathrm{6}−\mathrm{1}}=\mathrm{12}\checkmark \\ $$$${n}=\mathrm{4}:{c}=\frac{\mathrm{2}\left(\mathrm{4}\right)\left(\mathrm{4}−\mathrm{1}\right)}{\mathrm{2}^{\mathrm{4}} −\mathrm{2}\left(\mathrm{4}\right)−\mathrm{1}}=\frac{\mathrm{24}}{\mathrm{7}}\notin\mathbb{Z}^{+} \\ $$$${n}=\mathrm{5}:{c}=\frac{\mathrm{2}\left(\mathrm{5}\right)\left(\mathrm{5}−\mathrm{1}\right)}{\mathrm{2}^{\mathrm{5}} −\mathrm{2}\left(\mathrm{5}\right)−\mathrm{1}}=\frac{\mathrm{40}}{\mathrm{21}}\notin\mathbb{Z}^{+} \\ $$$${n}=\mathrm{6}:{c}=\frac{\mathrm{2}\left(\mathrm{6}\right)\left(\mathrm{6}−\mathrm{1}\right)}{\mathrm{2}^{\mathrm{6}} −\mathrm{2}\left(\mathrm{6}\right)−\mathrm{1}}=\frac{\mathrm{60}}{\mathrm{51}}\notin\mathbb{Z}^{+} \\ $$$${n}>\mathrm{6}:\:\mathrm{2}^{{n}} −\mathrm{2}{n}−\mathrm{1}>\mathrm{2}{n}\left({n}−\mathrm{1}\right) \\ $$$$\:{and}\:{hence}\:\mathrm{2}^{{n}} −\mathrm{2}{n}−\mathrm{1}\:\nmid\:\mathrm{2}{n}\left({n}−\mathrm{1}\right) \\ $$$${c}=\mathrm{12}\:{when}\:{n}=\mathrm{3} \\ $$$$\mathcal{V}{erification}: \\ $$$$\mathrm{2}\left(\mathrm{12}+\mathrm{14}+\mathrm{16}\right)\overset{?} {=}\mathrm{12}+\mathrm{24}+\mathrm{48} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{84}=\mathrm{84} \\ $$
