Question Number 185729 by Mingma last updated on 26/Jan/23

Commented by HeferH last updated on 26/Jan/23

$${not}\:{clear}\:{what}\:{segments}\:{are}\:{a},{b},{c} \\ $$
Commented by Mingma last updated on 26/Jan/23
The median BE of triangle ABC is trisected by its incircle. Calculate a:b:c
Commented by JDamian last updated on 26/Jan/23
I always was told that a triangle side is named using a lowercase letter after the uppercase vertex name located in front of that side -- the vertex having no contact with that side.
Commented by HeferH last updated on 26/Jan/23
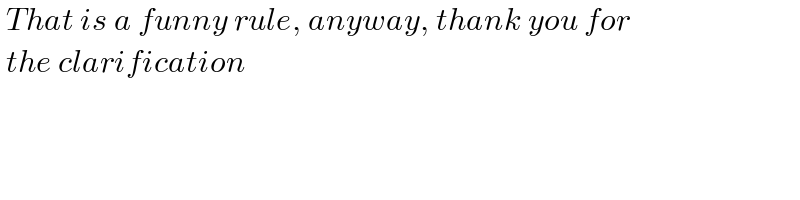
$$\:{That}\:{is}\:{a}\:{funny}\:{rule},\:{anyway},\:{thank}\:{you}\:{for} \\ $$$$\:{the}\:{clarification}\: \\ $$
Commented by Frix last updated on 26/Jan/23

$$\mathrm{Not}\:\mathrm{so}\:\mathrm{funny}. \\ $$$$\alpha=\measuredangle{BAC} \\ $$$$\mathrm{If}\:\mathrm{we}\:\mathrm{call}\:\mathrm{the}\:\mathrm{opposite}\:\mathrm{side}\:{a}\:\mathrm{etc}.\:\mathrm{we}\:\mathrm{get} \\ $$$$\frac{\mathrm{sin}\:\alpha}{{a}}=\frac{\mathrm{sin}\:\beta}{{b}}=\frac{\mathrm{sin}\:\gamma}{{c}} \\ $$$$\mathrm{and} \\ $$$${a}^{\mathrm{2}} +{b}^{\mathrm{2}} −\mathrm{2}{ab}\mathrm{cos}\gamma\:={c}^{\mathrm{2}} \\ $$$$\mathrm{where}\:\gamma\:\mathrm{is}\:\mathrm{the}\:\mathrm{angle}\:\mathrm{of}\:{a}\:\mathrm{and}\:{b} \\ $$
Commented by HeferH last updated on 27/Jan/23

$$\:{I}\:{don}'{t}\:{know}\:{if}\:{someone}\:{don}'{t}\:{use}\:{a},{b}\:{and}\:{c}\:{for}\: \\ $$$$\:{vertices}\:{A},{B}\:{and}\:{C}\:{gets}\:−\mathrm{1}\:{point}\:{or}\:{something}\: \\ $$
Commented by Frix last updated on 27/Jan/23

$$\mathrm{I}'\mathrm{ve}\:\mathrm{been}\:\mathrm{out}\:\mathrm{of}\:\mathrm{all}\:\mathrm{institutions}\:\mathrm{where}\:\mathrm{they} \\ $$$$\mathrm{can}\:\mathrm{take}\:\mathrm{points}\:\mathrm{from}\:\mathrm{you}\:\mathrm{for}\:\mathrm{more}\:\mathrm{than}\:\mathrm{15} \\ $$$$\mathrm{years}… \\ $$$$\mathrm{You}\:\mathrm{can}\:\mathrm{use}\:\mathrm{whatever}\:\mathrm{you}\:\mathrm{want}\:\mathrm{but}\:\mathrm{you} \\ $$$$\mathrm{have}\:\mathrm{to}\:\mathrm{explain}… \\ $$$$“\mathrm{Show}\:\mathrm{that}\:\mathfrak{F}^{\mathrm{2}} +\vartheta^{\mathrm{2}} −\mathrm{2}\mathfrak{F}\vartheta\mathrm{cos}\:\mathbb{V}\:={K}^{\mathrm{2}} ''\:\mathrm{might} \\ $$$$\mathrm{not}\:\mathrm{be}\:\mathrm{clear}\:\mathrm{enough} \\ $$
Commented by HeferH last updated on 27/Jan/23
��
Answered by mr W last updated on 27/Jan/23

Commented by mr W last updated on 27/Jan/23

$${say}\:{AK}={AH}={x} \\ $$$${BF}={FG}={GE}={m} \\ $$$${BD}^{\mathrm{2}} =\mathrm{2}{m}^{\mathrm{2}} \\ $$$${BK}^{\mathrm{2}} =\mathrm{2}{m}^{\mathrm{2}} \\ $$$${EH}^{\mathrm{2}} =\mathrm{2}{m}^{\mathrm{2}} \\ $$$$\Rightarrow{BD}={BK}={EH}=\frac{{b}}{\mathrm{2}}−{x} \\ $$$${AB}={c}={AE}=\frac{{b}}{\mathrm{2}}\:\Rightarrow\frac{{b}}{{c}}=\mathrm{2} \\ $$$${BC}={a}=\frac{{b}}{\mathrm{2}}−{x}+{b}−{x}=\frac{\mathrm{3}{b}}{\mathrm{2}}−\mathrm{2}{x} \\ $$$${BE}=\mathrm{3}{m}=\frac{\mathrm{3}×{BD}}{\:\sqrt{\mathrm{2}}}=\frac{\mathrm{3}}{\:\sqrt{\mathrm{2}}}\left(\frac{{b}}{\mathrm{2}}−{x}\right) \\ $$$$\mathrm{4}×{BE}^{\mathrm{2}} =\mathrm{2}{a}^{\mathrm{2}} +\mathrm{2}{c}^{\mathrm{2}} −{b}^{\mathrm{2}} \\ $$$$\mathrm{18}\left(\frac{{b}}{\mathrm{2}}−{x}\right)^{\mathrm{2}} =\mathrm{2}\left(\frac{\mathrm{3}{b}}{\mathrm{2}}−\mathrm{2}{x}\right)^{\mathrm{2}} +\mathrm{2}×\frac{{b}^{\mathrm{2}} }{\mathrm{4}}−{b}^{\mathrm{2}} \\ $$$$\mathrm{20}{x}^{\mathrm{2}} −\mathrm{12}{bx}+{b}^{\mathrm{2}} =\mathrm{0} \\ $$$$\Rightarrow{x}=\frac{{b}}{\mathrm{10}} \\ $$$$\Rightarrow{a}=\frac{\mathrm{3}{b}}{\mathrm{2}}−\mathrm{2}×\frac{{b}}{\mathrm{10}}=\frac{\mathrm{13}{b}}{\mathrm{10}}\:\Rightarrow\frac{{a}}{{b}}=\frac{\mathrm{13}}{\mathrm{10}} \\ $$$$\Rightarrow{a}:{b}:{c}=\mathrm{13}:\mathrm{10}:\mathrm{5}\:\checkmark \\ $$
