Question Number 187587 by Mingma last updated on 19/Feb/23
Commented by Mingma last updated on 19/Feb/23
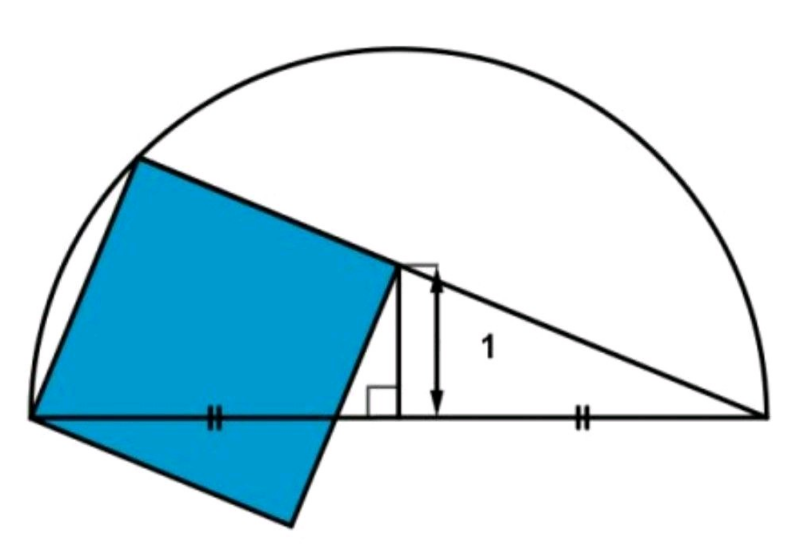
Blue area=?
Answered by mr W last updated on 19/Feb/23

Commented by mr W last updated on 19/Feb/23

$$\frac{{s}}{{s}+\sqrt{\mathrm{2}}{s}}=\frac{\mathrm{1}}{\:\sqrt{\left(\sqrt{\mathrm{2}}{s}\right)^{\mathrm{2}} −\mathrm{1}^{\mathrm{2}} }} \\ $$$$\Rightarrow{s}^{\mathrm{2}} =\mathrm{2}+\sqrt{\mathrm{2}}={blue}\:{area} \\ $$
Commented by Rupesh123 last updated on 22/Feb/23
Great work!
Answered by HeferH last updated on 19/Feb/23

Commented by HeferH last updated on 19/Feb/23
![d = (√(a^2 [(1+(√2))^2 +1])) = a(√(4+2(√2))) a(√2) = (√(4+2(√2))) a^2 = 2+(√2)](https://www.tinkutara.com/question/Q187657.png)
$${d}\:=\:\sqrt{{a}^{\mathrm{2}} \left[\left(\mathrm{1}+\sqrt{\mathrm{2}}\right)^{\mathrm{2}} +\mathrm{1}\right]}\:=\:{a}\sqrt{\mathrm{4}+\mathrm{2}\sqrt{\mathrm{2}}} \\ $$$${a}\sqrt{\mathrm{2}}\:=\:\sqrt{\mathrm{4}+\mathrm{2}\sqrt{\mathrm{2}}} \\ $$$${a}^{\mathrm{2}} \:=\:\mathrm{2}+\sqrt{\mathrm{2}} \\ $$
Commented by Rupesh123 last updated on 22/Feb/23
Great work!
