Question Number 187932 by Rupesh123 last updated on 23/Feb/23
Answered by a.lgnaoui last updated on 24/Feb/23
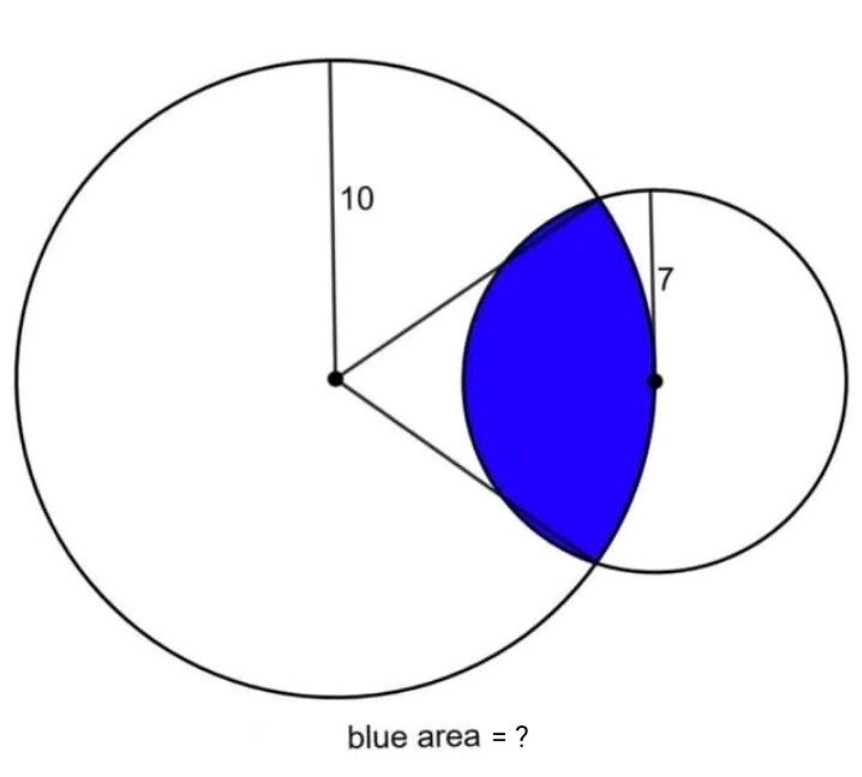
![O_1 :centre du cercle(C_1 )= origine Ref Cercles( C_1 )et(C_2 )d equations { ((x^2 +y_1 ^2 =100 (1))),(((x−10)^2 +y_2 ^2 =49 (2))) :} Ponts communs(M; N )definies par y_1 =y_2 ⇒ x=((151)/(20)) O_1 H=7,55 y=(√(100−((151^2 )/(20^2 )))) =±(√(43)) MH=O_1 Q=6,55724 Aire(Bleu) limite par C_1 erC_2 A=A_1 +A_2 tan α=((OQ)/(OH))= ((20(√(43)))/(151))⇒ [α=41°] Aire[A_1 ]=[αR_1 ^2 −O_1 H ×MH] α=((41π)/(180)) A_1 =((5×41π)/9)−49,5) Aire A_1 =22 Aire A_2 =ϕR_2 ^2 −O_2 H×MH O_2 H=OO−O_1 H=2,45⇒ tan ϕ=((MH)/(O_2 H)) ⇒ϕ≅ ((23π)/(60)) ⇒ AireA_2 =((49×23π)/(60)) −16=43 A=A_1 +A_2 Aire Totale=65 Totale generale Aire= 65+(αR_1 ^2 +ϕR_2 ^2 ) =65+131=196](https://www.tinkutara.com/question/Q188004.png)
$${O}_{\mathrm{1}} :{centre}\:{du}\:{cercle}\left({C}_{\mathrm{1}} \right)=\:\:\:{origine}\:{Ref} \\ $$$${Cercles}\left(\:{C}_{\mathrm{1}} \:\right){et}\left({C}_{\mathrm{2}} \right){d}\:{equations}\: \\ $$$$\begin{cases}{{x}^{\mathrm{2}} +{y}_{\mathrm{1}} ^{\mathrm{2}} \:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\mathrm{100}\:\:\left(\mathrm{1}\right)}\\{\left({x}−\mathrm{10}\right)^{\mathrm{2}} +{y}_{\mathrm{2}} ^{\mathrm{2}} =\mathrm{49}\:\:\:\:\:\left(\mathrm{2}\right)}\end{cases} \\ $$$${Ponts}\:{communs}\left({M};\:{N}\:\right){definies}\:{par} \\ $$$${y}_{\mathrm{1}} ={y}_{\mathrm{2}} \Rightarrow\:\:\:\:{x}=\frac{\mathrm{151}}{\mathrm{20}}\:\:\:\:\:\:\:{O}_{\mathrm{1}} {H}=\mathrm{7},\mathrm{55}\:\: \\ $$$${y}=\sqrt{\mathrm{100}−\frac{\mathrm{151}^{\mathrm{2}} }{\mathrm{20}^{\mathrm{2}} }}\:=\pm\sqrt{\mathrm{43}}\:\:\:\:{MH}={O}_{\mathrm{1}} {Q}=\mathrm{6},\mathrm{55724} \\ $$$${Aire}\left({Bleu}\right)\:{limite}\:{par}\:{C}_{\mathrm{1}} \:{erC}_{\mathrm{2}} \\ $$$${A}={A}_{\mathrm{1}} +{A}_{\mathrm{2}} \\ $$$$\mathrm{tan}\:\alpha=\frac{{OQ}}{{OH}}=\:\frac{\mathrm{20}\sqrt{\mathrm{43}}}{\mathrm{151}}\Rightarrow\:\:\:\left[\alpha=\mathrm{41}°\right] \\ $$$${Aire}\left[{A}_{\mathrm{1}} \right]=\left[\alpha{R}_{\mathrm{1}} ^{\mathrm{2}} −{O}_{\mathrm{1}} {H}\:×{MH}\right]\:\:\:\alpha=\frac{\mathrm{41}\pi}{\mathrm{180}} \\ $$$$\left.{A}_{\mathrm{1}} =\frac{\mathrm{5}×\mathrm{41}\pi}{\mathrm{9}}−\mathrm{49},\mathrm{5}\right)\:\:\:\:\boldsymbol{{Aire}}\:\boldsymbol{{A}}_{\mathrm{1}} =\mathrm{22} \\ $$$$ \\ $$$${Aire}\:{A}_{\mathrm{2}} =\varphi{R}_{\mathrm{2}} ^{\mathrm{2}} −{O}_{\mathrm{2}} {H}×{MH}\:\:\: \\ $$$$\:{O}_{\mathrm{2}} {H}={OO}−{O}_{\mathrm{1}} {H}=\mathrm{2},\mathrm{45}\Rightarrow\:\:\mathrm{tan}\:\varphi=\frac{{MH}}{{O}_{\mathrm{2}} {H}}\: \\ $$$$\Rightarrow\varphi\cong\:\:\frac{\mathrm{23}\pi}{\mathrm{60}}\:\Rightarrow\:\:\boldsymbol{{A}}{ireA}_{\mathrm{2}} =\frac{\mathrm{49}×\mathrm{23}\pi}{\mathrm{60}}\:−\mathrm{16}=\mathrm{43} \\ $$$$\:\:\:\boldsymbol{{A}}=\boldsymbol{{A}}_{\mathrm{1}} +\boldsymbol{{A}}_{\mathrm{2}} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\boldsymbol{{Aire}}\:\boldsymbol{{Totale}}=\mathrm{65} \\ $$$$ \\ $$$$\:\:\:\:{Totale}\:{generale} \\ $$$${A}\boldsymbol{{ire}}=\:\:\mathrm{65}+\left(\alpha{R}_{\mathrm{1}} ^{\mathrm{2}} +\varphi{R}_{\mathrm{2}} ^{\mathrm{2}} \right) \\ $$$$\:\:\:\:=\mathrm{65}+\mathrm{131}=\mathrm{196} \\ $$$$ \\ $$$$\:\: \\ $$
Commented by a.lgnaoui last updated on 25/Feb/23

Answered by mr W last updated on 24/Feb/23

Commented by mr W last updated on 24/Feb/23

$$\mathrm{sin}\:\gamma=\mathrm{cos}\:\beta=\frac{\mathrm{7}}{\mathrm{20}} \\ $$$$\mathrm{sin}\:\mathrm{2}\beta=\mathrm{2}×\frac{\mathrm{7}}{\mathrm{20}}×\frac{\sqrt{\mathrm{20}^{\mathrm{2}} −\mathrm{7}^{\mathrm{2}} }}{\mathrm{20}}=\frac{\mathrm{21}\sqrt{\mathrm{39}}}{\mathrm{200}} \\ $$$$\alpha=\mathrm{2}\gamma \\ $$$$\mathrm{cos}\:\alpha=\mathrm{1}−\mathrm{2}×\left(\frac{\mathrm{7}}{\mathrm{20}}\right)^{\mathrm{2}} =\frac{\mathrm{151}}{\mathrm{200}} \\ $$$$\mathrm{sin}\:\mathrm{2}\alpha=\mathrm{2}×\frac{\mathrm{151}}{\mathrm{200}}×\frac{\sqrt{\mathrm{200}^{\mathrm{2}} −\mathrm{151}^{\mathrm{2}} }}{\mathrm{200}}=\frac{\mathrm{3171}\sqrt{\mathrm{39}}}{\mathrm{20000}} \\ $$$${A}_{{blue}} =\frac{\mathrm{10}^{\mathrm{2}} }{\mathrm{2}}\left(\mathrm{2}\alpha−\mathrm{sin}\:\mathrm{2}\alpha\right)+\frac{\mathrm{7}^{\mathrm{2}} }{\mathrm{2}}\left(\mathrm{2}\beta−\mathrm{sin}\:\mathrm{2}\beta\right) \\ $$$$\:\:=\frac{\mathrm{10}^{\mathrm{2}} }{\mathrm{2}}\left(\mathrm{4}\:\mathrm{sin}^{−\mathrm{1}} \frac{\mathrm{7}}{\mathrm{20}}−\frac{\mathrm{3171}\sqrt{\mathrm{39}}}{\mathrm{20000}}\right)+\frac{\mathrm{7}^{\mathrm{2}} }{\mathrm{2}}\left(\mathrm{2}\:\mathrm{cos}^{−\mathrm{1}} \frac{\mathrm{7}}{\mathrm{20}}−\frac{\mathrm{21}\sqrt{\mathrm{39}}}{\mathrm{200}}\right) \\ $$$$\:\:\approx\mathrm{65}.\mathrm{389}\:\mathrm{778} \\ $$
Commented by Rupesh123 last updated on 01/Mar/23
Excellent!
