Question-189123

Question Number 189123 by Rupesh123 last updated on 12/Mar/23

Answered by mr W last updated on 13/Mar/23

Commented by mr W last updated on 13/Mar/23

let AC=p, AD=q ((AE)/(BF))=((AC)/(BC))=((CE)/(CF)) ((AE)/1)=(p/1)=(5/(CF)) ⇒AE=p, CF=(5/p) similarly ⇒AB=((3q)/2), DG=(8/q) cos α=((2^2 +p^2 −q^2 )/(2×2×p))=((6^2 +p^2 −(((3q)/2))^2 )/(2×6×p)) ⇒8p^2 −3q^2 =96 ...(i) cos β=((1^2 +(((3q)/2))^2 −p^2 )/(2×1×((3q)/2)))=((6^2 +(((3q)/2))^2 −p^2 )/(2×6×((3q)/2))) ⇒9q^2 −4p^2 =24 ...(ii) from (i) and (ii): ⇒p^2 =((78)/5), q^2 =((48)/5) ((x^2 −(p+(5/p))^2 −(q+(8/q))^2 )/(2×(p+(5/p))(q+(8/q))))=((3^2 −p^2 −q^2 )/(2×p×q)) x^2 =26+p^2 +((25)/p^2 )+q^2 +((64)/q^2 )+(9−p^2 −q^2 )(1+(5/p^2 ))(1+(8/q^2 )) x^2 =26+((78)/5)+((25×5)/(78))+((48)/5)+((64×5)/(48))+(9−((78)/5)−((48)/5))(1+((5×5)/(78)))(1+((8×5)/(48))) =((81)/4) ⇒x=(9/2)=4.5 ✓

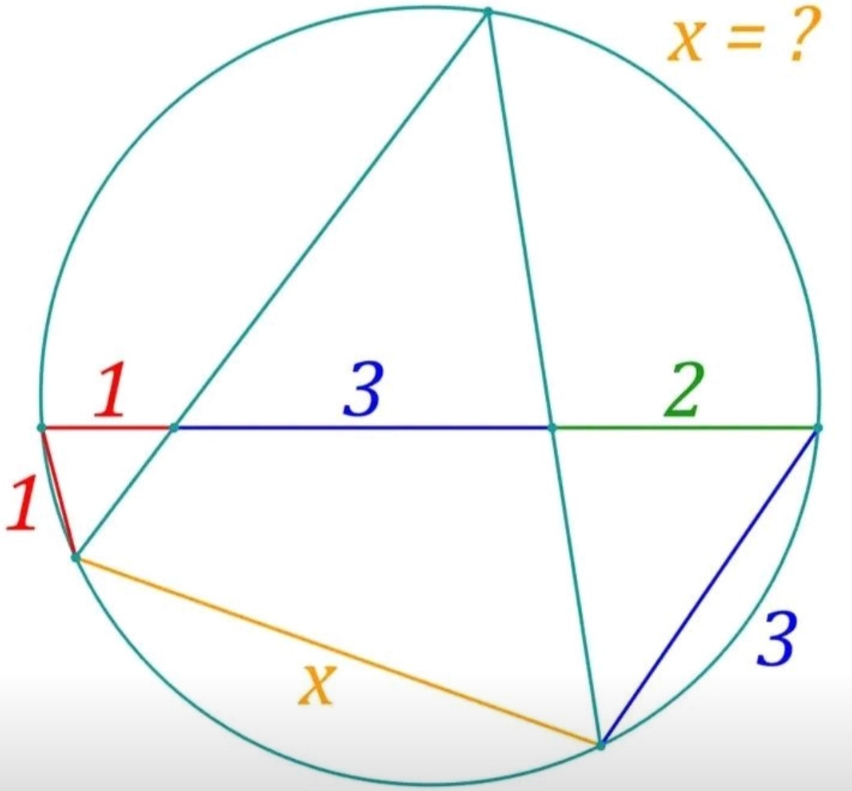
$${let}\:{AC}={p},\:{AD}={q} \\ $$$$\frac{{AE}}{{BF}}=\frac{{AC}}{{BC}}=\frac{{CE}}{{CF}} \\ $$$$\frac{{AE}}{\mathrm{1}}=\frac{{p}}{\mathrm{1}}=\frac{\mathrm{5}}{{CF}} \\ $$$$\:\Rightarrow{AE}={p},\:{CF}=\frac{\mathrm{5}}{{p}} \\ $$$${similarly} \\ $$$$\Rightarrow{AB}=\frac{\mathrm{3}{q}}{\mathrm{2}},\:{DG}=\frac{\mathrm{8}}{{q}} \\ $$$$\mathrm{cos}\:\alpha=\frac{\mathrm{2}^{\mathrm{2}} +{p}^{\mathrm{2}} −{q}^{\mathrm{2}} }{\mathrm{2}×\mathrm{2}×{p}}=\frac{\mathrm{6}^{\mathrm{2}} +{p}^{\mathrm{2}} −\left(\frac{\mathrm{3}{q}}{\mathrm{2}}\right)^{\mathrm{2}} }{\mathrm{2}×\mathrm{6}×{p}} \\ $$$$\Rightarrow\mathrm{8}{p}^{\mathrm{2}} −\mathrm{3}{q}^{\mathrm{2}} =\mathrm{96}\:\:\:…\left({i}\right) \\ $$$$\mathrm{cos}\:\beta=\frac{\mathrm{1}^{\mathrm{2}} +\left(\frac{\mathrm{3}{q}}{\mathrm{2}}\right)^{\mathrm{2}} −{p}^{\mathrm{2}} }{\mathrm{2}×\mathrm{1}×\frac{\mathrm{3}{q}}{\mathrm{2}}}=\frac{\mathrm{6}^{\mathrm{2}} +\left(\frac{\mathrm{3}{q}}{\mathrm{2}}\right)^{\mathrm{2}} −{p}^{\mathrm{2}} }{\mathrm{2}×\mathrm{6}×\frac{\mathrm{3}{q}}{\mathrm{2}}} \\ $$$$\Rightarrow\mathrm{9}{q}^{\mathrm{2}} −\mathrm{4}{p}^{\mathrm{2}} =\mathrm{24}\:\:\:…\left({ii}\right) \\ $$$${from}\:\left({i}\right)\:{and}\:\left({ii}\right): \\ $$$$\Rightarrow{p}^{\mathrm{2}} =\frac{\mathrm{78}}{\mathrm{5}},\:{q}^{\mathrm{2}} =\frac{\mathrm{48}}{\mathrm{5}} \\ $$$$\frac{{x}^{\mathrm{2}} −\left({p}+\frac{\mathrm{5}}{{p}}\right)^{\mathrm{2}} −\left({q}+\frac{\mathrm{8}}{{q}}\right)^{\mathrm{2}} }{\mathrm{2}×\left({p}+\frac{\mathrm{5}}{{p}}\right)\left({q}+\frac{\mathrm{8}}{{q}}\right)}=\frac{\mathrm{3}^{\mathrm{2}} −{p}^{\mathrm{2}} −{q}^{\mathrm{2}} }{\mathrm{2}×{p}×{q}} \\ $$$${x}^{\mathrm{2}} =\mathrm{26}+{p}^{\mathrm{2}} +\frac{\mathrm{25}}{{p}^{\mathrm{2}} }+{q}^{\mathrm{2}} +\frac{\mathrm{64}}{{q}^{\mathrm{2}} }+\left(\mathrm{9}−{p}^{\mathrm{2}} −{q}^{\mathrm{2}} \right)\left(\mathrm{1}+\frac{\mathrm{5}}{{p}^{\mathrm{2}} }\right)\left(\mathrm{1}+\frac{\mathrm{8}}{{q}^{\mathrm{2}} }\right) \\ $$$${x}^{\mathrm{2}} =\mathrm{26}+\frac{\mathrm{78}}{\mathrm{5}}+\frac{\mathrm{25}×\mathrm{5}}{\mathrm{78}}+\frac{\mathrm{48}}{\mathrm{5}}+\frac{\mathrm{64}×\mathrm{5}}{\mathrm{48}}+\left(\mathrm{9}−\frac{\mathrm{78}}{\mathrm{5}}−\frac{\mathrm{48}}{\mathrm{5}}\right)\left(\mathrm{1}+\frac{\mathrm{5}×\mathrm{5}}{\mathrm{78}}\right)\left(\mathrm{1}+\frac{\mathrm{8}×\mathrm{5}}{\mathrm{48}}\right) \\ $$$$\:\:\:\:\:=\frac{\mathrm{81}}{\mathrm{4}} \\ $$$$\Rightarrow{x}=\frac{\mathrm{9}}{\mathrm{2}}=\mathrm{4}.\mathrm{5}\:\checkmark \\ $$

Commented by Rupesh123 last updated on 14/Mar/23

Excellent!

Answered by HeferH last updated on 13/Mar/23