Question Number 189189 by MikeH last updated on 13/Mar/23

Answered by cortano12 last updated on 13/Mar/23

$$\mathrm{it}\:\mathrm{should}\:\mathrm{be}\:\underset{−\mathrm{1}/\mathrm{2}} {\overset{\mathrm{1}/\mathrm{2}} {\int}}\sqrt{\mathrm{x}^{\mathrm{2}} +\mathrm{1}+\sqrt{\mathrm{x}^{\mathrm{4}} +\mathrm{x}^{\mathrm{2}} +\mathrm{1}}}\:\mathrm{dx} \\ $$$$=\:\underset{−\mathrm{1}/\mathrm{2}} {\overset{\mathrm{1}/\mathrm{2}} {\int}}\sqrt{\frac{\mathrm{x}^{\mathrm{2}} +\mathrm{x}+\mathrm{1}}{\mathrm{2}}}\:+\sqrt{\frac{\mathrm{x}^{\mathrm{2}} −\mathrm{x}+\mathrm{1}}{\mathrm{2}}}\:\mathrm{dx} \\ $$$$=\:\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}\:\underset{−\mathrm{1}/\mathrm{2}} {\overset{\mathrm{1}/\mathrm{2}} {\int}}\sqrt{\left(\mathrm{x}+\frac{\mathrm{1}}{\mathrm{2}}\right)^{\mathrm{2}} +\frac{\mathrm{3}}{\mathrm{4}}}+\sqrt{\left(\mathrm{x}−\frac{\mathrm{1}}{\mathrm{2}}\right)^{\mathrm{2}} +\frac{\mathrm{3}}{\mathrm{4}}}\:\mathrm{dx} \\ $$$$=\:\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}\:\underset{\mathrm{0}} {\int}^{\mathrm{sinh}\:^{−\mathrm{1}} \left(\frac{\mathrm{2}}{\:\sqrt{\mathrm{3}}}\right)} \frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\mathrm{cosh}\:^{\mathrm{2}} \left(\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\theta\right)\mathrm{d}\theta \\ $$$$\:+\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}\:\underset{\mathrm{sinh}\:^{−\mathrm{1}} \left(\frac{\mathrm{2}}{\:\sqrt{\mathrm{3}}}\right)} {\overset{\mathrm{0}} {\int}}\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\mathrm{cosh}\:^{\mathrm{2}} \left(\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\theta\right)\mathrm{d}\theta \\ $$$$=\:\frac{\sqrt{\mathrm{7}}}{\mathrm{2}\sqrt{\mathrm{2}}}\:+\:\frac{\mathrm{3}}{\mathrm{4}\sqrt{\mathrm{2}}}\:\mathrm{ln}\:\left(\frac{\mathrm{2}+\sqrt{\mathrm{7}}}{\:\sqrt{\mathrm{3}}}\right) \\ $$
Commented by Frix last updated on 13/Mar/23
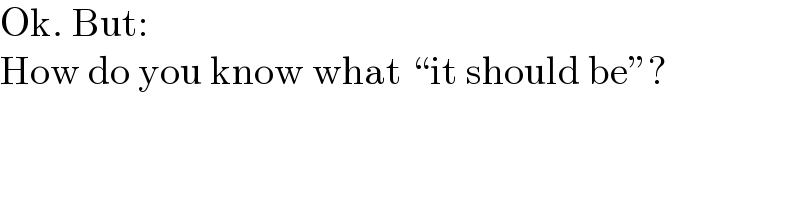
$$\mathrm{Ok}.\:\mathrm{But}: \\ $$$$\mathrm{How}\:\mathrm{do}\:\mathrm{you}\:\mathrm{know}\:\mathrm{what}\:“\mathrm{it}\:\mathrm{should}\:\mathrm{be}''? \\ $$
Commented by cortano12 last updated on 13/Mar/23

$$\mathrm{what}\:\mathrm{the}\:\mathrm{meaning} \\ $$$$\sqrt{\mathrm{x}^{\mathrm{2}} +\mathrm{1}\left(\sqrt{\left.\mathrm{x}^{\mathrm{4}} +\mathrm{x}^{\mathrm{2}} +\mathrm{1}\right)}\right.}? \\ $$
