Question Number 22742 by selestian last updated on 22/Oct/17

Answered by Sahib singh last updated on 22/Oct/17

$$\frac{\mathrm{3}}{\mathrm{4}}\:\:? \\ $$
Commented by math solver last updated on 22/Oct/17

$${yup}! \\ $$
Commented by Sahib singh last updated on 22/Oct/17

$$\mathrm{want}\:\mathrm{explanation}\:? \\ $$
Commented by math solver last updated on 22/Oct/17

$${i}\:{think}\:{selestian}\:{want}\:{explanation}. \\ $$
Commented by Sahib singh last updated on 22/Oct/17

$$\left.\mathrm{lol}\:;\right) \\ $$
Commented by Sahib singh last updated on 22/Oct/17

$$\mathrm{if}\:\mathrm{i}\:\mathrm{give}\:\mathrm{explanation}\:\mathrm{he}\: \\ $$$$\mathrm{might}\:\mathrm{feel}\:\mathrm{very}\:\mathrm{bad}.\mathrm{lol} \\ $$
Commented by Sahib singh last updated on 22/Oct/17
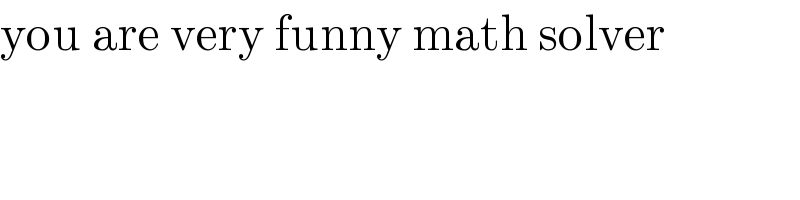
$$\mathrm{you}\:\mathrm{are}\:\mathrm{very}\:\mathrm{funny}\:\mathrm{math}\:\mathrm{solver} \\ $$
Commented by selestian last updated on 22/Oct/17
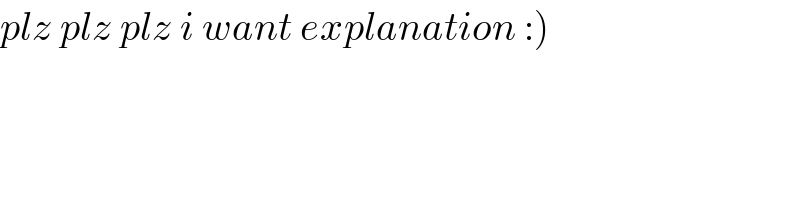
$$\left.{plz}\:{plz}\:{plz}\:{i}\:{want}\:{explanation}\::\right) \\ $$
Commented by selestian last updated on 22/Oct/17

$${hahha}\:{i}\:{got}\:{it}\:{woooo}\:{no}\:{need}\:{for}\: \\ $$$${solution}…{a}+{b}=\mathrm{60}\:{thats}\:{the}\:{trick}… \\ $$$${I}\:{was}\:{using}\:{different}\:{approach}\:{back} \\ $$$${there} \\ $$
Commented by NECx last updated on 22/Oct/17

$${please}\:{if}\:{you}\:{have}\:{an}\:{explanation} \\ $$$${give}\:{it}.\:{Other}\:{gurus}\:{like}\:{mrW}\mathrm{1}\:, \\ $$$${satyam}\:,\:{Ajfour}\:{n}\:{the}\:{others}\: \\ $$$${solve}\:{questions}\:{with}\:{necessary} \\ $$$${solutions}\:{irrespective}\:{of}\:{how}\: \\ $$$${simple}\:{the}\:{question}\:{may}\:{be}. \\ $$$$ \\ $$$${note}:\:{not}\:{everyone}\:{understands} \\ $$$${maths}\:{the}\:{way}\:{you}\:{do}. \\ $$$$ \\ $$$${Thanks}\:{for}\:{understanding}. \\ $$$$ \\ $$
Commented by Sahib singh last updated on 22/Oct/17

$$ \\ $$$$\mathrm{I}\:\mathrm{did}\:\mathrm{not}\:\mathrm{intend}\:\mathrm{to}\:\mathrm{show}\:\mathrm{my} \\ $$$$\mathrm{superiority}.\mathrm{I}\:\mathrm{just}\:\mathrm{wanted} \\ $$$$\mathrm{him}\:\mathrm{to}\:\mathrm{understand}\:\mathrm{that}\:\mathrm{simple} \\ $$$$\mathrm{trick}.\mathrm{I}\:\mathrm{wanted}\:\mathrm{him}\:\mathrm{to}\:\mathrm{give} \\ $$$$\mathrm{it}\:\mathrm{a}\:\mathrm{second}\:\mathrm{thought}. \\ $$$$\mathrm{Anyways},\:\mathrm{I}\:\mathrm{apologize}.\mathrm{I}\: \\ $$$$\mathrm{wont}\:\mathrm{do}\:\mathrm{that}\:\mathrm{again}.\mathrm{sorry}. \\ $$
Commented by Sahib singh last updated on 22/Oct/17

$$ \\ $$$$ \\ $$$$\mathrm{solution} \\ $$$$\mathrm{we}\:\mathrm{can}\:\mathrm{use}\:\mathrm{the}\:\mathrm{identity} \\ $$$$\mathrm{cos}^{\mathrm{2}} \mathrm{A}\:+\:\mathrm{cos}^{\mathrm{2}} \:\mathrm{B}\:+\:\mathrm{cos}^{\mathrm{2}} \:\mathrm{C} \\ $$$$=\mathrm{1}\:−\:\mathrm{2cos}\:\mathrm{A}\centerdot\mathrm{cos}\:\mathrm{B}\centerdot\mathrm{cos}\:\mathrm{C} \\ $$$$\mathrm{if}\:\mathrm{A}+\mathrm{B}+\mathrm{C}=\pi \\ $$$$ \\ $$$$\Rightarrow\mathrm{cos}^{\mathrm{2}} \mathrm{A}\:+\:\mathrm{cos}^{\mathrm{2}} \mathrm{B}\:+\:\mathrm{cos}^{\mathrm{2}} \left(\frac{\mathrm{2}\pi}{\mathrm{3}}\right) \\ $$$$=\mathrm{1}−\mathrm{2cosA}\centerdot\mathrm{cosB}\centerdot\mathrm{cos}\left(\frac{\mathrm{2}\pi}{\mathrm{3}}\right) \\ $$$$\Rightarrow\mathrm{cos}^{\mathrm{2}} \mathrm{A}+\mathrm{cos}^{\mathrm{2}} \mathrm{B}\:+\:\frac{\mathrm{1}}{\mathrm{4}}\:= \\ $$$$\:\mathrm{1}\:−\:\mathrm{2}\:\mathrm{cosA}\centerdot\mathrm{cosB}\centerdot\left(\frac{−\mathrm{1}}{\mathrm{2}}\right) \\ $$$$\Rightarrow\mathrm{cos}^{\mathrm{2}} \mathrm{A}+\mathrm{cos}^{\mathrm{2}} \mathrm{B}−\mathrm{cos}\centerdot\mathrm{AcosB} \\ $$$$=\:\frac{\mathrm{3}}{\mathrm{4}} \\ $$$$ \\ $$$$\mathrm{Trick}\:: \\ $$$$\mathrm{if}\:\mathrm{C}\:=\:\mathrm{120}° \\ $$$$\Rightarrow\:\mathrm{A}+\mathrm{B}\:=\:\mathrm{60}° \\ $$$$\mathrm{we}\:\mathrm{can}\:\mathrm{solve}\:\mathrm{the}\:\mathrm{question} \\ $$$$\mathrm{in}\:\:\mathrm{the}\:\mathrm{CASE}\:\mathrm{when}\:\mathrm{A}=\mathrm{B}=\mathrm{30}° \\ $$$$\mathrm{because}\:\mathrm{the}\:\mathrm{given}\:\mathrm{identity}\:\mathrm{is} \\ $$$$\mathrm{true}\:\mathrm{for}\:\mathrm{every}\:\mathrm{A}+\mathrm{B}=\mathrm{60}° \\ $$$$ \\ $$$$ \\ $$$$ \\ $$
Commented by NECx last updated on 22/Oct/17

$${thanks}\:{boss}…\:{I}\:{get}\:{you}\:{now}\:{but} \\ $$$${I}'{m}\:{more}\:{happy}\:{seeing}\:{the} \\ $$$${solution}.{I}\:{love}\:{your}\:{steps}. \\ $$
Commented by $@ty@m last updated on 22/Oct/17

$${We}\:{should}\:{not}\:{run}\:{behind}\:{STEPS} \\ $$$${all}\:{the}\:{time}. \\ $$$${I}\:{liked}\:{approach}\:{of}\:{Selestian}. \\ $$$${Such}\:{trics}\:{are}\:{very}\:{useful}\:{for} \\ $$$${MCQ}\:{where}\:{limited}\:{time}\:{is}\:{given}. \\ $$
Commented by Physics lover last updated on 22/Oct/17

$${that}'{s}\:{why}\:{i}\:{did}\:{not}\:{tell}\:{the}\:{solution} \\ $$$${at}\:{first}.{Wanted}\:{his}\:{mind}\:{to}\:{open}\:{up}. \\ $$$${i}\:{knew}\:{he}\:{can}\:{get}\:{it}\:{if}\:{he}\:{thinks}\:{a}\: \\ $$$${little}\:{more}. \\ $$
Commented by Physics lover last updated on 22/Oct/17

$${many}\:{times}\:{we}\:{keep}\:{on}\:{focusing} \\ $$$${on}\:{complicated}\:{things}\:{and}\:{pay} \\ $$$${little}\:{attention}\:{to}\:{very}\:{simple}\:{and} \\ $$$${easier}\:{things}. \\ $$
Answered by ajfour last updated on 22/Oct/17

$$\mathrm{cos}\:^{\mathrm{2}} {A}+\mathrm{cos}\:^{\mathrm{2}} {B}−\mathrm{cos}\:{A}\mathrm{cos}\:{B} \\ $$$$=\frac{\mathrm{2}+\mathrm{cos}\:\mathrm{2}{A}+\mathrm{cos}\:\mathrm{2}{B}−\mathrm{cos}\:\left({A}+{B}\right)−\mathrm{cos}\:\left({A}−{B}\right)}{\mathrm{2}} \\ $$$$=\mathrm{1}+\mathrm{cos}\:\left({A}+{B}\right)\mathrm{cos}\:\left({A}−{B}\right)−\frac{\mathrm{1}}{\mathrm{2}}\mathrm{cos}\:\left({A}+{B}\right)−\frac{\mathrm{1}}{\mathrm{2}}\mathrm{cos}\:\left({A}−{B}\right) \\ $$$$=\mathrm{1}+\frac{\mathrm{1}}{\mathrm{2}}\mathrm{cos}\:\left({A}−{B}\right)−\frac{\mathrm{1}}{\mathrm{4}}−\frac{\mathrm{1}}{\mathrm{2}}\mathrm{cos}\:\left({A}−{B}\right) \\ $$$$\:\:\:\:\:=\frac{\mathrm{3}}{\mathrm{4}}\:. \\ $$
