Question Number 23036 by ajfour last updated on 25/Oct/17
Commented by mrW1 last updated on 25/Oct/17
![(([PQRSTU])/([ABCDEF]))=(1/7)](https://www.tinkutara.com/question/Q23047.png)
$$\frac{\left[\boldsymbol{{PQRSTU}}\right]}{\left[\boldsymbol{{ABCDEF}}\right]}=\frac{\mathrm{1}}{\mathrm{7}} \\ $$
Commented by ajfour last updated on 25/Oct/17

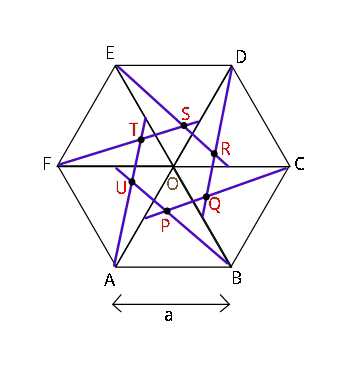
$${The}\:{blue}\:{line}\:{segment}\:{from}\:{A} \\ $$$${starts}\:{at}\:{A}\:{and}\:{ends}\:{in}\:{midpoint} \\ $$$${of}\:{OE},\:{blue}\:{line}\:{segment}\:{from}\:{B} \\ $$$${ends}\:{in}\:{midpoint}\:{of}\:{OF},..\:{and} \\ $$$${so}\:{on}.\:{Find}\:{ratio}\:\boldsymbol{{area}}\:\boldsymbol{{of}}\:{regular} \\ $$$${hexagon}\:\boldsymbol{{PQRSTU}}\:{so}\:{formed}, \\ $$$${to}\:{the}\:\boldsymbol{{area}}\:\boldsymbol{{of}}\:{given}\:{regular} \\ $$$${hexagon}\:\boldsymbol{{ABCDEF}}\:{of}\:{side}\:\boldsymbol{{a}}. \\ $$
Commented by ajfour last updated on 25/Oct/17

$${Pleaze}\:{explain}\:{Sir}. \\ $$$${I}\:{created}\:{this}\:{question}\:{myself}, \\ $$$${haven}'{t}\:{even}\:{attempted},\:{nice} \\ $$$${answer}\:{sir}.\:{It}\:{will}\:{take}\:{a}\:{long} \\ $$$${method}\:{if}\:{i}\:{solve}\:{with}\:{vectord} \\ $$$${or}\:{coordinate}\:{geometry}. \\ $$$${Kindly}\:{explain}\:{your}\:{way}\:{sir}. \\ $$
Commented by mrW1 last updated on 25/Oct/17
Commented by mrW1 last updated on 25/Oct/17

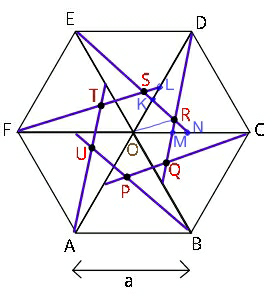
$$\mathrm{let}\:\mathrm{a}=\mathrm{1}\:\mathrm{to}\:\mathrm{make}\:\mathrm{the}\:\mathrm{writing}\:\mathrm{easier}. \\ $$$$ \\ $$$$\mathrm{OE}=\mathrm{OD}=…=\mathrm{a}=\mathrm{1} \\ $$$$\mathrm{OL}=\mathrm{ON}=…=\frac{\mathrm{a}}{\mathrm{2}}=\frac{\mathrm{1}}{\mathrm{2}} \\ $$$$\mathrm{EN}^{\mathrm{2}} =\mathrm{1}^{\mathrm{2}} +\left(\frac{\mathrm{1}}{\mathrm{2}}\right)^{\mathrm{2}} −\mathrm{2}×\mathrm{1}×\frac{\mathrm{1}}{\mathrm{2}}×\mathrm{cos}\:\mathrm{120}°=\mathrm{1}+\frac{\mathrm{1}}{\mathrm{4}}+\frac{\mathrm{1}}{\mathrm{2}}=\frac{\mathrm{7}}{\mathrm{4}} \\ $$$$\Rightarrow\mathrm{EN}=\frac{\sqrt{\mathrm{7}}}{\mathrm{2}} \\ $$$$ \\ $$$$\mathrm{OK}\:\mathrm{is}\:\mathrm{the}\:\mathrm{angle}\:\mathrm{bisector}\:\mathrm{of}\:\angle\mathrm{EON}, \\ $$$$\Rightarrow\frac{\mathrm{EK}}{\mathrm{KN}}=\frac{\mathrm{OE}}{\mathrm{ON}}=\mathrm{2} \\ $$$$\Rightarrow\mathrm{EK}=\frac{\mathrm{2}}{\mathrm{3}}×\mathrm{EN}=\frac{\mathrm{2}}{\mathrm{3}}×\frac{\sqrt{\mathrm{7}}}{\mathrm{2}}=\frac{\sqrt{\mathrm{7}}}{\mathrm{3}} \\ $$$$\Rightarrow\mathrm{KN}=\frac{\mathrm{1}}{\mathrm{3}}×\mathrm{EN}=\frac{\mathrm{1}}{\mathrm{3}}×\frac{\sqrt{\mathrm{7}}}{\mathrm{2}}=\frac{\sqrt{\mathrm{7}}}{\mathrm{6}} \\ $$$$\left\{\right. \\ $$$$\mathrm{EK}^{\mathrm{2}} =\mathrm{OE}^{\mathrm{2}} +\mathrm{OK}^{\mathrm{2}} −\mathrm{2}×\mathrm{OE}×\mathrm{OK}×\mathrm{cos}\:\mathrm{60}° \\ $$$$\frac{\mathrm{7}}{\mathrm{9}}=\mathrm{1}+\mathrm{OK}^{\mathrm{2}} −\mathrm{2}×\mathrm{1}×\mathrm{OK}×\frac{\mathrm{1}}{\mathrm{2}} \\ $$$$\mathrm{OK}^{\mathrm{2}} −\mathrm{OK}+\frac{\mathrm{2}}{\mathrm{9}}=\mathrm{0} \\ $$$$\Rightarrow\mathrm{OK}=\frac{\mathrm{1}}{\mathrm{2}}\left(\mathrm{1}\pm\sqrt{\mathrm{1}−\frac{\mathrm{8}}{\mathrm{9}}}\right)=\frac{\mathrm{1}}{\mathrm{2}}\left(\mathrm{1}\pm\frac{\mathrm{1}}{\mathrm{3}}\right)=\frac{\mathrm{3}\pm\mathrm{1}}{\mathrm{6}}=\begin{cases}{\frac{\mathrm{1}}{\mathrm{3}}}\\{\frac{\mathrm{2}}{\mathrm{3}}}\end{cases} \\ $$$$\mathrm{only}\:\frac{\mathrm{1}}{\mathrm{3}}\:\mathrm{is}\:\mathrm{suitable}\:\mathrm{for}\:\mathrm{us}. \\ $$$$\Rightarrow\mathrm{OK}=\frac{\mathrm{1}}{\mathrm{3}} \\ $$$$\left.\right\} \\ $$$$\left(\mathrm{one}\:\mathrm{can}\:\mathrm{also}\:\mathrm{directly}\:\mathrm{use}\:\mathrm{formula}\:\mathrm{for}\right. \\ $$$$\left.\mathrm{angle}\:\mathrm{bisector}\:\mathrm{to}\:\mathrm{get}\:\mathrm{OK}\:\mathrm{instead}\:\mathrm{of}\:\left\{…\right\}\right) \\ $$$$\mathrm{KD}=\mathrm{OD}−\mathrm{OK}=\mathrm{1}−\frac{\mathrm{1}}{\mathrm{3}}=\frac{\mathrm{2}}{\mathrm{3}} \\ $$$$ \\ $$$$\Delta\mathrm{DKR}\sim\Delta\mathrm{EKO} \\ $$$$\frac{\mathrm{KR}}{\mathrm{KD}}=\frac{\mathrm{OK}}{\mathrm{EK}} \\ $$$$\Rightarrow\mathrm{KR}=\frac{\mathrm{1}}{\mathrm{3}}×\frac{\mathrm{3}}{\:\sqrt{\mathrm{7}}}×\frac{\mathrm{2}}{\mathrm{3}}=\frac{\mathrm{2}\sqrt{\mathrm{7}}}{\mathrm{21}} \\ $$$$\frac{\mathrm{RD}}{\mathrm{KD}}=\frac{\mathrm{OK}}{\mathrm{EO}} \\ $$$$\Rightarrow\mathrm{RD}=\frac{\mathrm{1}×\mathrm{3}}{\:\sqrt{\mathrm{7}}}×\frac{\mathrm{2}}{\mathrm{3}}=\frac{\mathrm{2}\sqrt{\mathrm{7}}}{\mathrm{7}} \\ $$$$\mathrm{RM}=\mathrm{DM}−\mathrm{RD}=\mathrm{EK}−\mathrm{RD}=\frac{\sqrt{\mathrm{7}}}{\mathrm{3}}−\frac{\mathrm{2}\sqrt{\mathrm{7}}}{\mathrm{7}}=\frac{\sqrt{\mathrm{7}}}{\mathrm{21}} \\ $$$$ \\ $$$$\mathrm{SR}=\mathrm{SK}+\mathrm{KR}=\mathrm{RM}+\mathrm{KR}=\frac{\sqrt{\mathrm{7}}}{\mathrm{21}}+\frac{\mathrm{2}\sqrt{\mathrm{7}}}{\mathrm{21}}=\frac{\sqrt{\mathrm{7}}}{\mathrm{7}} \\ $$$$=\mathrm{side}\:\mathrm{length}\:\mathrm{of}\:\mathrm{the}\:\mathrm{small}\:\mathrm{hexagon} \\ $$$$ \\ $$$$\frac{\mathrm{A}_{\mathrm{small}} }{\mathrm{A}_{\mathrm{big}} }=\left(\frac{\mathrm{side}\:\mathrm{length}\:\mathrm{small}}{\mathrm{side}\:\mathrm{length}\:\mathrm{big}}\right)^{\mathrm{2}} =\left(\frac{\frac{\sqrt{\mathrm{7}}}{\mathrm{7}}\mathrm{a}}{\mathrm{a}}\right)^{\mathrm{2}} =\frac{\mathrm{1}}{\mathrm{7}} \\ $$
Commented by ajfour last updated on 25/Oct/17

$${thank}\:{you}\:{sir},\:{but}\:{i}\:{shall}\:{also} \\ $$$${attempt}\:{now}.\:{Vector}\:{way}.. \\ $$$${perhaps}! \\ $$
Commented by mrW1 last updated on 25/Oct/17
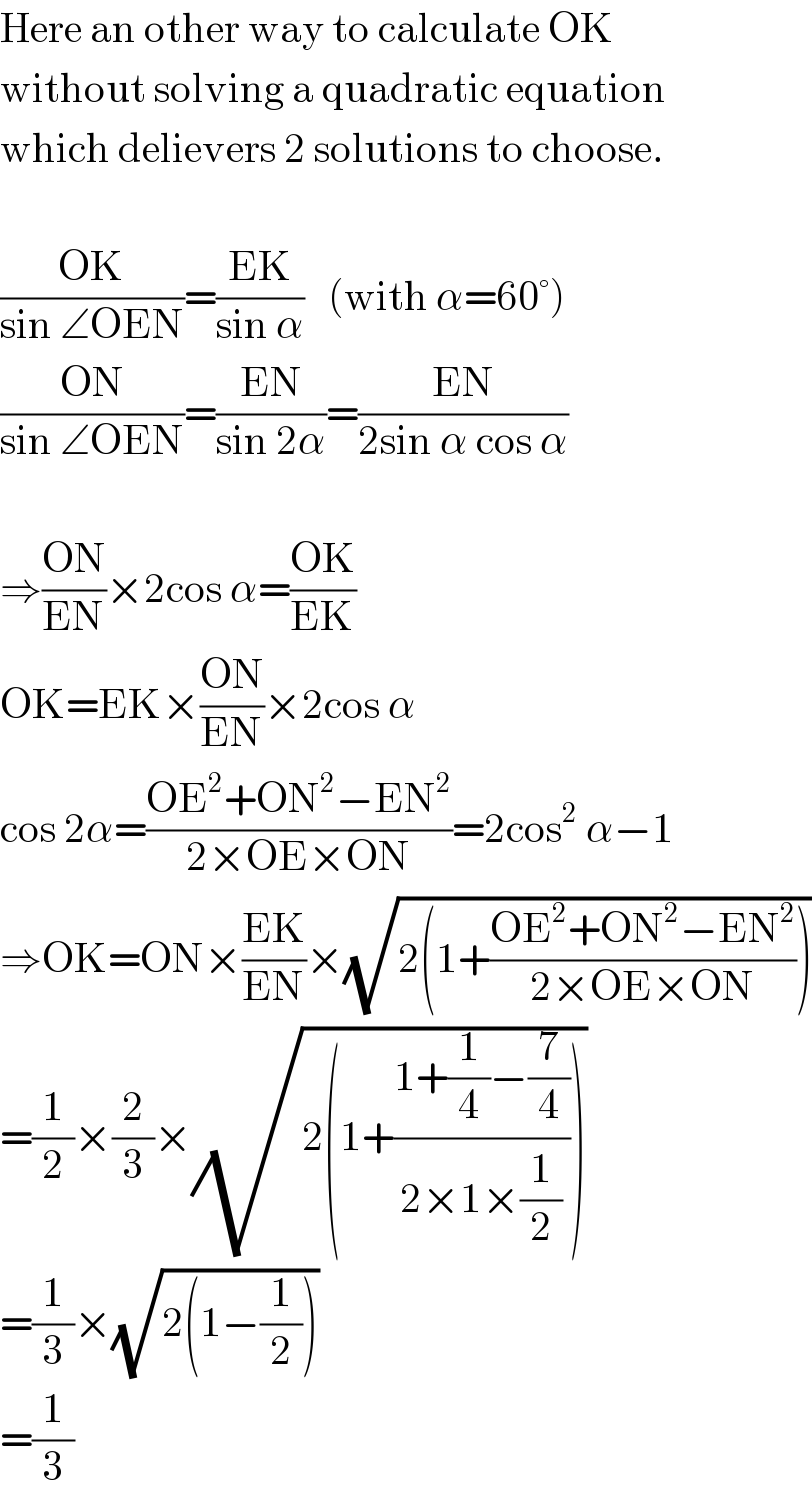
$$\mathrm{Here}\:\mathrm{an}\:\mathrm{other}\:\mathrm{way}\:\mathrm{to}\:\mathrm{calculate}\:\mathrm{OK} \\ $$$$\mathrm{without}\:\mathrm{solving}\:\mathrm{a}\:\mathrm{quadratic}\:\mathrm{equation} \\ $$$$\mathrm{which}\:\mathrm{delievers}\:\mathrm{2}\:\mathrm{solutions}\:\mathrm{to}\:\mathrm{choose}. \\ $$$$ \\ $$$$\frac{\mathrm{OK}}{\mathrm{sin}\:\angle\mathrm{OEN}}=\frac{\mathrm{EK}}{\mathrm{sin}\:\alpha}\:\:\:\left(\mathrm{with}\:\alpha=\mathrm{60}°\right) \\ $$$$\frac{\mathrm{ON}}{\mathrm{sin}\:\angle\mathrm{OEN}}=\frac{\mathrm{EN}}{\mathrm{sin}\:\mathrm{2}\alpha}=\frac{\mathrm{EN}}{\mathrm{2sin}\:\alpha\:\mathrm{cos}\:\alpha} \\ $$$$ \\ $$$$\Rightarrow\frac{\mathrm{ON}}{\mathrm{EN}}×\mathrm{2cos}\:\alpha=\frac{\mathrm{OK}}{\mathrm{EK}} \\ $$$$\mathrm{OK}=\mathrm{EK}×\frac{\mathrm{ON}}{\mathrm{EN}}×\mathrm{2cos}\:\alpha \\ $$$$\mathrm{cos}\:\mathrm{2}\alpha=\frac{\mathrm{OE}^{\mathrm{2}} +\mathrm{ON}^{\mathrm{2}} −\mathrm{EN}^{\mathrm{2}} }{\mathrm{2}×\mathrm{OE}×\mathrm{ON}}=\mathrm{2cos}^{\mathrm{2}} \:\alpha−\mathrm{1} \\ $$$$\Rightarrow\mathrm{OK}=\mathrm{ON}×\frac{\mathrm{EK}}{\mathrm{EN}}×\sqrt{\mathrm{2}\left(\mathrm{1}+\frac{\mathrm{OE}^{\mathrm{2}} +\mathrm{ON}^{\mathrm{2}} −\mathrm{EN}^{\mathrm{2}} }{\mathrm{2}×\mathrm{OE}×\mathrm{ON}}\right)} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}×\frac{\mathrm{2}}{\mathrm{3}}×\sqrt{\mathrm{2}\left(\mathrm{1}+\frac{\mathrm{1}+\frac{\mathrm{1}}{\mathrm{4}}−\frac{\mathrm{7}}{\mathrm{4}}}{\mathrm{2}×\mathrm{1}×\frac{\mathrm{1}}{\mathrm{2}}}\right)} \\ $$$$=\frac{\mathrm{1}}{\mathrm{3}}×\sqrt{\mathrm{2}\left(\mathrm{1}−\frac{\mathrm{1}}{\mathrm{2}}\right)} \\ $$$$=\frac{\mathrm{1}}{\mathrm{3}} \\ $$
Commented by ajfour last updated on 25/Oct/17
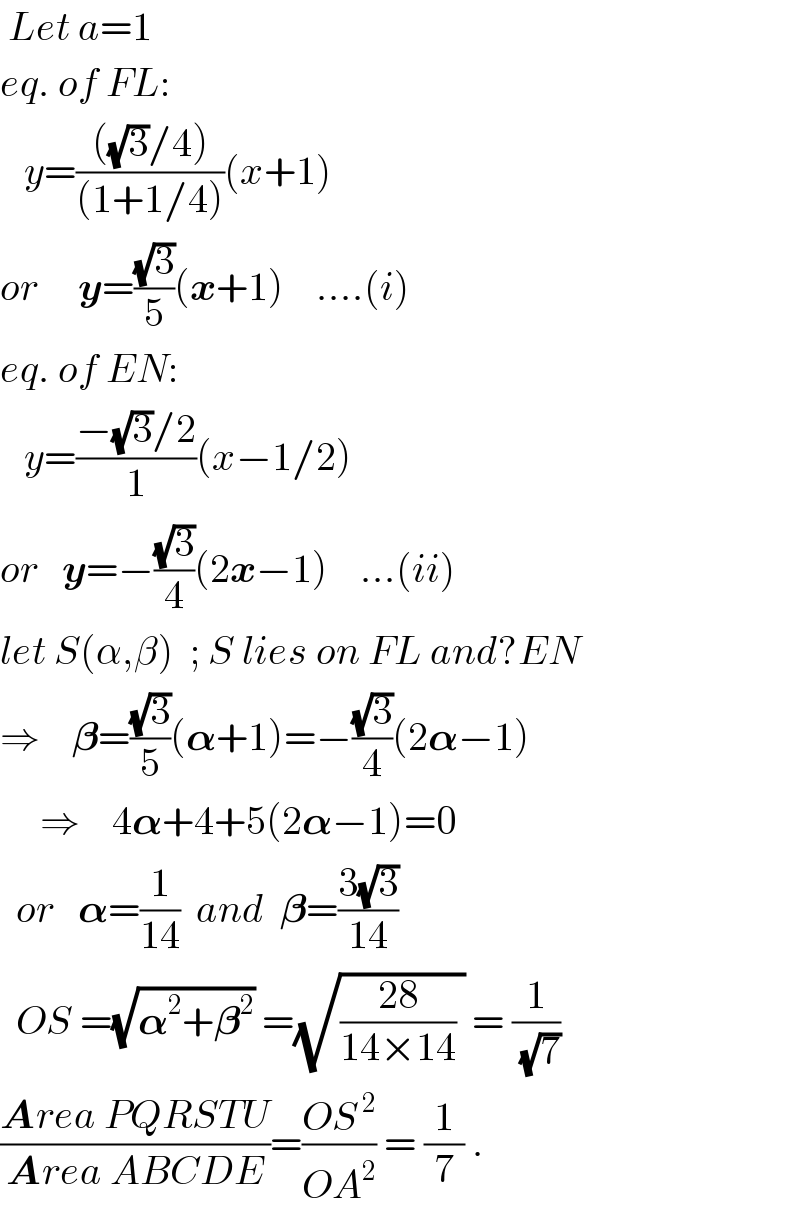
$$\:{Let}\:{a}=\mathrm{1} \\ $$$${eq}.\:{of}\:{FL}: \\ $$$$\:\:\:{y}=\frac{\left(\sqrt{\mathrm{3}}/\mathrm{4}\right)}{\left(\mathrm{1}+\mathrm{1}/\mathrm{4}\right)}\left({x}+\mathrm{1}\right) \\ $$$${or}\:\:\:\:\:\boldsymbol{{y}}=\frac{\sqrt{\mathrm{3}}}{\mathrm{5}}\left(\boldsymbol{{x}}+\mathrm{1}\right)\:\:\:\:….\left({i}\right) \\ $$$${eq}.\:{of}\:{EN}: \\ $$$$\:\:\:{y}=\frac{−\sqrt{\mathrm{3}}/\mathrm{2}}{\mathrm{1}}\left({x}−\mathrm{1}/\mathrm{2}\right) \\ $$$${or}\:\:\:\boldsymbol{{y}}=−\frac{\sqrt{\mathrm{3}}}{\mathrm{4}}\left(\mathrm{2}\boldsymbol{{x}}−\mathrm{1}\right)\:\:\:\:…\left({ii}\right) \\ $$$${let}\:{S}\left(\alpha,\beta\right)\:\:;\:{S}\:{lies}\:{on}\:{FL}\:{and}?{EN} \\ $$$$\Rightarrow\:\:\:\:\boldsymbol{\beta}=\frac{\sqrt{\mathrm{3}}}{\mathrm{5}}\left(\boldsymbol{\alpha}+\mathrm{1}\right)=−\frac{\sqrt{\mathrm{3}}}{\mathrm{4}}\left(\mathrm{2}\boldsymbol{\alpha}−\mathrm{1}\right) \\ $$$$\:\:\:\:\:\Rightarrow\:\:\:\:\mathrm{4}\boldsymbol{\alpha}+\mathrm{4}+\mathrm{5}\left(\mathrm{2}\boldsymbol{\alpha}−\mathrm{1}\right)=\mathrm{0} \\ $$$$\:\:{or}\:\:\:\boldsymbol{\alpha}=\frac{\mathrm{1}}{\mathrm{14}}\:\:{and}\:\:\boldsymbol{\beta}=\frac{\mathrm{3}\sqrt{\mathrm{3}}}{\mathrm{14}} \\ $$$$\:\:{OS}\:=\sqrt{\boldsymbol{\alpha}^{\mathrm{2}} +\boldsymbol{\beta}^{\mathrm{2}} }\:=\sqrt{\frac{\mathrm{28}}{\mathrm{14}×\mathrm{14}}\:}\:=\:\frac{\mathrm{1}}{\:\sqrt{\mathrm{7}}} \\ $$$$\frac{\boldsymbol{{A}}{rea}\:{PQRSTU}}{\boldsymbol{{A}}{rea}\:{ABCDE}}=\frac{{OS}^{\:\mathrm{2}} }{{OA}^{\mathrm{2}} }\:=\:\frac{\mathrm{1}}{\mathrm{7}}\:. \\ $$
Commented by mrW1 last updated on 25/Oct/17

$$\mathrm{very}\:\mathrm{nice}\:\mathrm{way}! \\ $$
Commented by math solver last updated on 25/Oct/17

$${sir}\:,\:{in}\:{eq}.\:{of}\:{FL}\:,\:{while}\:{writing}\:{slope} \\ $$$${i}\:{didn}'{t}\:{get}\:{the}\:{numerator}?? \\ $$$${i}\:{think}\:{numerator}\:{should}\:{be}\: \\ $$$${tan}\mathrm{30}×\frac{\mathrm{5}}{\mathrm{4}}=\mathrm{5}/\mathrm{4}\:×\sqrt{\:\mathrm{3}} \\ $$
Commented by ajfour last updated on 25/Oct/17

$${yes}. \\ $$
Commented by ajfour last updated on 25/Oct/17
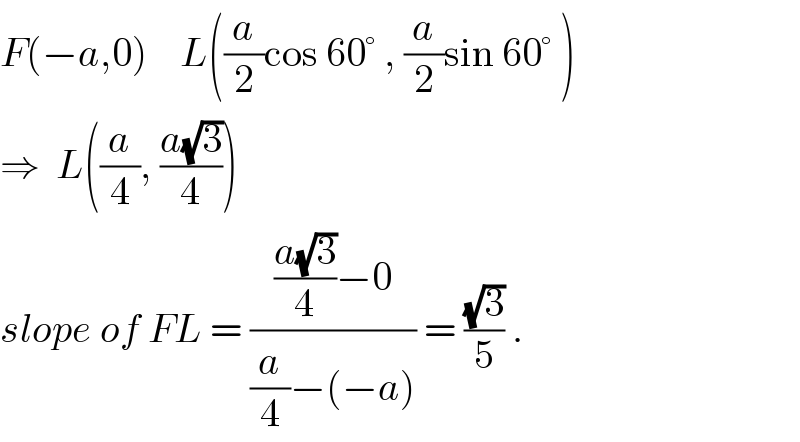
$${F}\left(−{a},\mathrm{0}\right)\:\:\:\:{L}\left(\frac{{a}}{\mathrm{2}}\mathrm{cos}\:\mathrm{60}°\:,\:\frac{{a}}{\mathrm{2}}\mathrm{sin}\:\mathrm{60}°\:\right) \\ $$$$\Rightarrow\:\:{L}\left(\frac{{a}}{\mathrm{4}},\:\frac{{a}\sqrt{\mathrm{3}}}{\mathrm{4}}\right) \\ $$$${slope}\:{of}\:{FL}\:=\:\frac{\frac{{a}\sqrt{\mathrm{3}}}{\mathrm{4}}−\mathrm{0}}{\frac{{a}}{\mathrm{4}}−\left(−{a}\right)}\:=\:\frac{\sqrt{\mathrm{3}}}{\mathrm{5}}\:. \\ $$
Commented by math solver last updated on 25/Oct/17
$${is}\:{L}\:{mid}\:{point}\:{of}\:{OD}? \\ $$
Commented by math solver last updated on 25/Oct/17
$${isn}'{t}\:{E}\left(−\mathrm{0}.\mathrm{5},\mathrm{1}\right)\:{and}\:{N}\left(\mathrm{0}.\mathrm{5},\mathrm{0}\right)\:{when}\: \\ $$$${a}=\mathrm{1}??? \\ $$
Commented by ajfour last updated on 26/Oct/17

$${E}\left(−\mathrm{0}.\mathrm{5},\:\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\right)\: \\ $$
Commented by math solver last updated on 26/Oct/17

$$\mathrm{ok}\:,\:\mathrm{i}\:\mathrm{got}\:\mathrm{it}\:\mathrm{now}\:. \\ $$$$\mathrm{thanks}! \\ $$
Answered by Tinkutara last updated on 26/Oct/17
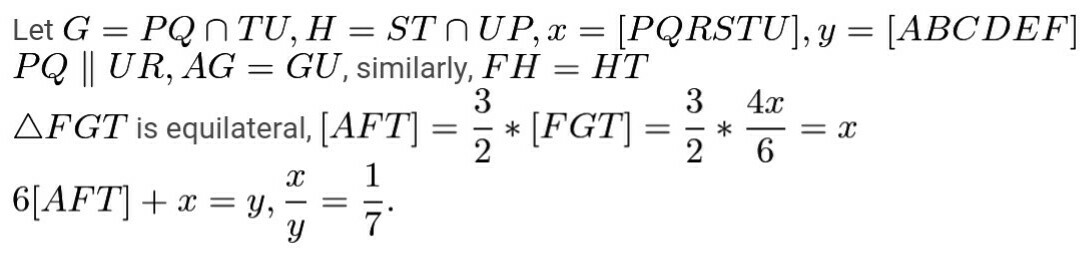
Commented by Tinkutara last updated on 26/Oct/17
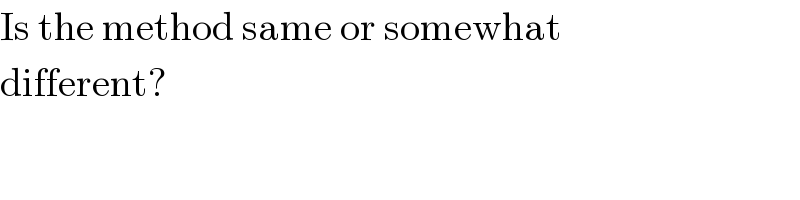
$$\mathrm{Is}\:\mathrm{the}\:\mathrm{method}\:\mathrm{same}\:\mathrm{or}\:\mathrm{somewhat} \\ $$$$\mathrm{different}? \\ $$
