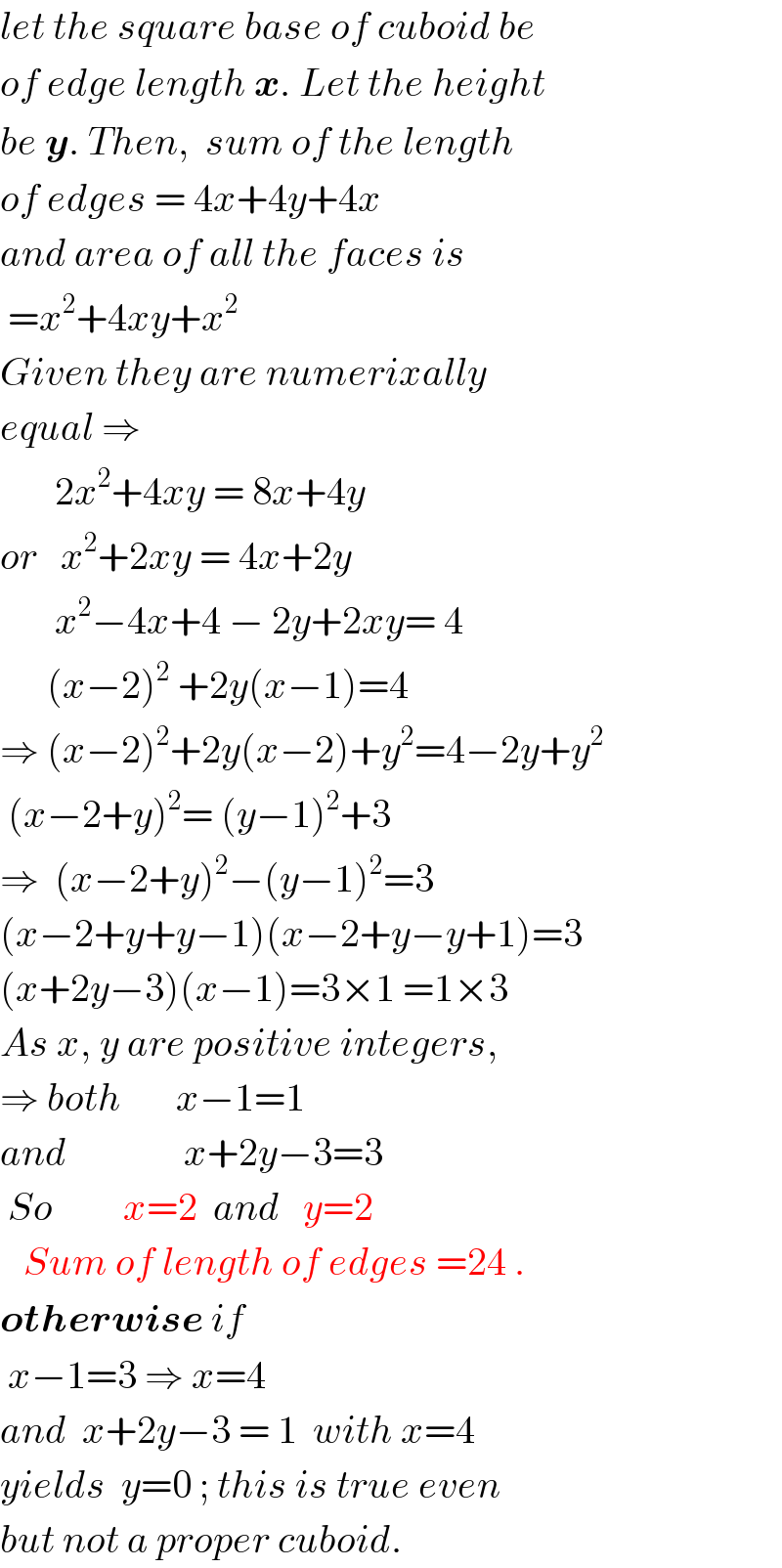Question-23537

Question Number 23537 by math solver last updated on 01/Nov/17

Commented by math solver last updated on 01/Nov/17

q . 7 ?

Commented by ajfour last updated on 01/Nov/17

y e s 24, a = 2, h = 2

i h a v e n e a r l y s o l v e d, b u t s o m e

p r o o f i s s t i l l w a n t i n g . .

Commented by math solver last updated on 01/Nov/17

sir it is not even in options!

Commented by math solver last updated on 01/Nov/17

the answer is 24 .

but i {don}^{'} t know how ?

Commented by math solver last updated on 01/Nov/17

did you get the required proof ?

Commented by Rasheed.Sindhi last updated on 02/Nov/17

{2 a}^{2} + 4 ah = 8 a + 4 h

a^{2} + 2 ah - 4 a = 2 h

a (a + 2 h - 4) = 2 h

\frac{2 h}{a} = a + 2 h - 4

\frac{2 h}{a} \in Z

a ∣ 2 or a ∣ h

a ∣ 2 \Rightarrow a = 1, 2

For a = 1

{2 a}^{2} + 4 ah = 8 a + 4 h

\Rightarrow 2 + 4 h = 8 + 4 h (i m p o s s i b l e)

\Rightarrow a = 2

{2 a}^{2} + 4 ah = 8 a + 4 h

8 + 8 h = 16 + 4 h \Rightarrow h = 2

One solution is a = h = 2

Hence sum of edges = 24

For a ∣ h

Let h = ka for some integer k .

{2 a}^{2} + 4 ah = 8 a + 4 h

\Rightarrow {2 a}^{2} + 4 a . ka = 8 a + 4 . ka

\Rightarrow {2 a}^{2} + {4 ka}^{2} = 8 a + 4 ka

\Rightarrow a + 2 ka = 4 + 2 k

\Rightarrow 2 k (a - 1) = 4 - a

\frac{4 - a}{2 (a - 1)} = k

k = 1 \Rightarrow 4 - a = 2 a - 2 \Rightarrow a = 2

Again a = h = 2 and sum of edges = 24

k = 2 \Rightarrow 4 - a = 4 (a - 1) \Rightarrow a = \frac{8}{5} \notin N

Continue

Commented by ajfour last updated on 01/Nov/17

y e s, j u s t n o w .

Commented by math solver last updated on 01/Nov/17

from here how you get a = h = 2

Answered by ajfour last updated on 01/Nov/17

l e t t h e s q u a r e b a s e o f c u b o i d b e

o f e d g e l e n g t h x . L e t t h e h e i g h t

b e y . T h e n, s u m o f t h e l e n g t h

o f e d g e s = 4 x + 4 y + 4 x

a n d a r e a o f a l l t h e f a c e s i s

= x^{2} + 4 x y + x^{2}

G i v e n t h e y a r e n u m e r i x a l l y

e q u a l \Rightarrow

2 x^{2} + 4 x y = 8 x + 4 y

o r x^{2} + 2 x y = 4 x + 2 y

x^{2} - 4 x + 4 - 2 y + 2 x y = 4

{(x - 2)}^{2} + 2 y (x - 1) = 4

\Rightarrow {(x - 2)}^{2} + 2 y (x - 2) + y^{2} = 4 - 2 y + y^{2}

{(x - 2 + y)}^{2} = {(y - 1)}^{2} + 3

\Rightarrow {(x - 2 + y)}^{2} - {(y - 1)}^{2} = 3

(x - 2 + y + y - 1) (x - 2 + y - y + 1) = 3

(x + 2 y - 3) (x - 1) = 3 \times 1 = 1 \times 3

A s x, y a r e p o s i t i v e i n t e g e r s,

\Rightarrow b o t h x - 1 = 1

a n d x + 2 y - 3 = 3

S o x = 2 a n d y = 2

S u m o f l e n g t h o f e d g e s = 24 .

o t h e r w i s e i f

x - 1 = 3 \Rightarrow x = 4

a n d x + 2 y - 3 = 1 w i t h x = 4

y i e l d s y = 0; t h i s i s t r u e e v e n

b u t n o t a p r o p e r c u b o i d .

Facebook Tweet Pin