Question Number 25975 by mariahameed97@gmail.com last updated on 17/Dec/17

Answered by Rasheed.Sindhi last updated on 17/Dec/17
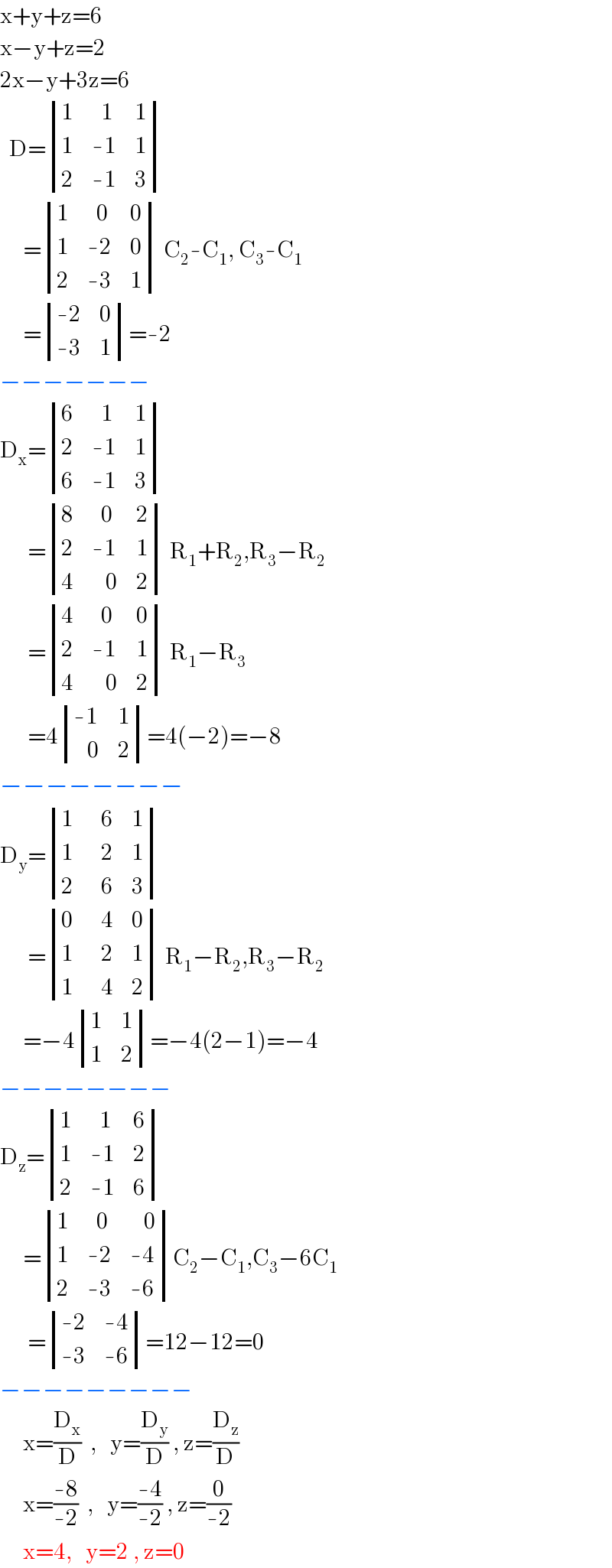
$$\mathrm{x}+\mathrm{y}+\mathrm{z}=\mathrm{6} \\ $$$$\mathrm{x}−\mathrm{y}+\mathrm{z}=\mathrm{2} \\ $$$$\mathrm{2x}−\mathrm{y}+\mathrm{3z}=\mathrm{6} \\ $$$$\:\:\mathrm{D}=\begin{vmatrix}{\mathrm{1}}&{\:\:\mathrm{1}}&{\mathrm{1}}\\{\mathrm{1}}&{-\mathrm{1}}&{\mathrm{1}}\\{\mathrm{2}}&{-\mathrm{1}}&{\mathrm{3}}\end{vmatrix} \\ $$$$\:\:\:\:\:=\begin{vmatrix}{\mathrm{1}}&{\:\:\mathrm{0}}&{\mathrm{0}}\\{\mathrm{1}}&{-\mathrm{2}}&{\mathrm{0}}\\{\mathrm{2}}&{-\mathrm{3}}&{\mathrm{1}}\end{vmatrix}\:\mathrm{C}_{\mathrm{2}} -\mathrm{C}_{\mathrm{1}} ,\:\mathrm{C}_{\mathrm{3}} -\mathrm{C}_{\mathrm{1}} \\ $$$$\:\:\:\:\:=\begin{vmatrix}{-\mathrm{2}}&{\mathrm{0}}\\{-\mathrm{3}}&{\mathrm{1}}\end{vmatrix}=-\mathrm{2} \\ $$$$−−−−−−− \\ $$$$\mathrm{D}_{\mathrm{x}} =\begin{vmatrix}{\mathrm{6}}&{\:\:\mathrm{1}}&{\mathrm{1}}\\{\mathrm{2}}&{-\mathrm{1}}&{\mathrm{1}}\\{\mathrm{6}}&{-\mathrm{1}}&{\mathrm{3}}\end{vmatrix} \\ $$$$\:\:\:\:\:\:=\begin{vmatrix}{\mathrm{8}}&{\:\:\mathrm{0}}&{\mathrm{2}}\\{\mathrm{2}}&{-\mathrm{1}}&{\mathrm{1}}\\{\mathrm{4}}&{\:\:\:\mathrm{0}}&{\mathrm{2}}\end{vmatrix}\:\mathrm{R}_{\mathrm{1}} +\mathrm{R}_{\mathrm{2}} ,\mathrm{R}_{\mathrm{3}} −\mathrm{R}_{\mathrm{2}} \\ $$$$\:\:\:\:\:\:=\begin{vmatrix}{\mathrm{4}}&{\:\:\mathrm{0}}&{\mathrm{0}}\\{\mathrm{2}}&{-\mathrm{1}}&{\mathrm{1}}\\{\mathrm{4}}&{\:\:\:\mathrm{0}}&{\mathrm{2}}\end{vmatrix}\:\mathrm{R}_{\mathrm{1}} −\mathrm{R}_{\mathrm{3}} \\ $$$$\:\:\:\:\:\:=\mathrm{4}\begin{vmatrix}{-\mathrm{1}}&{\mathrm{1}}\\{\:\:\:\mathrm{0}}&{\mathrm{2}}\end{vmatrix}=\mathrm{4}\left(−\mathrm{2}\right)=−\mathrm{8} \\ $$$$−−−−−−−− \\ $$$$\mathrm{D}_{\mathrm{y}} =\begin{vmatrix}{\mathrm{1}}&{\:\:\mathrm{6}}&{\mathrm{1}}\\{\mathrm{1}}&{\:\:\mathrm{2}}&{\mathrm{1}}\\{\mathrm{2}}&{\:\:\mathrm{6}}&{\mathrm{3}}\end{vmatrix} \\ $$$$\:\:\:\:\:\:=\begin{vmatrix}{\mathrm{0}}&{\:\:\mathrm{4}}&{\mathrm{0}}\\{\mathrm{1}}&{\:\:\mathrm{2}}&{\mathrm{1}}\\{\mathrm{1}}&{\:\:\mathrm{4}}&{\mathrm{2}}\end{vmatrix}\:\mathrm{R}_{\mathrm{1}} −\mathrm{R}_{\mathrm{2}} ,\mathrm{R}_{\mathrm{3}} −\mathrm{R}_{\mathrm{2}} \\ $$$$\:\:\:\:\:=−\mathrm{4}\begin{vmatrix}{\mathrm{1}}&{\mathrm{1}}\\{\mathrm{1}}&{\mathrm{2}}\end{vmatrix}=−\mathrm{4}\left(\mathrm{2}−\mathrm{1}\right)=−\mathrm{4} \\ $$$$−−−−−−−− \\ $$$$\mathrm{D}_{\mathrm{z}} =\begin{vmatrix}{\mathrm{1}}&{\:\:\mathrm{1}}&{\mathrm{6}}\\{\mathrm{1}}&{-\mathrm{1}}&{\mathrm{2}}\\{\mathrm{2}}&{-\mathrm{1}}&{\mathrm{6}}\end{vmatrix} \\ $$$$\:\:\:\:\:=\begin{vmatrix}{\mathrm{1}}&{\:\:\mathrm{0}}&{\:\:\:\mathrm{0}}\\{\mathrm{1}}&{-\mathrm{2}}&{-\mathrm{4}}\\{\mathrm{2}}&{-\mathrm{3}}&{-\mathrm{6}}\end{vmatrix}\mathrm{C}_{\mathrm{2}} −\mathrm{C}_{\mathrm{1}} ,\mathrm{C}_{\mathrm{3}} −\mathrm{6C}_{\mathrm{1}} \\ $$$$\:\:\:\:\:\:=\begin{vmatrix}{-\mathrm{2}}&{-\mathrm{4}}\\{-\mathrm{3}}&{-\mathrm{6}}\end{vmatrix}=\mathrm{12}−\mathrm{12}=\mathrm{0} \\ $$$$−−−−−−−−− \\ $$$$\:\:\:\:\:\mathrm{x}=\frac{\mathrm{D}_{\mathrm{x}} }{\mathrm{D}}\:\:,\:\:\:\mathrm{y}=\frac{\mathrm{D}_{\mathrm{y}} }{\mathrm{D}}\:,\:\mathrm{z}=\frac{\mathrm{D}_{\mathrm{z}} }{\mathrm{D}} \\ $$$$\:\:\:\:\:\mathrm{x}=\frac{-\mathrm{8}}{-\mathrm{2}}\:\:,\:\:\:\mathrm{y}=\frac{-\mathrm{4}}{-\mathrm{2}}\:,\:\mathrm{z}=\frac{\mathrm{0}}{-\mathrm{2}} \\ $$$$\:\:\:\:\:\mathrm{x}=\mathrm{4},\:\:\:\mathrm{y}=\mathrm{2}\:,\:\mathrm{z}=\mathrm{0} \\ $$
Commented by mariahameed97@gmail.com last updated on 17/Dec/17

$$\mathrm{thnxx} \\ $$
