Question-29522

Question Number 29522 by 803jaideep@gmail.com last updated on 09/Feb/18

Commented by 803jaideep@gmail.com last updated on 09/Feb/18

40 th ques sry

Commented by Tinkutara last updated on 09/Feb/18

120?

Commented by 803jaideep@gmail.com last updated on 09/Feb/18

yes yes but how

Commented by Tinkutara last updated on 09/Feb/18

See Q 20739

Commented by math solver last updated on 10/Feb/18

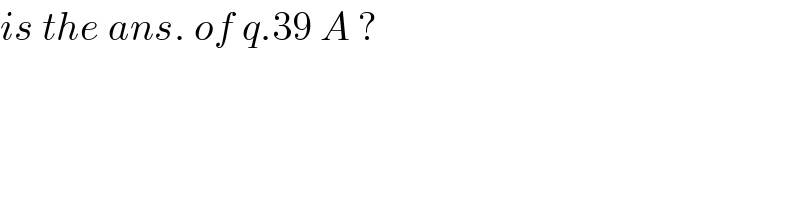
i s t h e a n s . o f q . 39 A ?

Commented by prof Abdo imad last updated on 11/Feb/18

38) i f z = c o s α + i s i n α l e t f i n d a r g (z^{2} + z^{-})

t h e m o i v r e f o r m u l a g i v e z^{2} = c o s (2 α) + i s i n (2 α)

a m d z^{-} = c o s α - i s i n α \Rightarrow

z^{2} + z^{-} = c o s α + c o s (2 α) + i (s i n (2 α) - s i n α)

= 2 c o s (\frac{3 α}{2}) c o s (\frac{α}{2}) + i (s i n (2 α) - s i n α)

s i n p ? - s i n q = c o s (\frac{π}{2} - p) + c o s (\frac{π}{2} + q)

= 2 c o s (\frac{π - p + q}{2}) c o s \frac{p + q}{2} = 2 s i n (\frac{p - q}{2}) c o s (\frac{p + q}{2})

s i n (2 α) - s i n α = 2 s i n (\frac{α}{2}) c o s (\frac{3 α}{2}) \Rightarrow

z^{2} + z^{-} = 2 c o s (\frac{3 α}{2}) (c o s (\frac{α}{2}) + i s i n (\frac{α}{2}) \Rightarrow

a r g (z^{2} + z^{-}) = \frac{α}{2} .

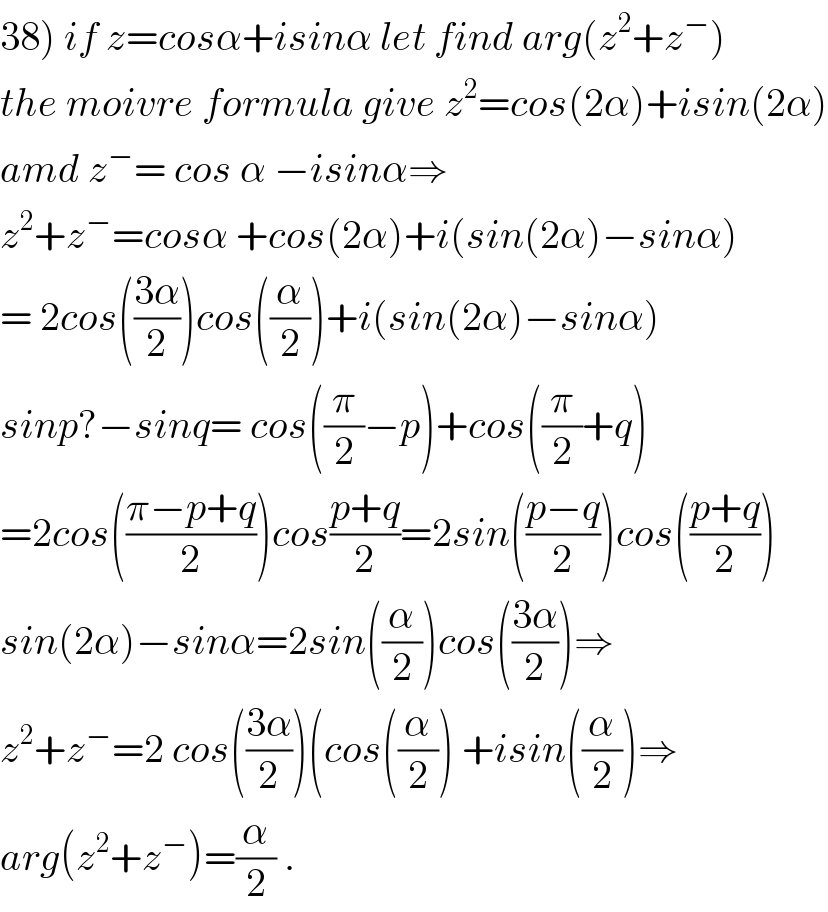
Commented by math solver last updated on 11/Feb/18

wow, sir .

Answered by 803jaideep@gmail.com last updated on 09/Feb/18

10 th