Question-31584

Question Number 31584 by Tinkutara last updated on 10/Mar/18

Answered by mrW2 last updated on 10/Mar/18

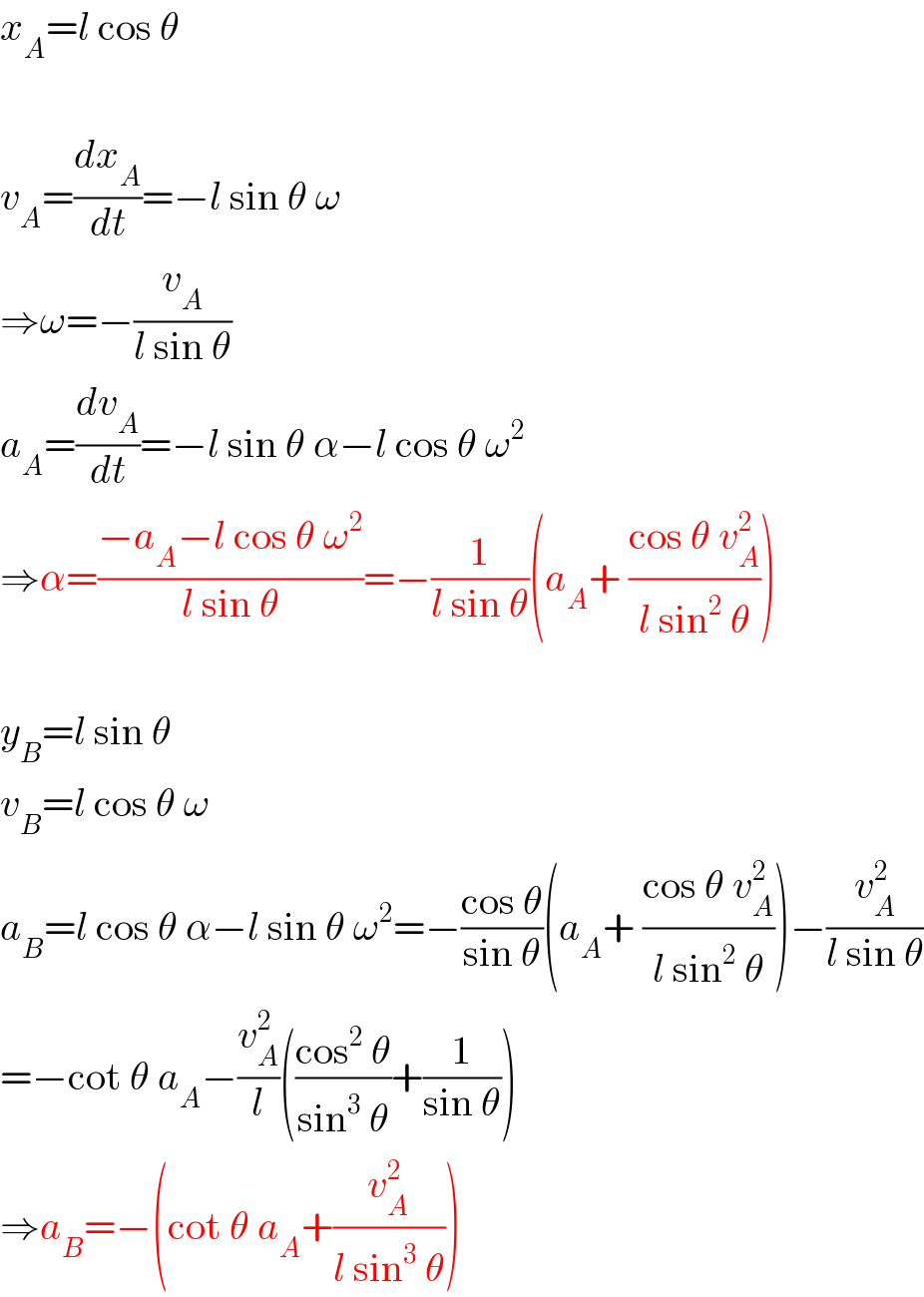
x_A =l cos θ v_A =(dx_A /dt)=−l sin θ ω ⇒ω=−(v_A /(l sin θ)) a_A =(dv_A /dt)=−l sin θ α−l cos θ ω^2 ⇒α=((−a_A −l cos θ ω^2 )/(l sin θ))=−(1/(l sin θ))(a_A + ((cos θ v_A ^2 )/(l sin^2 θ))) y_B =l sin θ v_B =l cos θ ω a_B =l cos θ α−l sin θ ω^2 =−((cos θ)/(sin θ))(a_A + ((cos θ v_A ^2 )/(l sin^2 θ)))−(v_A ^2 /(l sin θ)) =−cot θ a_A −(v_A ^2 /l)(((cos^2 θ)/(sin^3 θ))+(1/(sin θ))) ⇒a_B =−(cot θ a_A +(v_A ^2 /(l sin^3 θ)))

$${x}_{{A}} ={l}\:\mathrm{cos}\:\theta \\ $$$$ \\ $$$${v}_{{A}} =\frac{{dx}_{{A}} }{{dt}}=−{l}\:\mathrm{sin}\:\theta\:\omega \\ $$$$\Rightarrow\omega=−\frac{{v}_{{A}} }{{l}\:\mathrm{sin}\:\theta} \\ $$$${a}_{{A}} =\frac{{dv}_{{A}} }{{dt}}=−{l}\:\mathrm{sin}\:\theta\:\alpha−{l}\:\mathrm{cos}\:\theta\:\omega^{\mathrm{2}} \\ $$$$\Rightarrow\alpha=\frac{−{a}_{{A}} −{l}\:\mathrm{cos}\:\theta\:\omega^{\mathrm{2}} }{{l}\:\mathrm{sin}\:\theta}=−\frac{\mathrm{1}}{{l}\:\mathrm{sin}\:\theta}\left({a}_{{A}} +\:\frac{\mathrm{cos}\:\theta\:{v}_{{A}} ^{\mathrm{2}} }{{l}\:\mathrm{sin}^{\mathrm{2}} \:\theta}\right) \\ $$$$ \\ $$$${y}_{{B}} ={l}\:\mathrm{sin}\:\theta \\ $$$${v}_{{B}} ={l}\:\mathrm{cos}\:\theta\:\omega \\ $$$${a}_{{B}} ={l}\:\mathrm{cos}\:\theta\:\alpha−{l}\:\mathrm{sin}\:\theta\:\omega^{\mathrm{2}} =−\frac{\mathrm{cos}\:\theta}{\mathrm{sin}\:\theta}\left({a}_{{A}} +\:\frac{\mathrm{cos}\:\theta\:{v}_{{A}} ^{\mathrm{2}} }{{l}\:\mathrm{sin}^{\mathrm{2}} \:\theta}\right)−\frac{{v}_{{A}} ^{\mathrm{2}} }{{l}\:\mathrm{sin}\:\theta} \\ $$$$=−\mathrm{cot}\:\theta\:{a}_{{A}} −\frac{{v}_{{A}} ^{\mathrm{2}} }{{l}}\left(\frac{\mathrm{cos}^{\mathrm{2}} \:\theta}{\mathrm{sin}^{\mathrm{3}} \:\theta}+\frac{\mathrm{1}}{\mathrm{sin}\:\theta}\right) \\ $$$$\Rightarrow{a}_{{B}} =−\left(\mathrm{cot}\:\theta\:{a}_{{A}} +\frac{{v}_{{A}} ^{\mathrm{2}} }{{l}\:\mathrm{sin}^{\mathrm{3}} \:\theta}\right) \\ $$

Commented by Tinkutara last updated on 10/Mar/18

Commented by Tinkutara last updated on 10/Mar/18

Do I need to further do vector sum of these? And how to find ω and Iα?

$${Do}\:{I}\:{need}\:{to}\:{further}\:{do}\:{vector}\:{sum} \\ $$$${of}\:{these}?\:{And}\:{how}\:{to}\:{find}\:\omega\:{and}\:{I}\alpha? \\ $$

Commented by Tinkutara last updated on 11/Mar/18

Please see it's my book solution but I can't understand that.

Commented by mrW2 last updated on 11/Mar/18

$${i}\:{can}\:{not}\:{help}\:{you}\:{sir}.\:{i}\:{personally} \\ $$$${won}'{t}\:{do}\:{in}\:{that}\:{way}\:{as}\:{the}\:{book}. \\ $$

Commented by Tinkutara last updated on 11/Mar/18

I am not asking you sir to solve that way. I am just asking that the book is correct to say that the final acceleration is the vector sum of that 3 so we need to find that? I highly appreciate your method!

Facebook Tweet Pin

Leave a Reply Cancel reply