Question-33214

Question Number 33214 by Tinkutara last updated on 13/Apr/18

Commented by Tinkutara last updated on 13/Apr/18

Commented by Tinkutara last updated on 15/Apr/18

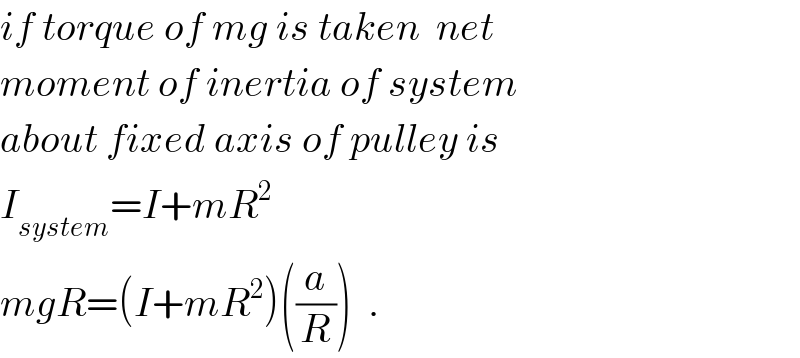
But if we consider the torque of mg instead of ma it still gives approximately the same answer. Which one is conceptually correct?

Commented by ajfour last updated on 14/Apr/18

Commented by ajfour last updated on 14/Apr/18

m g - T = m a

T R = I (\frac{a}{R})

\Rightarrow m g = a [m + \frac{I}{R^{2}}] \dots (1)

s = \frac{1}{2} {a t}^{2} \Rightarrow a = \frac{2 s}{t^{2}}

u s i n g i n (1)

m g = \frac{2 s}{t^{2}} (m + \frac{I}{R^{2}})

o r I = (\frac{{m g t}^{2}}{2 s} - m) R^{2}

= (\frac{0.5 \times 10 \times 16}{2 \times 1.5} - 0.5) {(0.15)}^{2}

= (\frac{80}{3} - 0.5) (0.0225) k g . m^{2}

= (\frac{80}{3} - \frac{1}{2}) (\frac{9}{400}) k g . m^{2}

= \frac{157}{6} \times \frac{9}{400} = \frac{157 \times 3}{800} = \frac{4.71}{8}

= 0.59 k g . m^{2} .

Commented by ajfour last updated on 15/Apr/18

i f t o r q u e o f m g i s t a k e n n e t

m o m e n t o f i n e r t i a o f s y s t e m

a b o u t f i x e d a x i s o f p u l l e y i s

I_{s y s t e m} = I + {m R}^{2}

m g R = (I + {m R}^{2}) (\frac{a}{R}) .

Commented by Tinkutara last updated on 15/Apr/18

Both are same in value approximately. Which is correct then?

Commented by ajfour last updated on 15/Apr/18

h a v e s a m e v a l u e e x a c t l y . h o w a p p r o x i m a t e l y ?

Commented by Tinkutara last updated on 15/Apr/18

Thank you very much Sir! I got the answer. ��