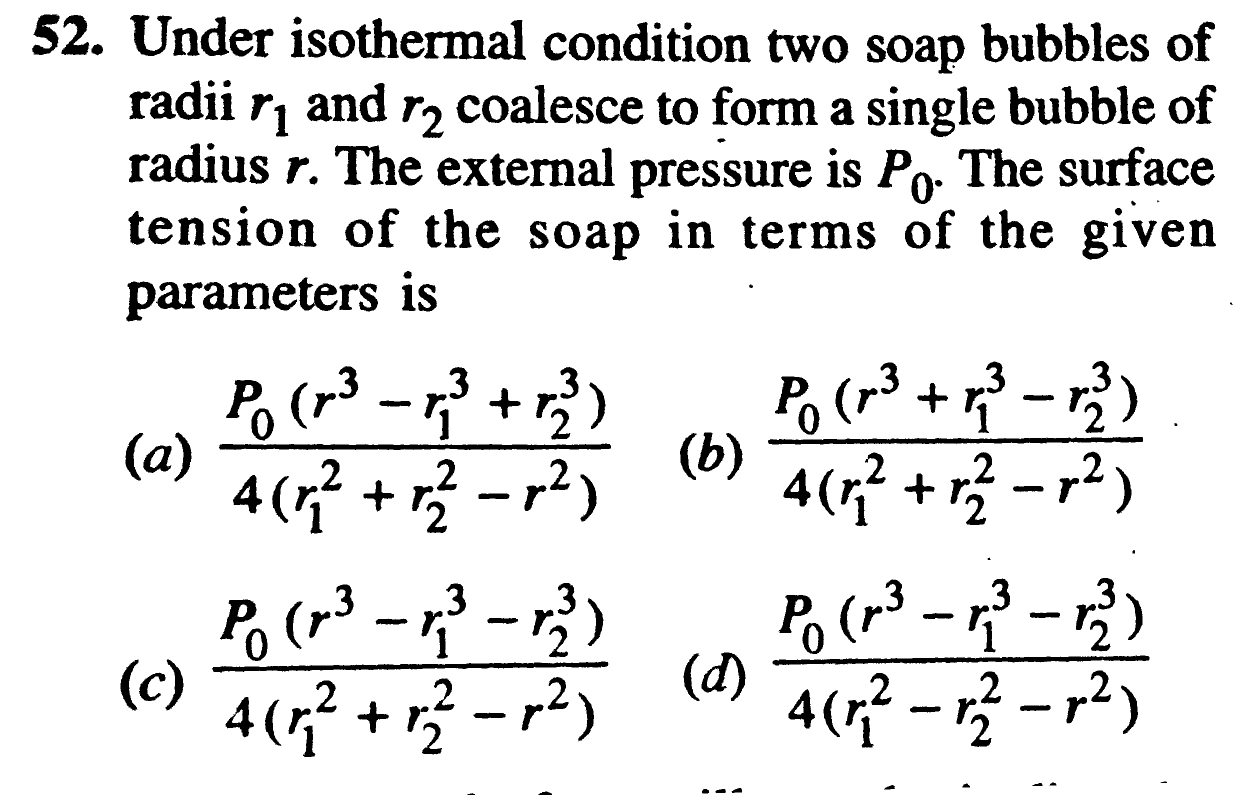
Question Number 34762 by Tinkutara last updated on 10/May/18
Answered by ajfour last updated on 10/May/18

$${n}_{\mathrm{1}} +{n}_{\mathrm{2}} ={n} \\ $$$$\left({P}_{\mathrm{0}} +\frac{\mathrm{4}{S}}{{r}_{\mathrm{1}} }\right){r}_{\mathrm{1}} ^{\mathrm{3}} +\left({P}_{\mathrm{0}} +\frac{\mathrm{4}{S}}{{r}_{\mathrm{2}} }\right){r}_{\mathrm{2}} ^{\mathrm{3}} =\left({P}_{\mathrm{0}} +\frac{\mathrm{4}{S}}{{r}}\right){r}^{\mathrm{3}} \\ $$$$\Rightarrow\:{S}=\frac{{P}_{\mathrm{0}} \left({r}^{\mathrm{3}} −{r}_{\mathrm{1}} ^{\mathrm{3}} −{r}_{\mathrm{2}} ^{\mathrm{3}} \right)}{\mathrm{4}\left({r}_{\mathrm{1}} ^{\mathrm{2}} +{r}_{\mathrm{2}} ^{\mathrm{2}} −{r}^{\mathrm{2}} \right)}\:\:.\:\:\:\:\left({c}\right)\:. \\ $$
Commented by Tinkutara last updated on 11/May/18

$${But}\:{here}\:{if}\:{we}\:{see}\:{numerator},\:{then} \\ $$$${it}\:{should}\:{be}\:\mathrm{0}?\:{Because}\:{applying} \\ $$$${condition}\:{r}^{\mathrm{3}} ={r}_{\mathrm{1}} ^{\mathrm{3}} +{r}_{\mathrm{2}} ^{\mathrm{3}} .\:{Why}\:{this}\:{is}\:{wrong}? \\ $$
Commented by ajfour last updated on 11/May/18

$${we}\:{cannot}\:{balance}\:{volume}\:{of} \\ $$$${air}\:{in}\:{bubbles},\:{we}\:{can}\:{only} \\ $$$${conserve}\:{the}\:{no}.\:{of}\:{moles}\:{of}\:{air}. \\ $$$${So}\:\:\:\:{r}^{\mathrm{3}} \:{may}\:{not}\:{be}\:{equal}\:{to}\:{r}_{\mathrm{1}} ^{\mathrm{3}} +{r}_{\mathrm{2}} ^{\mathrm{3}} \:. \\ $$
Commented by Tinkutara last updated on 11/May/18

$${But}\:{we}\:{conserve}\:{volume}\:{in}\:{drops}\:{why} \\ $$$${then}? \\ $$
Commented by ajfour last updated on 11/May/18

$${water}\:\:{is}\:{nearly}\:{incompressible}, \\ $$$${air}\:{volume}\:{changes}\:{if}\:{pressure} \\ $$$${changes}. \\ $$
Commented by Tinkutara last updated on 11/May/18
Thank you very much Sir! I got the answer. ��������
