Question Number 35153 by Victor31926 last updated on 16/May/18
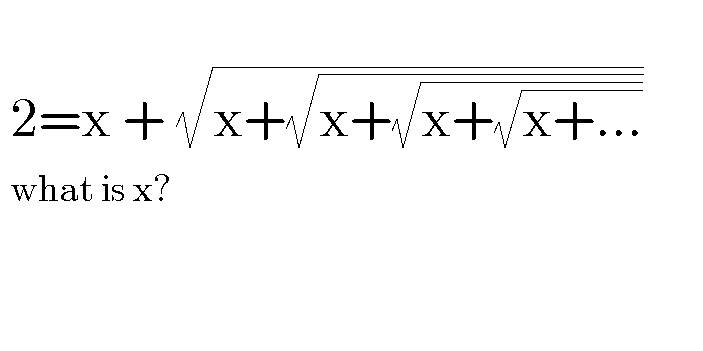
Answered by Rasheed.Sindhi last updated on 16/May/18
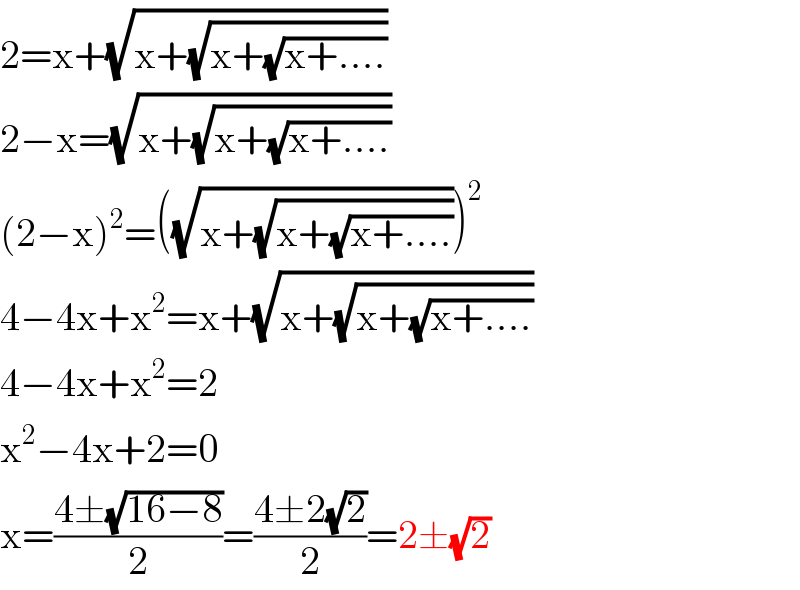
$$\mathrm{2}=\mathrm{x}+\sqrt{\mathrm{x}+\sqrt{\mathrm{x}+\sqrt{\mathrm{x}+….}}} \\ $$$$\mathrm{2}−\mathrm{x}=\sqrt{\mathrm{x}+\sqrt{\mathrm{x}+\sqrt{\mathrm{x}+….}}} \\ $$$$\left(\mathrm{2}−\mathrm{x}\right)^{\mathrm{2}} =\left(\sqrt{\mathrm{x}+\sqrt{\mathrm{x}+\sqrt{\mathrm{x}+….}}}\right)^{\mathrm{2}} \\ $$$$\mathrm{4}−\mathrm{4x}+\mathrm{x}^{\mathrm{2}} =\mathrm{x}+\sqrt{\mathrm{x}+\sqrt{\mathrm{x}+\sqrt{\mathrm{x}+….}}} \\ $$$$\mathrm{4}−\mathrm{4x}+\mathrm{x}^{\mathrm{2}} =\mathrm{2} \\ $$$$\mathrm{x}^{\mathrm{2}} −\mathrm{4x}+\mathrm{2}=\mathrm{0} \\ $$$$\mathrm{x}=\frac{\mathrm{4}\pm\sqrt{\mathrm{16}−\mathrm{8}}}{\mathrm{2}}=\frac{\mathrm{4}\pm\mathrm{2}\sqrt{\mathrm{2}}}{\mathrm{2}}=\mathrm{2}\pm\sqrt{\mathrm{2}} \\ $$
