Question Number 35551 by tanmay.chaudhury50@gmail.com last updated on 20/May/18
Commented by abdo mathsup 649 cc last updated on 21/May/18
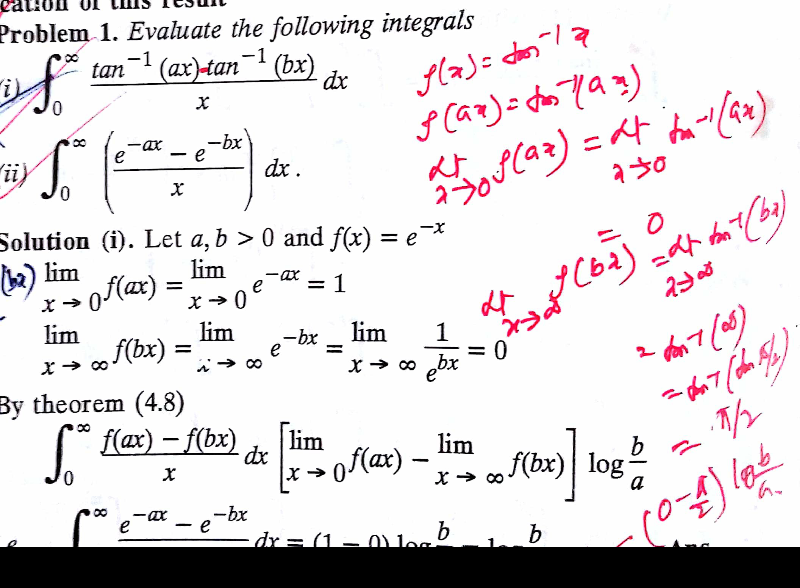
![let find the vslue of ∫_0 ^∞ ((e^(−ax) −e^(−bx) )/x)dx with a>0,b>0 let consider f(t) =∫_0 ^∞ ((e^(−ax) −e^(−bx) )/x)e^(−tx) d3 witht>0 after verifying the condition of derivsbility we have f^′ (t) = −∫_0 ^∞ e^(−tx) { e^(−ax) −e^(−bx) }dx =∫_0 ^∞ {e^(−(b+t)x) −e^(−(a+t)x) }dx =[((−1)/(b+t)) e^(−(b+t)x) + (1/(a+t)) e^(−(a+t)x) ]_0 ^(+∞) = (1/(b+t)) −(1/(a+t)) ⇒ f(t) = ln∣((b+t)/(a+t))∣ +c but ∃m>0 / ∣f(t)∣ ≤ m ∫_0 ^∞ e^(−tx) dx =(m/t) →0(t→+∞) c =lim_(t→+∞) { f(t) −ln∣((b+t)/(a+t))∣} =0⇒ f(t) =ln (((b+t)/(a+t))) and ∫_0 ^∞ ((e^(−ax) −e^(−bx) )/x)dx =f(0) =ln((b/a)) .](https://www.tinkutara.com/question/Q35663.png)
$${let}\:{find}\:{the}\:{vslue}\:{of}\:\int_{\mathrm{0}} ^{\infty} \:\:\:\frac{{e}^{−{ax}} \:−{e}^{−{bx}} }{{x}}{dx}\:{with} \\ $$$${a}>\mathrm{0},{b}>\mathrm{0}\:{let}\:{consider}\:\:{f}\left({t}\right)\:=\int_{\mathrm{0}} ^{\infty} \:\frac{{e}^{−{ax}} \:−{e}^{−{bx}} }{{x}}{e}^{−{tx}} {d}\mathrm{3} \\ $$$${witht}>\mathrm{0}\:{after}\:{verifying}\:{the}\:{condition}\:{of} \\ $$$${derivsbility}\:{we}\:{have}\: \\ $$$${f}^{'} \left({t}\right)\:=\:−\int_{\mathrm{0}} ^{\infty} \:\:{e}^{−{tx}} \left\{\:{e}^{−{ax}} \:−{e}^{−{bx}} \right\}{dx} \\ $$$$=\int_{\mathrm{0}} ^{\infty} \:\:\left\{{e}^{−\left({b}+{t}\right){x}} \:\:−{e}^{−\left({a}+{t}\right){x}} \right\}{dx}\: \\ $$$$=\left[\frac{−\mathrm{1}}{{b}+{t}}\:{e}^{−\left({b}+{t}\right){x}} \:\:+\:\frac{\mathrm{1}}{{a}+{t}}\:{e}^{−\left({a}+{t}\right){x}} \right]_{\mathrm{0}} ^{+\infty} \\ $$$$=\:\frac{\mathrm{1}}{{b}+{t}}\:−\frac{\mathrm{1}}{{a}+{t}}\:\:\Rightarrow\:{f}\left({t}\right)\:=\:{ln}\mid\frac{{b}+{t}}{{a}+{t}}\mid\:+{c}\:\:{but}\:\exists{m}>\mathrm{0}\:/ \\ $$$$\mid{f}\left({t}\right)\mid\:\leqslant\:{m}\:\int_{\mathrm{0}} ^{\infty} \:{e}^{−{tx}} {dx}\:=\frac{{m}}{{t}}\:\rightarrow\mathrm{0}\left({t}\rightarrow+\infty\right)\: \\ $$$${c}\:={lim}_{{t}\rightarrow+\infty} \left\{\:{f}\left({t}\right)\:−{ln}\mid\frac{{b}+{t}}{{a}+{t}}\mid\right\}\:=\mathrm{0}\Rightarrow \\ $$$${f}\left({t}\right)\:={ln}\:\left(\frac{{b}+{t}}{{a}+{t}}\right)\:\:{and}\:\int_{\mathrm{0}} ^{\infty} \:\:\:\:\frac{{e}^{−{ax}} \:−{e}^{−{bx}} }{{x}}{dx} \\ $$$$={f}\left(\mathrm{0}\right)\:={ln}\left(\frac{{b}}{{a}}\right)\:. \\ $$
